Histogram (Non-Uniform Widths)
These lessons cover histograms with non-uniform class widths, how to draw and interpret such histograms.
Related Pages
Histograms
Histograms - Illustrative Math
Frequency Tables
More Statistics Lessons
What is a Histogram?
How to interpret and draw a histogram?
When constructing a histogram with non-uniform (unequal) class widths, we must ensure that the areas of the rectangles are proportional to the class frequencies.
Remember that the histogram differs from a bar chart in that it is the area of the bar that denotes the value, not the height. This means that we would need to consider the widths in order to determine the height of each rectangle.
Example:
The following frequency distribution gives the masses of 48 objects measured to the nearest gram.
Draw a histogram to illustrate the data.
| Mass (g) | 10 – 19 | 20 – 24 | 25 – 34 | 35 – 50 | 51 – 55 |
|---|---|---|---|---|---|
| Frequency | 6 | 4 | 12 | 18 | 8 |
Solution:
Evaluate each class widths.
| Mass (g) | 10 – 19 | 20 – 24 | 25 – 34 | 35 – 50 | 51 – 55 |
|---|---|---|---|---|---|
| Frequency | 6 | 4 | 12 | 18 | 8 |
| Class width | 10 | 5 | 10 | 15 | 5 |
Since the class widths are not equal, we choose a convenient width as a standard and adjust the heights of the rectangles accordingly.
We notice that the smallest width size is 5. We can choose 5 to be the standard width. The other widths are then multiples of the standard width.
The table below shows the calculations of the heights of the rectangles.
| Mass (g) | 10 – 19 | 20 – 24 | 25 – 34 | 35 – 50 | 51 – 55 |
|---|---|---|---|---|---|
| Frequency | 6 | 4 | 12 | 18 | 8 |
| Class widths | 10 | 5 | 10 | 15 | 5 |
| 2 × standard | standard | 2 × standard | 3 × standard | standard | |
| Rectangle’s height in histogram | 6 ÷ 2 = 3 | 4 | 12 ÷ 2 = 6 | 18 ÷ 3 = 6 | 8 |
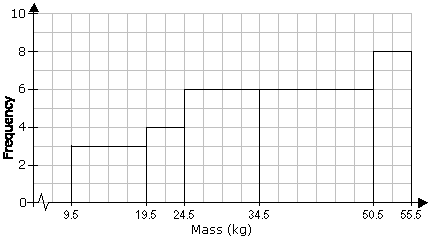
Histogram with uneven class widths
Higher GCSE topic revision
Understand what is a histogram.
Draw a histogram given some information.
Interpret a histogram.
GCSE Maths - Histograms - Unequal Class Intervals - Frequency Density - Higher A/A* grade
Example 1: The table below shows the length in mm of some
worms found in Steve’s garden. Draw a histogram to represent the information.
Example 2: The histogram shows the range of ages of members of a sports centre. Complete the frequency table.
Example 3: The histogram gives information about the heights of 540 Christmas trees. Work out an estimate for the number of Christmas trees with a height greater than 3 metres.
How to find the width and height of a class interval?
Example: The lifetime of a bulb in hours is given in the table.
If the width of the 95-105 class is 2cm and the height is 9cm. Find the width and height of the 105-130 class.
Histogram (unequal class intervals)
Example: The police wants to know how many cars exceed the speed
limit. An officer stands with a speed gun and records the speeds of 1000 consecutive cars.
(a) Identify one possible source of bias for this experiment.
(b) The grouped frequency table represents the speeds of the 1000 cars. On the grid, show the data on a histogram.
(c) The speed limit for the road is 30 miles per hour. Two cars are chosen at random from the 1000 cars. Estimate the probability that both cars are at least 10% above the speed limit.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.