Horizontal Asymptotes of Rational Functions

The following diagrams show how to find the horizontal asymptotes of rational functions. Scroll down the page for more examples and solutions on how to find horizontal asymptotes.

 

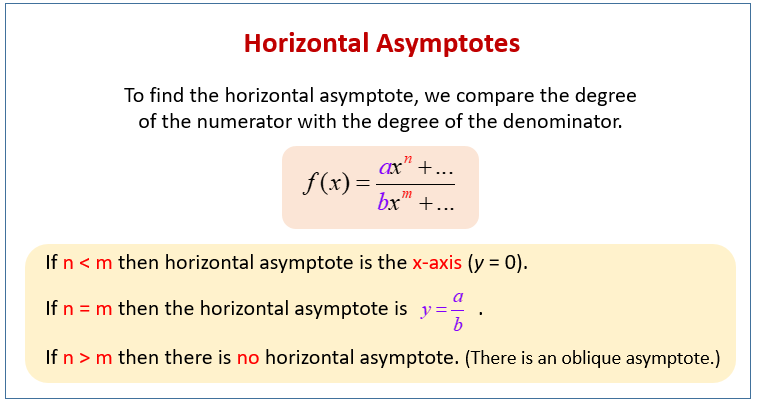
Graphing Rational Functions, n less than m
There are different characteristics to look for when graphing rational functions. When graphing rational functions where the degree of the numerator function is less than the degree of denominator function, we know that y = 0 is a horizontal asymptote. When the degree of the numerator is equal to or greater than that of the denominator, there are other techniques for graphing rational functions.

• Show Video Lesson

Graphing Rational Functions, n = m
There are different characteristics to look for when creating rational function graphs. With rational function graphs where the degree of the numerator function is equal to the degree of denominator function, we can find a horizontal asymptote. When the degree of the numerator is less than or greater than that of the denominator, there are other techniques for drawing rational function graphs.
How to recognize when a rational function has a horizontal asymptote, and how to find its equation.

• Show Video Lesson

Graphing Rational Functions, n > m
There are different characteristics to look for when creating rational function graphs. With rational function graphs where the degree of the numerator function is greater than the degree of denominator function, there is no horizontal asymptote, but there may be an oblique asymptote.

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.