Inscribed And Circumscribed Polygons
In these lessons, we will learn about the properties of inscribed polygons and circumscribed polygons. We will also learn how to solve problems involving inscribed quadrilaterals and inscribed triangles.
Related Pages
Angles of Polygons
Cyclic Quadrilaterals
Circles
What Are Inscribed Or Circumscribed Polygons
An inscribed polygon is a polygon in which all vertices lie on a circle. The polygon is inscribed in the circle and the circle is circumscribed about the polygon. (It is a polygon in a circle)
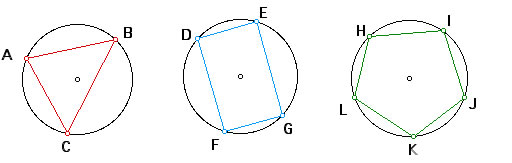
A circumscribed polygon is a polygon in which each side is a tangent to a circle. The circle is inscribed in the polygon and the polygon is circumscribed about the circle. (It is a circle in a polygon)
<img loading=“lazy” src="/image-files/circumscribedpolygon.jpg" alt=“circumscribed polygon”
title=“circumscribed polygon"width=“217” height=“139” />
Inscribed and Circumscribed Polygons
A video lesson on polygons inscribed in and circumscribed about a circle.
The circumcenter of a polygon is the center of a circle circumscribed about a polygon.
The incenter of a polygon is the center of a circle inscribed in the polygon.
If a quadrilateral is inscribed in a circle, its opposite angles are supplementary.
If a parallelogram is inscribed in a circle, it must be a rectangle.
Concyclic is a set of points that must all lie on a circle.
Inscribed Quadrilaterals
Square Inscribed in a Circle
The relationship between a circle and an inscribed square.
For an inscribed square, the diameter of the circle = side of square × square root of 2.
Circles - Inscribed Quadrilaterals
How to find missing angles inside inscribed quadrilaterals?
• The interior angles add up to 360°
• Both pairs of opposite angles are supplementary.
How to solve problems involving quadrilaterals inscribed in circles?
Examples:
- Find the measure of each unknown angle.
- Given m∠X = 110, WZ ≅ YZ, and m∠Y = 100. Find m∠Z.
Inscribed Quadrilaterals and Triangles
A quadrilateral can be inscribed in a circle if and only if its opposite angles are supplementary.
If a right triangle is inscribed in a circle, then the hypotenuse is a diameter of the circle.
Conversely, if one side of an inscribed triangle is a diameter, then the triangle is a right triangle,
and the angle opposite the diameter is a right angle.
Example:
For each inscribed quadrilaterals find the value of each variable.
Cyclic Quadrilaterals
In this lesson we looked at properties of cyclic quadrilaterals.
A cyclic quadrilateral is a four sided figure whose corners are on the edge of a circle.
Properties of a cyclic quadrilateral:
• Opposite angles in a cyclic quadrilateral add to 180°
• Interior opposite angles are equal to their corresponding exterior angle.
Inscribed Triangles
If an inscribed triangle is a right triangle, then the hypotenuse is the diameter. If an inscribed
angle has a diameter as one of its sides, then its a right triangle.
Circles Inscribed in Right Triangles
This problem involves two circles that are inscribed in a right triangle.
Example:
The circle with center A has radius 3 and its tangent to both the positive x-axis and the positive y-axis.
The circle with center B has radius 1 and is tangent to both the x-axis and the circle with center A.
The line L is tangent to both circles. Find the y-intercept of line L.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.