Law of Cosines / Cosine Rule
Related Pages
Law Of Sines
Lessons On Trigonometry
More Algebra 2 Lessons
These lessons with examples, solutions, and videos to help High School students learn to use the law of cosines.
In these lessons, we will learn:
- the Law of Cosines
- how to use the Law of Cosines when given two sides and an included angle
- how to use the Law of Cosines when given three sides
- how to proof the Law of Cosines
- how to solve applications or word problems using the Law of Cosine
Law of Cosines
The Law of Cosines relates the lengths of the sides of a triangle with the cosine of one of its angles.
The Law of Cosines is also sometimes called the Cosine Rule or Cosine Formula.
If we are given two sides and an included angle (SAS) or three sides (SSS) then we can use the Law of Cosines to solve the triangle i.e. to find all the unknown sides and angles.
We can use the Law of Sines to solve triangles when we are given two angles and a side (AAS or ASA) or two sides and a non-included angle (SSA).
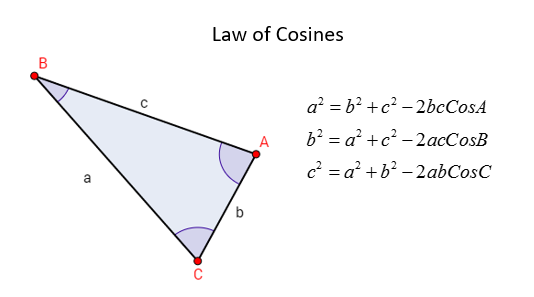
The Law of Cosines, for any triangle ABC is
a2 = b2 + c 2 – 2bccos A
b2 = a2 + c 2 – 2ac cos B
c2 = a2 + b 2 – 2ab cos C
The following diagram shows the Law of Cosines. Scroll down the page if you need more examples and solutions on how to use the Law of Cosines and how to proof the Law of Cosines.
Printable & Online Trigonometry Worksheets
Law of Cosines: Given two sides and an included-angle
Example:
Solve triangle PQR in which p = 6.5 cm, q = 7.4 cm and ∠R = 58°.
Solution:
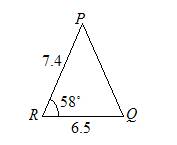
Using the Cosine rule,
r2 = p2 + q2– 2pq cos R
r2 = (6.5)2 + (7.4)2 – 2(6.5)(7.4) cos58°
= 46.03
r = 6.78 cm
Using the Sine rule,

∠Q = 180° – 58° – 54.39°
= 67.61°
∠P = 54.39°, ∠Q = 67.61° and r = 6.78 cm
Law of Cosines: Given three sides
Example:
In triangle ABC, a = 9 cm, b = 10 cm and c = 13 cm. Find the size of the largest angle.
Solution:
The largest angle is the one facing the longest side, i.e. C.
c2 = a2 + b2 – 2ab cos C
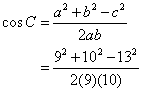
= 0.067
∠C = 86.2°
How to use the cosine rule?
One given SAS and the other given SSS.
Using Law of Cosines to solve triangles given 3 sides (SSS) and 2 sides and the angle in between (SAS).
How to solve an oblique triangle given SSS using the Law of Cosines?
Example:
Solve the triangle using the information given.
a = 3.2, b = 7.6, c = 6.4
Proof of the Law of Cosines
How to prove the law of cosines by using coordinate geometry and the Pythagorean theorem?
Applications using the Law of Cosines
How to solve two word problems using the Law of Cosine?
Example 1:
An engineering firm decides to bid on a proposed tunnel through a mountain. Find how long is the
tunnel and the bid amount.
Example 2:
Find the range of service of a transmission tower.
How to use the Law of Cosines to determine the length across the lake?
Use the Law of Cosines to find the distance a plane has traveled after a change in direction
Example:
A pilot flies in a straight path for 1 h 30 min. She then makes a course correction, heading 8 degrees
to the left and flies 2 h in the new direction. If she maintains a constant speed of 450 mi/h, how far
is she from her starting position?
Use the Law of Cosines to determine the length of a diagonal of a parallelogram
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.