Illustrative Mathematics Grade 8, Unit 8, Lesson 12: Edge Lengths and Volumes

Related Pages
Illustrative Math
Grade 8

Lesson 12: Edge Lengths and Volumes

Let’s explore the relationship between volume and edge lengths of cubes.

Illustrative Math Unit 8.8, Lesson 12 (printable worksheets)

Lesson 12 Summary

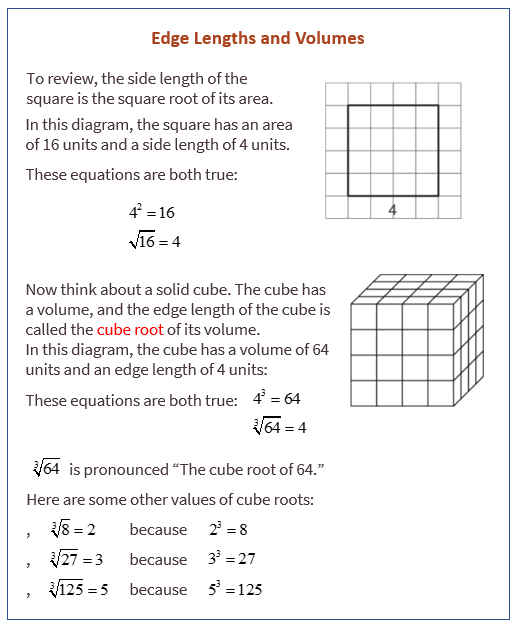
The following diagram explore the relationship between volume and edge lengths of cubes.

Lesson 12.1 Ordering Squares and Cubes

Let a, b, c, d, e, and f be positive numbers.
Given these equations, arrange a, b, c, d, e, and f from least to greatest. Explain your reasoning.

• See Video for Whole Lesson

Lesson 12.2 Name That Edge Length!

Fill in the missing values using the information provided:

Are you ready for more?

A cube has a volume of 8 cubic centimeters. A square has the same value for its area as the value for the surface area of the cube. How long is each side of the square?

• Show Answers

  A cube that has a volume of 8 cubic centimeters would have an edge length of 2 cm.
  Surface area of the cube = 2 · 2 · 6 = 24 square cm.
  The side of the square would be √24 = 2√6 cm.

Lesson 12.3 Card Sort: Rooted in the Number Line

Your teacher will give your group a set of cards. For each card with a letter and value, find the two other cards that match. One shows the location on a number line where the value exists, and the other shows an equation that the value satisfies. Be prepared to explain your reasoning.

Lesson 12 Practice Problems

1. a. What is the volume of a cube with a side length of
   b. What is the side length of a cube with a volume of
2. Write an equivalent expression that doesn’t use a cube root symbol.
3. Find the distance between each pair of points. If you get stuck, try plotting the points on graph paper.
4. Here is a 15-by-8 rectangle divided into triangles. Is the shaded triangle a right triangle? Explain or show your reasoning.
5. Here is an equilateral triangle. The length of each side is 2 units. A height is drawn. In an equilateral triangle, the height divides the opposite side into two pieces of equal length.
   a. Find the exact height.
   b. Find the area of the equilateral triangle.
   c. (Challenge) Using x for the length of each side in an equilateral triangle, express its area in terms of x.

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.