Illustrative Mathematics Grade 8, Unit 2, Lesson 11: Writing Equations for Lines

Related Pages
Illustrative Math
Grade 8

Lesson 11: Writing Equations for Lines

Let’s explore the relationship between points on a line and the slope of the line.

Illustrative Math Unit 8.2, Lesson 11 (printable worksheets)

Lesson 11 Summary

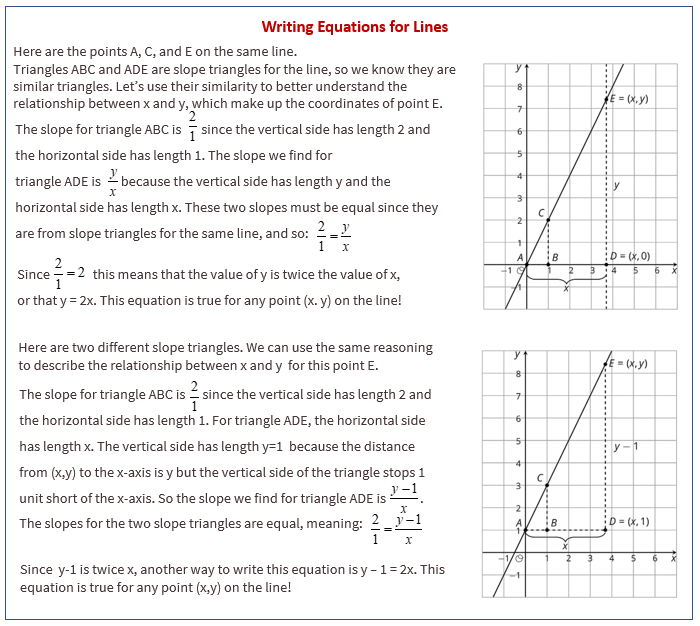
The following diagram shows the relationship between points on a line and the slope of the line.

Lesson 11.1 Coordinates and Lengths in the Coordinate Plane

Find each of the following and explain your reasoning:

1. The length of segment BE.
2. The coordinates of E.

• See Video for Whole Lesson

Lesson 11.2 What We Mean by an Equation of a Line

Line j is shown in the coordinate plane.

1. What are the coordinates of B and D?
2. Is point (20,15) on line j? Explain how you know.
3. Is point (100,75) on line j? Explain how you know.
4. Is point (90,68) on line j? Explain how you know.
5. Suppose you know the x- and y-coordinates of a point. Write a rule that would allow you to test whether the point is on line j.

Lesson 11.3 Writing Relationships from Slope Triangles

Here are two diagrams:

1. Complete each diagram so that all vertical and horizontal segments have expressions for their lengths.
2. Use what you know about similar triangles to find an equation for the quotient of the vertical and horizontal side lengths of triangle DFE in each diagram.

Are you ready for more?

1. Find the area of the shaded region by summing the areas of the shaded triangles.
   • Show Answer

     Area = (1/2 · 24 · 15) + 2 · (1/2 · 5 · 10)
     = 230

2. Find the area of the shaded region by subtracting the area of the unshaded region from the large triangle.
   • Show Answer

     Area = (1/2 · 34 · 25) - 10 · 24
     = 185

3. What is going on here?
   • Show Answer

     The 2 areas are not the same.
     This because there is an error in the diagram.
     The side of the larger triangle does not form a straight line because the slope 10/5 does not equal the slope 15/12.

Lesson 11 Practice Problems

1. For each pair of points, find the slope of the line that passes through both points. If you get stuck, try plotting the points on graph paper and drawing the line through them with a ruler.
2. Line l is shown in the coordinate plane.
   a. What are the coordinates of B and D?
   b. Is the point (16,20) on line l? Explain how you know.
   c. Is the point (20,24) on line l? Explain how you know.
   d. Is the point (80,100) on line l? Explain how you know.
   e. Write a rule that would allow you to test whether (x,y) is on line l.
3. Consider the graphed line.
   Mai uses Triangle A and says the slope of this line is 6/4. Elena uses Triangle B and says no, the slope of this line is 1.5. Do you agree with either of them? Explain.
4. A rectangle has length 6 and height 4.
   Which of these would tell you that quadrilateral is definitely not similar to this rectangle?
   Select all that apply.

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.