Linear Sequences
Related Pages
More Lessons for GCSE Maths
Math Worksheets
Examples, videos, solutions, activities and worksheets that are suitable for GCSE Maths.
What is a sequence?
A sequence is a list of numbers that follow a pattern.
What is a linear sequence?
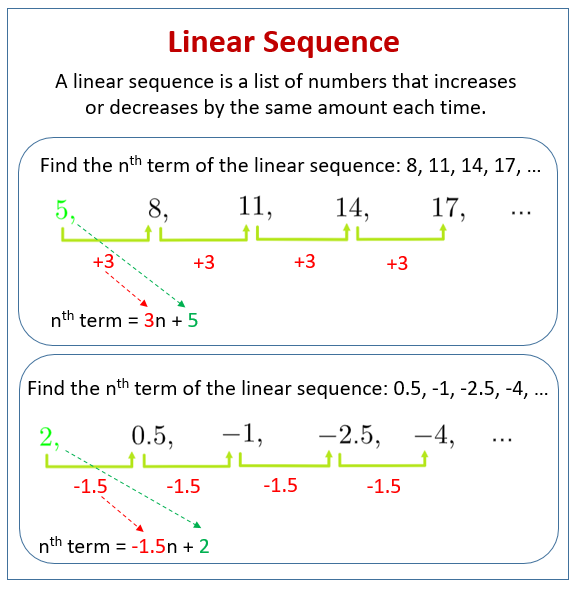
A linear sequence is a list of numbers that increases or decreases by the same amount each time.
How to find the nth term of a linear sequence?
The following diagrams show how to find the nth term of a linear sequence. Scroll down the page for more examples and solutions on finding and using the nth term of a linear sequence.
Sequence Worksheets
Practice your skills With the following worksheets:
Steps to Find the nth Term
- Find the Common Difference (d):
Subtract any term from the term that follows it.
d = (any term) - (the term before it) - Find the First Term (a):
Identify the first term in the sequence. - Use the Formula:
The nth term (an) of a linear sequence is given by:
an = a + (n - 1)d
Where:
an is the nth term.
a is the first term.
n is the term number.
d is the common difference. - Simplify the formula to get the nth term.
How to describe a linear sequence?
How to find the next few terms?
How to find the nth term?
How to use the nth term to find a term in the sequence?
Linear Sequences (nth term)
Finding and using the nth term of a linear sequence
The nth term of a simple linear sequence
linear sequences - finding the nth term.
Linear sequences
Linear sequences 2
Key Points:
- If the common difference (d) is positive, the sequence is increasing.
- If the common difference (d) is negative, the sequence is decreasing.
- The nth term formula allows you to find any term in the sequence.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.