Lines of Symmetry

New York State Common Core Math Grade 4, Module 4, Lesson 12

Worksheets for Grade 4

The following diagram shows a worksheet to learn how to draw lines of symmetry for given figures. Scroll down the page for more examples of lines of symmetry.

Click on the following worksheet to get more lines of symmetry printable documents (pdf) and answers.

 

Lesson 12 Concept Development

• Problem 1: Recognize folded symmetry.
• Problem 2: Identify lines of symmetry in familiar figures.
• Problem 3: Draw lines of symmetry.

Problem Set

1. Circle the figures that have a correct line of symmetry drawn.
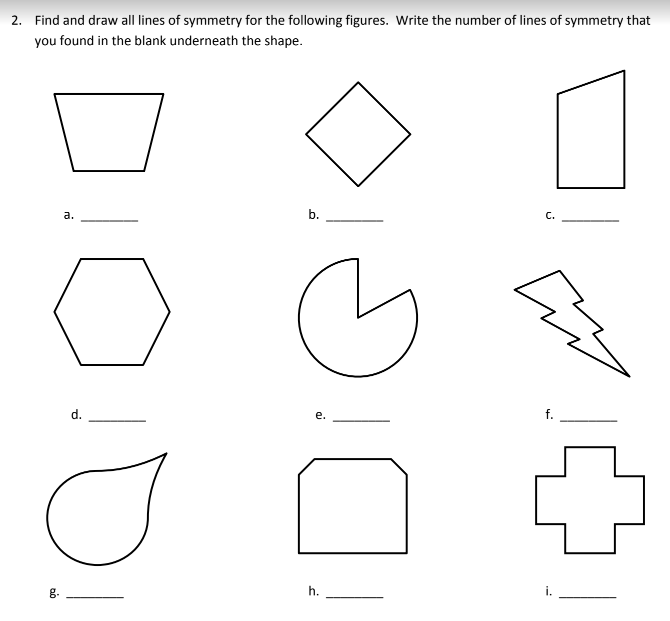
2. Find and draw all lines of symmetry for the following figures. Write the number of lines of symmetry that you found in the blank underneath the shape.
3. Half of each figure below has been drawn. Use the line of symmetry, represented by the dashed line, to complete each figure.
4. The figure below is a circle. How many lines of symmetry does the figure have? Explain.
   

• Show Video Lesson

NYS Math Module 4 Grade 4 Lesson 12 Homework

1. Circle the figures that have a correct line of symmetry drawn.
2. Find and draw all lines of symmetry for the following figures. Write the number of lines of symmetry that you found in the blank underneath the shape.
3. Half of each figure below has been drawn. Use the line of symmetry, represented by the dashed line, to complete each figure.
4. Is there another shape that has the same number of lines of symmetry as a circle? Explain.

• Show Video Lesson

• Show Video Lesson

Common Shapes and Their Lines of Symmetry:

1. Circle: A circle has an infinite number of lines of symmetry. Any line that passes through the center of the circle divides it into two identical halves.
   
2. Square:
   A square has four lines of symmetry:
   One horizontal line through the center.
   One vertical line through the center.
   Two diagonal lines connecting opposite corners.
   
3. Rectangle:
   A rectangle has two lines of symmetry:
   One horizontal line through the center.
   One vertical line through the center.
   
4. Equilateral Triangle:
   An equilateral triangle (all sides equal) has three lines of symmetry. Each line runs from a vertex (corner) to the midpoint of the opposite side.
   
5. Isosceles Triangle:
   An isosceles triangle (two sides equal) has one line of symmetry. This line runs from the vertex between the two equal sides to the midpoint of the opposite side.
   
6. Scalene Triangle:
   A scalene triangle (no sides equal) has no lines of symmetry.
   
7. Rhombus:
   A rhombus has two lines of symmetry. Those lines run from corner to opposite corner, diagonally through the shape.
   
8. Kite:
   A kite has one line of symmetry. That line runs down the center of the two sets of equal length sides.
   
9. Regular Polygons:
   In general, a regular polygon (all sides and angles equal) has the same number of lines of symmetry as it has sides. For example:
   A regular pentagon has 5 lines of symmetry.
   A regular hexagon has 6 lines of symmetry.
   

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.