Illustrative Mathematics Grade 6, Unit 8, Lesson 10: Finding and Interpreting the Mean as the Balance Point

Related Pages
Illustrative Math
Grade 6

Lesson 10: Finding and Interpreting the Mean as the Balance Point

Let’s look at another way to understand the mean of a data set.

Illustrative Math Unit 6.8, Lesson 10 (printable worksheets)

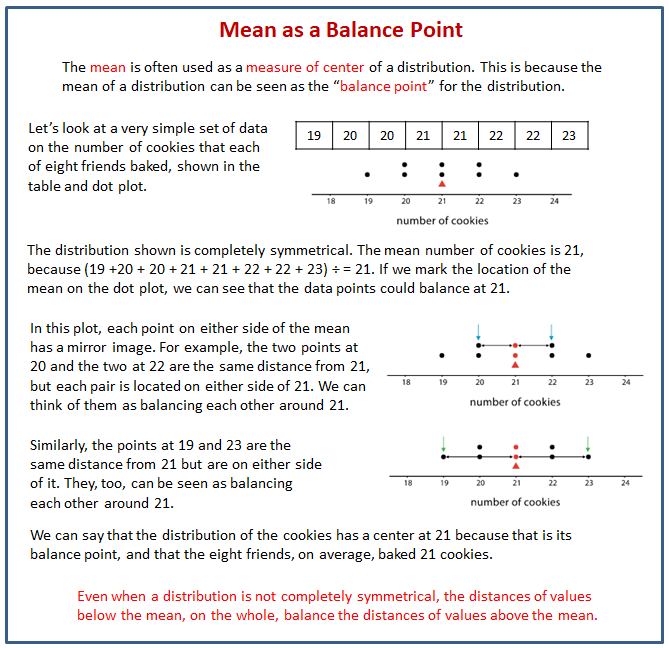
Lesson 10 Summary

The following diagram explains how the mean represents a balance point for the data on a dot plot.

Lesson 10.1 Which One Doesn’t Belong: Division

Which expression does not belong? Be prepared to explain your reasoning.

• See Video for Whole Lesson

Lesson 10.2 Travel Times (Part 1)

Here is the data set from an earlier lesson showing how long it takes for Diego to walk to school, in minutes, over 5 days. The mean number of minutes was 11.

1. Represent Diego’s data on a dot plot. Mark the location of the mean with a triangle.
   
2. The mean can also be seen a measure of center that balances the points in a data set. If we find the distance between every point and the mean, add the distances on each side of the mean, and compare the two sums, we can see this balancing.
   a. Record the distance between each point and 11 and its location relative to 11.
   b. Sum of distances left of 11:___________ Sum of distances right of 11:___________
   What do you notice about the two sums?
   
3. Can another point that is not the mean produce similar sums of distances?
   Let’s investigate whether 10 can produce similar sums as those of 11.
   a. Complete the table with the distance of each data point from 10.
   b. Sum of distances left of 10:___________ Sum of distances right of 10:___________
   What do you notice about the two sums?
   
4. Based on your work so far, explain why the mean can be considered a balance point for the data set.
   

Lesson 10.3 Travel Times (Part 2)

1. Here are dot plots showing how long Diego’s trips to school took in minutes—which you studied earlier—and how long Andre’s trips to school took in minutes. The dot plots include the means for each data set, marked by triangles.
   a. Which of the two data sets has a larger mean? In this context, what does a larger mean tell us?
   b. Which of the two data sets has larger sums of distances to the left and right of the mean? What do these sums tell us about the variability in Diego’s and Andre’s travel times?
   
2. Here is a dot plot showing lengths of Lin’s trips to school.
   a. Calculate the mean of Lin’s travel times.
   b. Complete the table with the distance between each point and the mean as well as whether the point is to the left or right of the mean.
   c. Find the sum of distances to the left of the mean and the sum of distances to the right of the mean.
   d. Use your work to compare Lin’s travel times to Andre’s. What can you say about their average travel times? What about the variability in their travel times?
   

Glossary Terms

measure of center
A measure of center is a value that seems typical for a data distribution.
Mean and median are both measures of center.

Lesson 9 Practice Problems

1. A preschool teacher is rearranging four boxes of playing blocks so that each box contains an equal number of blocks. Currently Box 1 has 32 blocks, Box 2 has 18, Box 3 has 41, and Box 4 has 9.
   Select all the ways he could make each box have the same number of blocks.
   A. Remove all the blocks and make four equal piles of 25, then put each pile in one of the boxes.
   B. Remove 7 blocks from Box 1 and place them in Box 2.
   C. Remove 21 blocks from Box 3 and place them in Box 4.
   D. Remove 7 blocks from Box 1 and place them in Box 2, and remove 21 blocks from Box 3 and place them in Box 4.
   E. Remove 7 blocks from Box 1 and place them in Box 2, and remove 16 blocks from Box 3 and place them in Box 4.
2. In a round of mini-golf, Clare records the number of strokes it takes to hit the ball into the hole of each green. She said that, if she redistributed the strokes on different greens, she could tell that her average number of strokes per hole is 3.
   Explain how Clare is correct.
   
3. Three sixth-grade classes raised $25.50, $49.75, and $37.25 for their classroom libraries. They agreed to share the money raised equally. What is each class’s equal share? Explain or show your reasoning.
   
4. In her English class, Mai’s teacher gives 4 quizzes each worth 5 points. After 3 quizzes, she has the scores 4, 3, and 4. What does she need to get on the last quiz to have a mean score of 4? Explain or show your reasoning.
   
5. An earthworm farmer examined two containers of a certain species of earthworm so that he could learn about their lengths. He measured 25 earthworms in each container and recorded their lengths in millimeters.
   Here are histograms of the lengths for each container.
   a. Which container tends to have longer worms than the other container?
   b. For which container would 15 millimeters be a reasonable description of a typical length of the worms in the container?
   c. If length is related to age, which container had the most young worms?
   
6. Diego thinks that x = 3 is a solution to the equation x² = 16. Do you agree? Explain or show your reasoning.
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.