Illustrative Mathematics Grade 6, Unit 8, Lesson 12: Using Mean and MAD to Make Comparisons

Related Pages
Illustrative Math
Grade 6

Lesson 12: Using Mean and MAD to Make Comparisons

Let’s use mean and MAD to describe and compare distributions.

Illustrative Math Unit 6.8, Lesson 12 (printable worksheets)

Lesson 12 Summary

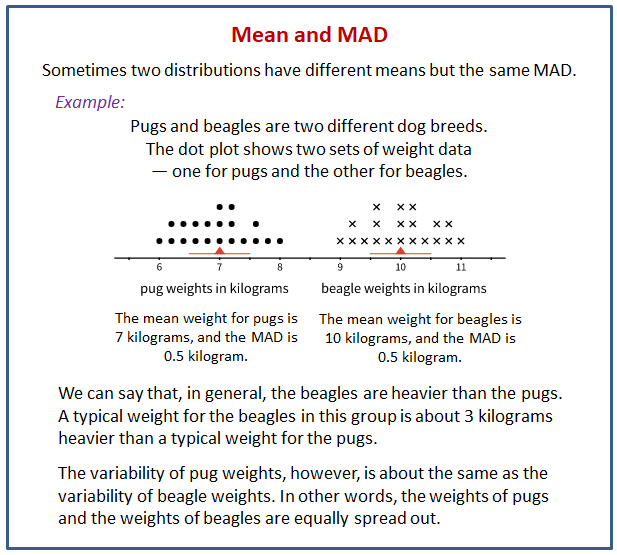
The following diagram explains how to use means and MADs to compare groups.

Lesson 12.1 Number Talk: Decimal Division

Find the value of each expression mentally.
24 ÷ 12
2.4 ÷ 12
44.4 ÷ 12
46.8 ÷ 12

• See Video for Whole Lesson

Lesson 12.2 Which Player Would You Choose?

1. Andre and Noah joined Elena, Jada, and Lin in recording their basketball scores. They all recorded their scores in the same way: the number of baskets made out of 10 attempts. Each collected 12 data points.
   Andre’s mean number of baskets was 5.25, and his MAD was 2.6. Noah’s mean number of baskets was also 5.25, but his MAD was 1.
   Here are two dot plots that represent the two data sets. The triangle indicates the location of the mean.
   a. Without calculating, decide which dot plot represents Andre’s data and which represents Noah’s. Explain how you know.
   b. If you were the captain of a basketball team and could use one more player on your team, would you choose Andre or Noah? Explain your reasoning.
   
2. An eighth-grade student decided to join Andre and Noah and kept track of his scores. His data set is shown
   here. The mean number of baskets he made is 6.
   a. Calculate the MAD. Show your reasoning.
   b. Draw a dot plot to represent his data and mark the location of the mean with a triangle ().
   c. Compare the eighth-grade student’s mean and MAD to Noah’s mean and MAD. What do you notice?
   d. Compare their dot plots. What do you notice about the distributions?
   e. What can you say about the two players’ shooting accuracy and consistency?
   

Are you ready for more?

Invent a data set with a mean of 7 and a MAD of 1.

• Show Answer

  Example:
  5.5, 7, 8.5
  Mean = (5.5 + 7 + 8.5)/3 = 7
  MAD = (1.5 + 1 + 1.5)/3 = 1

Lesson 12.3 Swimmers Over the Years

In 1984, the mean age of swimmers on the U.S. women’s swimming team was 18.2 years and the MAD was 2.2 years. In 2016, the mean age of the swimmers was 22.8 years, and the MAD was 3 years.

1. How has the average age of the women on the U.S. swimming team changed from 1984 to 2016? Explain your reasoning.
   
2. Are the swimmers on the 1984 team closer in age to one another than the swimmers on the 2016 team are to one another? Explain your reasoning.
   
3. Here are dot plots showing the ages of the women on the U.S. swimming team in 1984 and in 2016. Use them to make two other comments about how the women’s swimming team has changed over the years.
   

Lesson 12 Practice Problems

1. The dot plots show the amounts of time that ten U.S. students and ten Australian students took to get to school. Which statement is true about the MAD of the Australian data set?.
   A. It is significantly less than the MAD of the U.S. data set.
   B. It is exactly equal to the MAD of the U.S. data set.
   C. It is approximately equal to the MAD of the U.S. data set.
   D. It is significantly greater than the MAD of the U.S. data set.
   
2. The dot plots show the amounts of time that ten South African students and ten Australian students took to get to school. Without calculating, answer the questions.
   a. Which data set has the smaller mean? Explain your reasoning.
   b. Which data set has the smaller MAD? Explain your reasoning.
   c. What does a smaller mean tell us in this context?
   d. What does a smaller MAD tell us in this context?
   
3. Two high school basketball teams have identical records of 15 wins and 2 losses. Sunnyside High School’s mean score is 50 points and its MAD is 4 points. Shadyside High School’s mean score is 60 points and its MAD is 15 points.
   Lin read the records of each team’s score. She likes the team that had nearly the same score for every game it played. Which team do you think Lin likes? Explain your reasoning.
   
4. Jada thinks the perimeter of this rectangle can be represented with the expression a + a + b + b. Andre thinks it can be represented with 2a + 2b.
   
5. Draw a number line.
   a. Plot and label three numbers between -2 and -8 (not including -2 and -8).
   b. Use the numbers you plotted and the symbols < and > to write three inequality statements.
   
6. Adult elephant seals generally weigh about 5,500 pounds. If you weighed 5 elephant seals, would you expect each seal to weigh exactly 5,500 pounds? Explain your reasoning.
   Do you agree with either, both, or neither of them? Explain your reasoning.
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.