Median From Frequency Tables
In these lessons, we will learn:
- how to find the median of a frequency table when the number of observations is odd.
- how to find the median of a frequency table when the number of observations is even.
- how to find the median for both discrete and grouped data.
Related Pages
Median
Mean And Mode From The Frequency Table
Central Tendency
More Statistics Lessons
What Is The Median?
The median is the middle value in an ordered set of data.
In a frequency table, the observations are already arranged in an ascending order. We can obtain the median by looking for the value in the middle position.
If there is an odd number of observations, the median is the middle number.
If there is an even number of observations, the median will be the mean of the two central numbers.
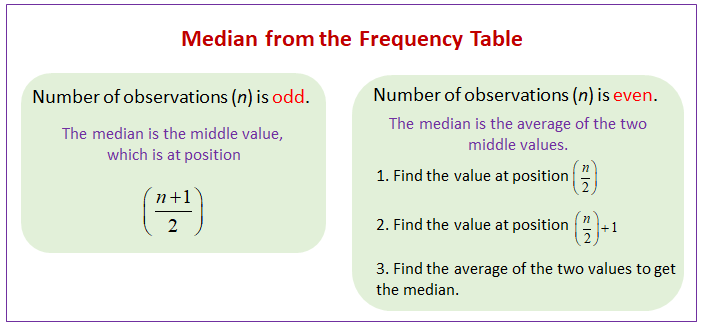
The following table shows how to find the median from the frequency table with odd number of observations and with even number of observations. Scroll down the page for examples and step-by-step solutions.
How To Find The Median Of A Frequency Table When The Number Of Observations Is Odd?
Case 1. When the number of observations (n) is odd, then
the median is the value at the 
position.
Example:
The following is a frequency table of the score obtained in a mathematics quiz. Find the median score.
| Score | 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| Frequency | 3 | 4 | 7 | 6 | 3 |
Solution:
Number of scores = 3 + 4 + 7 + 6 + 3 = 23 (odd number)
Since the number of scores is odd, the median is at the 
position.
To find out the 12 th position, we need to add up the frequencies as shown:
| Score | 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| Frequency | 3 | 4 | 7 | 6 | 3 |
| Position | 3 | 3 + 4 = 7 | 7 + 7 =14 |
The 12th position is after the 7th position but before the 14th position. So, the median is 2.
How To Find The Median Of A Frequency Table When The Number Of Observations Is Even?
Case 2. When the number of observations (n) is even, then the median is the average of values at the n/2 and (n/2 + 1) positions.
Example:
The table is a frequency table of the scores obtained in a competition. Find the median score.
| Scores | 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| Frequency | 11 | 9 | 5 | 10 | 15 |
Solution:
Number of scores = 11 + 9 + 5 + 10 + 15 = 50 (even number)
Since the number of scores is even, the median is at the average of the 
position and 
position.
To find out the 25th position and 26th position, we add up the frequencies as shown:
| Scores | 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| Frequency | 11 | 9 | 5 | 10 | 15 |
| Position | 11 | 11 + 9 = 20 | 20 + 5 = 25 | 25 + 10 = 35 | 36 to 50 |
The score at the 25th position is 2 and the score at the 26th position is 3.
The median is the average of the scores at 25th and 26th positions = 
How To Find The Median From A Frequency Table (n is even)?
How to find the Mean, Median and Mode from a frequency distribution table?
Example:
The one hundred families in a particular neighborhood are asked their annual household income, to the
nearest $5 thousand dollars. The results are summarized in a frequency table. Find the median household
income.
How To Find The Median Of A Frequency Table (n is odd)?
How To Find The Mean, Mode And Median From A Frequency Distribution Table For Both Discrete And Grouped Data?
How To Estimate The Median, Quartiles From A Grouped Frequency Table Or Class Intervals?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.