Illustrative Mathematics Grade 6, Unit 8, Lesson 13: The Median of a Data Set

Related Pages
Illustrative Math
Grade 6

Lesson 13: The Median of a Data Set

Let’s explore the median of a data set and what it tells us.

Illustrative Math Unit 6.8, Lesson 13 (printable worksheets)

Lesson 13 Summary

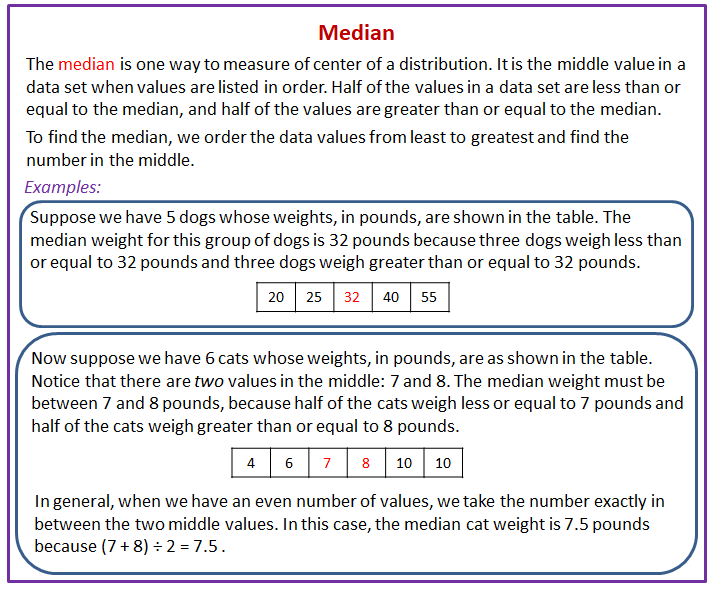
The following diagram explains what the median represents and how to find the median for a set of data.

Lesson 13.1 The Plot of the Story

1. Here are two dot plots and two stories. Match each story with a dot plot that could represent it. Be prepared to explain your reasoning.

• Twenty people—high school students, parents, guardians, and teachers—attended a rehearsal for a high school musical. The mean age was 38.5 years and the MAD was 16.5 years.
• High school soccer team practice is usually watched by family members of the players. One evening, twenty people watched the team practice. The mean age was 38.5 years and the MAD was 12.7 years.
  

2. Another evening, twenty people watched the soccer team practice. The mean age was similar to that from the first evening, but the MAD was greater (about 20 years).
   Make a dot plot that could illustrate the distribution of ages in this story.
   

• See Video for Whole Lesson

Lesson 13.2 Siblings in the House

Here is a table that shows the numbers of siblings of ten students in Tyler’s class.

1. Represent the data shown in the table with a dot plot.
2. Based on your dot plot, estimate the center of the data without making any calculations. What is your estimate of a typical number of siblings of these sixth-grade students? Mark the location of that number on your dot plot.
3. Find the mean. Show your reasoning.
4. a. How does the mean compare to the value that you marked on the dot plot as a typical number of siblings? (Is the mean that you calculated a little larger, a lot larger, exactly the same, a little smaller, or a lot smaller than your estimate?)
   b. Do you think the mean summarizes the data set well? Explain your reasoning.
   

Are you ready for more?

Invent a data set with a mean that is significantly lower than what you would consider a typical value for the data set.

• Show Answer

  Example:
  1, 20, 21
  Mean = (1 + 20 + 20 + 21)/4 = 15.5
  

Lesson 13.3 Finding the Middle

1. Your teacher will give you an index card. Write your first and last names on the card. Then record the total number of letters in your name. After that, pause for additional instructions from your teacher.
   
2. a. Here is the data set on numbers of siblings from an earlier activity. Sort the data from least to greatest, and then find the median.
   b. In this situation, do you think the median is a good measure of a typical number of siblings for this group? Explain your reasoning.
   
3. a. Here is the dot plot showing the travel time, in minutes, of Elena’s bus rides to school. Find the median travel time. Be prepared to explain your reasoning.
   b. What does the median tell us in this context?
   

Glossary Terms

median
The median is one way to measure the center of a data set. It is the middle number when the data set is listed in order.
For the data set 7, 9, 12, 13, 14, the median is 12.
For the data set 3, 5, 6, 8, 11, 12, there are two numbers in the middle. The median is the average of these two numbers. 6 + 8 = 14 and 14 ÷ 2 = 7.

Lesson 13 Practice Problems

1. Here is a table that shows student’s scores for 10 rounds of a video game.
   What is the median score?
   A. 125
   B. 145
   C. 147
   D. 50
   
2. When he sorts the class’s scores on the last test, the teacher notices that exactly 12 students scored better than Clare and exactly 12 students scored worse than Clare. Does this mean that Clare’s score on the test is the median? Explain your reasoning.
   
3. The medians of the following dot plots are 6, 12, 13, and 15, but not in that order. Match each dot plot with its median.
   
4. Invent a data set with five numbers that has a mean of 10 and a median of 12.
   
5. Ten sixth-grade students reported the hours of sleep they get on nights before a school day. Their responses are recorded in the dot plot.
   Looking at the dot plot, Lin estimated the mean number of hours of sleep to be 8.5 hours. Noah’s estimate was 7.5 hours. Diego’s estimate was 6.5 hours.
   Which estimate do you think is best? Explain how you know.
   
6. In one study of wild bears, researchers measured the weights, in pounds, of 143 wild bears that ranged in age from newborn to 15 years old. The data were used to make this histogram.
   a. What can you say about the heaviest bear in this group?
   What is a typical weight for the bears in this group?
   b. What can you say about the heaviest bear in this group?
   What is a typical weight for the bears in this group?
   c. Do more than half of the bears in this group weigh less than 250 pounds?
   d. If weight is related to age, with older bears tending to have greater body weights, would you say that there were more old bears or more young bears in the group? Explain your reasoning.
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.