Algebra: Mixture Word Problems

What Are Mixture Problems?

Mixture problems are word problems where items or quantities of different values are mixed together.

Sometimes different liquids are mixed together changing the concentration of the mixture as shown in example 1, example 2 and example 3.

At other times, quantities of different costs are mixed together as shown in [example 4](#mix

We recommend using a table to organize your information for mixture problems. Using a table allows you to think of one number at a time instead of trying to handle the whole mixture problem at once.

We will show you how it is done by the following examples of mixture problems:

• Adding to the Solution
  
• Removing from the Solution
  
• Replacing the Solution
  
• Mixing Quantities of Different Costs

How To Solve Mixture Problems When We Are Adding To The Solution?

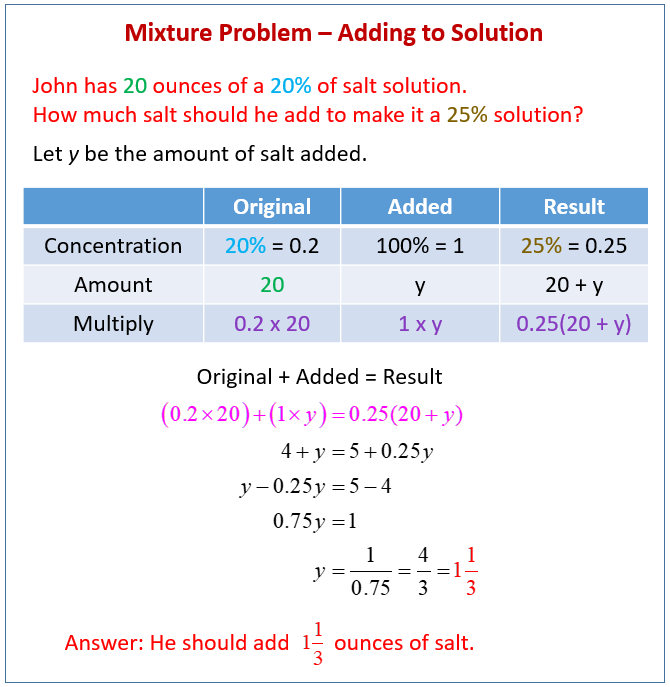
Mixture Problems: Example 1:
John has 20 ounces of a 20% of salt solution. How much salt should he add to make it a 25% solution?

Solution:
Set up a table for salt using the information from the question.

Printable & Online Algebra Worksheets

How To Solve Mixture Problems When We Are Removing From The Solution?

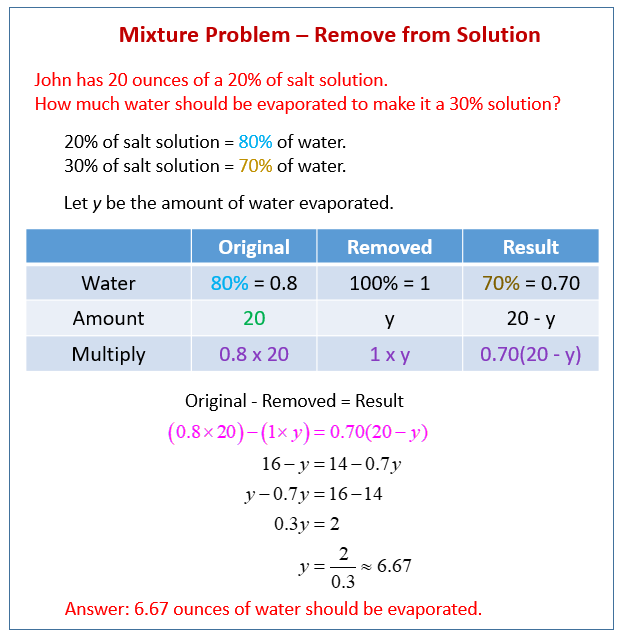
Mixture Problems: Example 2:
John has 20 ounces of a 20% of salt solution. How much water should he evaporate to make it a 3 0% solution?

Solution:
Set up a table for water. The water is removed from the original solution.

How To Solve Mixture Problems When We Are Replacing The Solution?

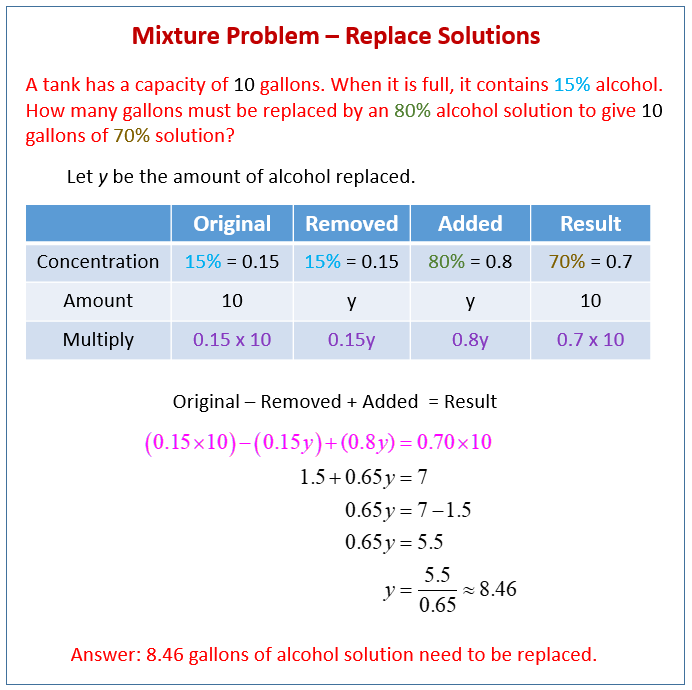
Mixture Problems: Example 3:
A tank has a capacity of 10 gallons. When it is full, it contains 15% alcohol. How many gallons must be replaced by an 80% alcohol solution to give 10 gallons of 70% solution?

Solution:
Set up a table for alcohol. The alcohol is replaced: an amount of 15% alcohol is removed and the same amount of 80% alcohol is added.

Mixture Word Problems: Mixing Quantities Of Different Costs

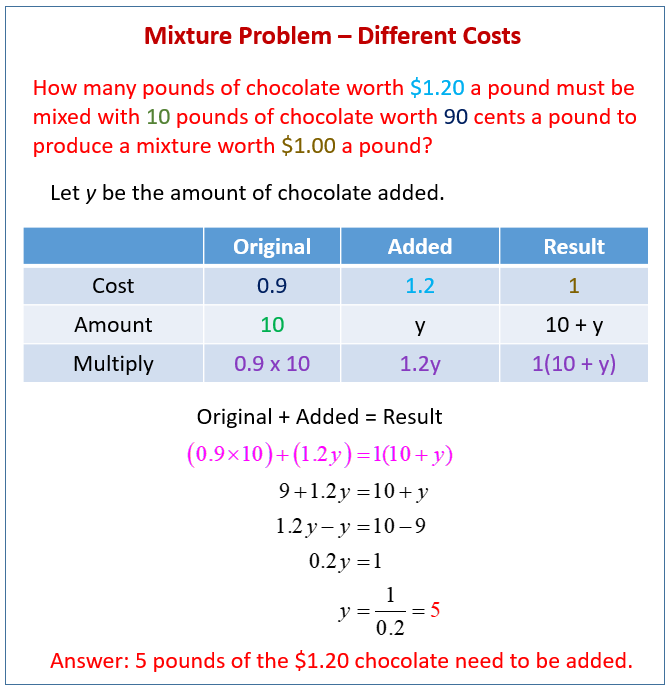
Mixture Problems: Example 4:
How many pounds of chocolate worth $1.20 a pound must be mixed with 10 pounds of chocolate worth 90 cents a pound to produce a mixture worth $1.00 a pound?

Solution:
Set up a table for the chocolates with different costs.

How To Set Up And Solve Mixture Word Problems?

Some word problems using systems of equations involve mixing two quantities with different prices. To solve mixture problems, knowledge of solving systems of equations. is necessary. Most often, these problems will have two variables, but more advanced problems have systems of equations with three variables. Other types of word problems using systems of equations include rate word problems and work word problems.

How To Solve Acid Solution Problems?

Example:
The mad scientist has one solution that is 30% acid and another solution that is 18% acid. How much of each should he use to get 300 L of a solution that is 21% acid?

• Show Video Lesson

Example:
How much pure acid must be mixed with 200 mL of 5% acid to get a 25% acid?

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.