Math Statistics: Mode, Median and Mean

Related Pages
Mean
Median
Central Tendency
More Statistics Lessons

What Is Mode, Median And Mean?

In statistics, mode, median and mean are typical values to represent a pool of numerical observations. They are calculated from the pool of observations.

Mode is the most common value among the given observations. For example, a person who sells ice creams might want to know which flavor is the most popular.

Median is the middle value, dividing the number of data into 2 halves. In other words, 50% of the observations is below the median and 50% of the observations is above the median.

Mean is the average of all the values. For example, a teacher may want to know the average marks of a test in his class.

The following diagrams show how to find the mean, median, mode and range. Scroll down the page for examples and solutions.

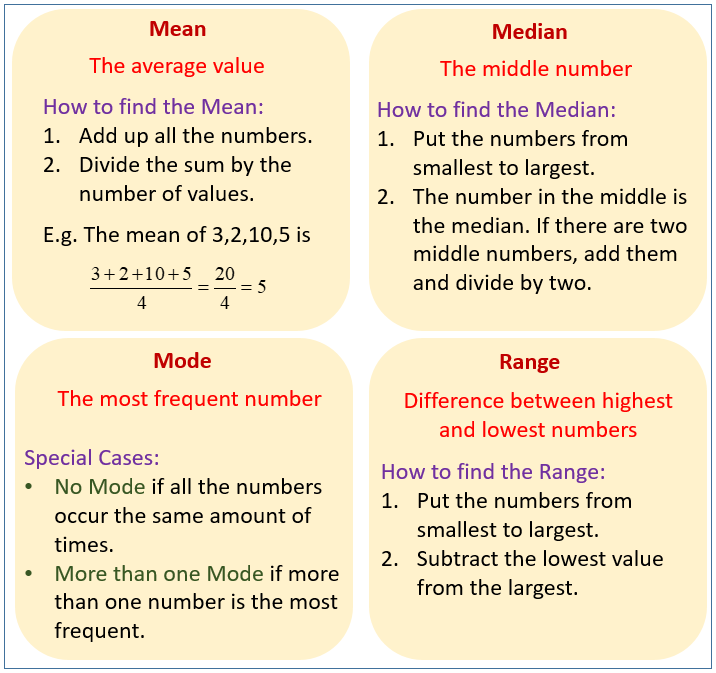
Mode

The mode of a set of observations is the value that occurs most frequently in the set. A set of observations may have no mode, one mode or more than one mode.

Example:
Find the mode of the following set of scores.
14 11 15 9 11 15 11 7 13 12

Solution:
The mode is 11 because 11 occurred more times than the other numbers.

If the observations are given in the form of a frequency table, the mode is the value that has the highest frequency.

Example:
Find the mode of the following set of marks.

Marks	1	2	3	4	5
Frequency	6	7	7	5	3

Solution:
The marks 2 and 3 have the highest frequency. So, the modes are 2 and 3.

Note: The above example shows that a set of observations may have more than one mode.

Example:
Find the mode for each of the following frequency tables:
The frequency table below shows the weights of different bags of rice.

Weight (kg)	45	50	55	60	65	70	75	80
Bags of rice (Frequency)	8	11	7	10	9	10	12	8

There are 8 number cards with values 0 – 7. Each time a card is drawn at random and the card value is recorded. The frequency refers to the number of times a value is shown.

Card values	0	1	2	3	4	5	6	7
Frequency	8	12	7	10	12	13	12	10

Solution:
a) Mode: 75 kg (highest frequency of 12)
b) Mode: 5 (highest frequency of 13)

Example:
The following frequency table shows the marks obtained by students in a quiz. Given that 4 is the mode, what is the least value for x?

Marks	1	2	3	4	5	6
Number of students (Frequency)	7	9	10	x	9	11

Solution:
x is as least 12
(if x is less than 12 then 4 will not be the mode)

Mean, Median, Mode, Range, Interquartile Range

This lesson shows you how to find the mean, median, mode, range and interquartile range for a list of numbers.

Example:
Find the mean, median, mode, range and interquartile range for the following data.
5, 7, 9, 9, 10, 11, 11, 11, 12

• Show Video Lesson

Frequency Table - Mean, Median, Mode, Range And Interquartile Range

This lesson shows you how to find the mean, median, mode, range and interquartile range from a frequency table.

• Show Video Lesson

Mean, Mode And Median From Frequency Tables

How to find the mean, mode and median from a frequency table for both discrete and grouped data?

For grouped data:

• Mean: multiply midpoints by frequencies and add the sub-totals. Divide by the total of the frequencies.
• Mode: find the largest frequency - the corresponding value is the modal value or modal class.
• Median: calculate a running total of the frequencies - the first interval that is above half the total contains the median.

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.