

Money Word Problems
Compound Interest Word Problems
Other Algebra Word Problems
First, we will look at a money word problem involving calculating Simple Interest. Simple Interest word problems are based on the formula for Simple Interest and the formula for Amount. Then, we will look at a money word problem that involves coins and dollar bills.
The following diagram gives the Simple Interest Formula. Scroll down the page for more examples and solutions on how to use the Simple Interest Formula to solve simple interest word problems.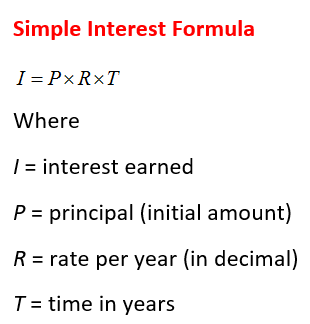
Simple Interest Word Problems
Formula for Simple Interest
i = prt
i represents the interest earned.
p represents the principal which is the number of dollars invested.
r represents the interest rate per year.
t represents the time the money is invested which is generally stated in years or fractions of a year.
Formula for Amount
A represents what your investment is worth if you consider the total amount of the original investment (p) and the interest earned (i)
Example:
James needs interest income of $5,000. How much money must he invest for one year at 7%? (Give your answer to the nearest dollar)
Solution:
5,000 = p(0.07)(1)
p = 71,428.57
He must invest $71,429
Simple Interest Word Problems
Example:Pam invested $5000. She earned 14% on part of her investment and 6% on the rest. If she earned a total of $396 in interest for the year, how much did she invest at each rate?
Note that this problem requires a chart to organize the information. The chart is based on the interest formula, which states that the amount invested times the rate of interest = interest earned. The chart is then used to set up the equation. How to solve simple interest word problems?
Example:
Suppose $7,000 is divided into two bank accounts. One account pays 10% simple interest per year and the other pays 5%. After three years there is a total of $1451.25 in interest between the two accounts. How much was invested into each account (rounded to the nearest cent)?
Dollar and Coin Word Problems
Example:
Paul has $31.15 from paper route collections. He has 5 more nickels than quarters and 7 fewer dimes than quarters. How many of each coin does Paul have?
Solution:
Let x be the number of quarters
x + 5 be the number of nickels
x – 7 be the number of dimes
25x + 5(x + 5) + 10(x – 7) = 3,115
25x + 5x + 25 + 10x – 70 = 3,115
40x = 3,160
x = 79
Algebra Money Word Problems
Algebra Money Word Problems with two variables (x and y). How to solve equations with two variables?Example:
David has only $5 bills and $10 bills in his wallet. If he has 5 bills totaling $35, how many of each does he have?
Coin Word Problem
Example:Martin has a total of 19 nickels and dimes worth $1.65. How many of each type of coin does he have? Note that this problem requires a chart to organize the information. The chart is based on the total value formula, which states that the number of coins times the value of each coin = the total value. The chart is then used to set up the equation.
How to solve word problems involving coins and money?
Example:
You have three times as many quarters as dimes and the total amount of money is $6.80. How many quarters and dimes do you have? How to solve algebra word problem involving money?
Example:
You have 6 times as many quarters as dimes and the total amount of money ia $8.00. How many quarters and dimes do you have? How to solve a coin word problem involving pennies and nickels?
Example:
A pile of 16 coins consists of pennies and nickels. The total amount of money is 36 cents. How many nickels and pennies do you have? How to solve a word problem involving stamps?
Example:
You bought 16 stamps consisting of 37-cent stamps and 23-cent stamps. If the total cost of the stamps is $4.10, find the number and types of stamps purchased.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.



We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.