Illustrative Mathematics Grade 8, Unit 1, Lesson 9: Moves in Parallel

Related Pages
Illustrative Math
Grade 7

Lesson 9: Moves in Parallel

Let’s transform some lines.

Illustrative Math Unit 8.1, Lesson 9 (printable worksheets)

Lesson 9 Summary

The following diagrams describe the effects of a rigid transformation on a pair of parallel lines and show that vertical angles are congruent.

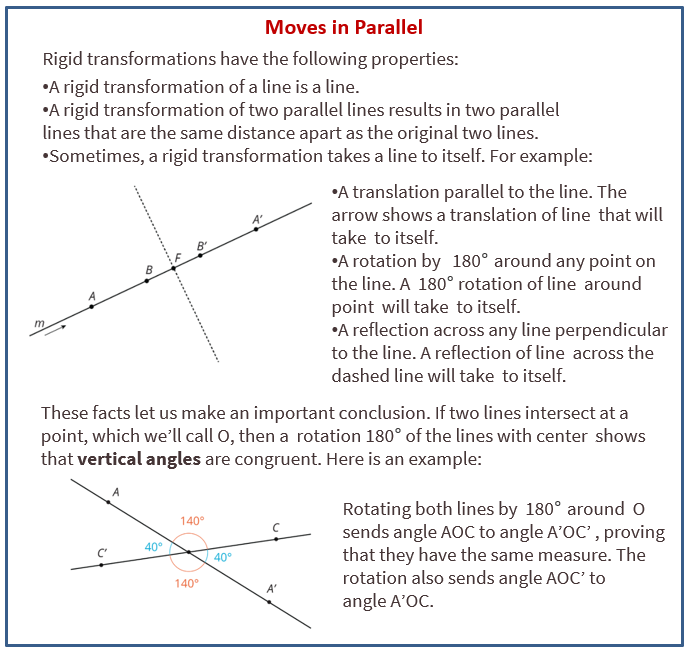
Lesson 9.1 Line Moves

For each diagram, describe a translation, rotation, or reflection that takes line l to line l'. Then plot and label A' and B', the images of A and B.

• See Video for Whole Lesson

Lesson 9.2 Parallel Lines

Use a piece of tracing paper to trace lines a and b and point K. Then use that tracing paper to draw the images of the lines under the three different transformations listed.
As you perform each transformation, think about the question:
What is the image of two parallel lines under a rigid transformation?

1. Translate lines a and b 3 units up and 2 units to the right.
   a. What do you notice about the changes that occur to lines a and b after the translation?
   b. What is the same in the original and the image?
2. Rotate lines a and b counterclockwise 180 degrees using K as the center of rotation.
   a. What do you notice about the changes that occur to lines a and b after the rotation?
   b. What is the same in the original and the image?
3. Reflect lines a and b across line h.
   a. What do you notice about the changes that occur to lines a and b after the reflection?
   b. What is the same in the original and the image?

Are you ready for more?

When you rotate two parallel lines, sometimes the two original lines intersect their images and form a quadrilateral. What is the most specific thing you can say about this quadrilateral? Can it be a square? A rhombus? A rectangle that isn’t a square? Explain your reasoning.

Lesson 9.3 Let’s Do Some 180’s

1. The diagram shows a line with points labeled A, C, D, and B.
   a. On the diagram, draw the image of the line and points A, C, and B after the line has been rotated 180 degrees around point D.
   b. Label the images of the points A', B', and C'.
   c. What is the order of all seven points? Explain or show your reasoning.
2. The diagram shows a line with points A and C on the line and a segment AD where D is not on the line.
   a. Rotate the figure 180 degrees about point C. Label the image of A as A' and the image of D as D'.
   b. What do you know about the relationship between angle CAD and angle CA’D'? Explain or show your reasoning.
3. The diagram shows two lines l and m that intersect at a point O with point A on l and point D on m.
   a. Rotate the figure 180 degrees around O. Label the image of A as A' and the image of D as D'.
   b. What do you know about the relationship between the angles in the figure? Explain or show your reasoning.

Lesson 9 Practice Problems

1. a. Draw parallel lines AB and CD.
   b. Pick any point E. Rotate AB 90 degrees clockwise around E.
   c. Rotate CD 90 degrees clockwise around E.
   d. What do you notice?
2. Use the diagram to find the measures of each angle. Explain your reasoning.
3. Points P and Q are plotted on a line.
   a. Find a point R so that a 180-degree rotation with center R sends P to Q and Q to P.
   b. Is there more than one point R that works for part a?
4. In the picture triangle A’B’C' is an image of triangle ABC after a rotation. The center of rotation is D.
   a. What is the length of side B’C'? Explain how you know.
   b. What is the measure of angle B? Explain how you know.
   c. What is the measure of angle C? Explain how you know.
5. The point (-4,1) is rotated 180 degrees counterclockwise using center (0,0). What are the coordinates of the image?

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.