Illustrative Mathematics Unit 6.7, Lesson 2: Points on the Number Line

Related Pages
Illustrative Math
Grade 6

Lesson 2: Points on the Number Line

Let’s plot positive and negative numbers on the number line.

Illustrative Math Unit 6.7, Lesson 2 (printable worksheets)

Lesson 2 Summary

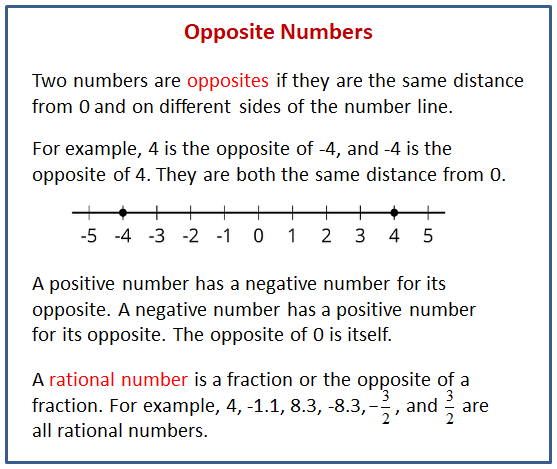
The following diagram shows what it means for numbers to be opposites.

Lesson 2.1 A Point on the Number Line

Which of the following numbers could be B?

• See Video for Whole Lesson

Scroll down the page for the solutions to the “Are you ready for more?” section.

Lesson 2.2 What’s the Temperature?

Here are five thermometers. The first four thermometers show temperatures in Celsius. Write the temperatures in the blanks.

1. The last thermometer is missing some numbers. Write them in the boxes.
2. Elena says that the thermometer shown here reads -2.5°C because the line of the liquid is above -2°C. Jada says that it is -1.5°C. Do you agree with either one of them? Explain your reasoning.
3. One morning, the temperature in Phoenix, Arizona was 8°C and the temperature in Portland, Maine was 12°C cooler. What was the temperature in Portland?

Lesson 2.3 Folded Number Lines

Your teacher will give you a sheet of tracing paper on which to draw a number line.

1. Follow the steps to make your own number line.

• Use a straightedge or a ruler to draw a horizontal line. Mark the middle point of the line and label it 0.
• To the right of 0, draw tick marks that are 1 centimeter apart. Label the tick marks 1, 2, 3. . . 10. This represents the positive side of your number line.
• Fold your paper so that a vertical crease goes through 0 and the two sides of the number line match up perfectly.
• Use the fold to help you trace the tick marks that you already drew onto the opposite side of the number line.
  Unfold and label the tick marks -1, -2, -3. . . -10. This represents the negative side of your number line.
  

2. Use your number line to answer these questions:
   a. Which number is the same distance away from zero as is the number 4?
   b. Which number is the same distance away from zero as is the number -7?
   c. Two numbers that are the same distance from zero on the number line are called opposites. Find another pair of opposites on the number line.
   d. Determine how far away the number 5 is from 0. Then, choose a positive number and a negative number that is each farther away from zero than is the number 5.
   e. Determine how far away the number -2 is from 0. Then, choose a positive number and a negative number that is each farther away from zero than is the number -2.
   Pause here so your teacher can review your work.
   
3. Here is a number line with some points labeled with letters. Determine the location of points P, X, and Y. If you get stuck, trace the number line and points onto a sheet of tracing paper, fold it so that a vertical crease goes through 0, and use the folded number line to help you find the unknown values.

Are you ready for more?

At noon, the temperatures in Portland, Maine and Phoenix, Arizona had opposite values. The temperature in Portland was 18°C lower than in Phoenix. What was the temperature in each city? Explain your reasoning.

• Show Answer

  18 ÷ 2 = 9.
  The temperature at Portland is -9°C and the temperature at Phoenix is 9°C.

Glossary Terms

opposite
Two numbers are opposites if they are the same distance from 0 and on different sides of the number line.
For example, 4 is the opposite of -4, and -4 is the opposite of 4. They are both the same distance from 0. One is negative, and the other is positive.

positive number
A rational number is a fraction or the opposite of a fraction.
For example, 8 and -8 are rational numbers because they can be written as 8/1 and -8/1.
Also, 0.75 and -0.75 are rational numbers because they can be written as 75/100 and -75/100.

Lesson 2 Practice Problems

1. For each number, name its opposite.
   a. -5
   b. 28
   c. -10.4
   d. 0.875
   e. 0
   f. -8,003
   
2. Plot the numbers -1.5, 3/2, -3/2, and -4/3 on the number line. Label each point with its numeric value.
   
3. Plot the following points on a number line.
   

• -1.5
• the opposite of -2
• the opposite of 0.5
• -2
  

4. a. Represent each of these temperatures in degrees Fahrenheit with a positive or negative number.
   i. 5 degrees above zero
   ii. 3 degrees below zero
   iii. 6 degrees above zero
   iv. 2 3/4 degrees below zero
   b. Order the temperatures above from the coldest to the warmest.
   
5. Solve each equation.
   a. 8x = 2/3
   b. 1 1/2 = 2x
   c. 5x = 2/7
   d. 1/4 x = 5
   e. 1/5 = 2/3 x
   
6. Write the solution to each equation as a fraction and as a decimal.
   a. 2x = 3
   b. 5y = 3
   c. 0.3z = 0.009
   
7. There are 15.24 centimeters in 6 inches.
   a. How many centimeters are in 1 foot?
   b. How many centimeters are in 1 yard?
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.