Illustrative Mathematics Grade 8, Unit 1, Lesson 16: Adding the Angles in a Triangle

Related Pages
Illustrative Math
Grade 8

Lesson 16: Parallel Lines and the Angles in a Triangle

Let’s see why the angles in a triangle add to 180 degrees.

Illustrative Math Unit 8.1, Lesson 16 (printable worksheets)

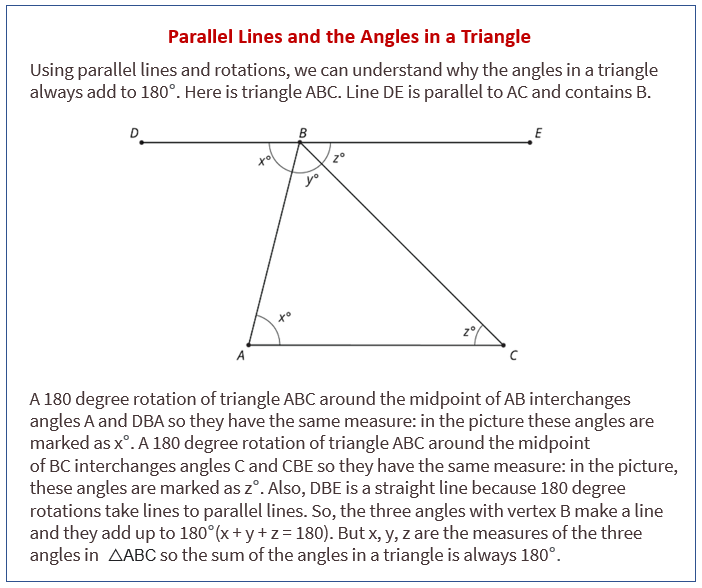
Lesson 16 Summary

The following diagram uses parallel lines to show that the sum of angles in a triangle is 180 degrees.

Lesson 16.1 True or False: Computational Relationships

Is each equation true or false?

• See Video for Whole Lesson

Lesson 16.2 Angle Plus Two

Here is triangle ABC.

1. Rotate triangle ABC 180° around the midpoint of side AC. Right click on the point and select Rename to label the new vertex D.
2. Rotate triangle ABC 180° around the midpoint of side AB. Right click on the point and select Rename to label the new vertex E.
3. Look at angles EAC, BAC, and CAD. Without measuring, write what you think is the sum of the measures of these angles. Explain or show your reasoning.
4. Is the measure of angle EAB equal to the measure of any angle in triangle ABC? If so, which one? If not, how do you know?
5. Is the measure of angle CAD equal to the measure of any angle in triangle ABC? If so, which one? If not, how do you know?
6. What is the sum of the measures of angles ABC, BAC, and ACB?

Lesson 16.3 Every Triangle in the World

Here is △ABC. Line DE is parallel to line AC.

1. What is m△DBA + b + m△CBE? Explain how you know.
2. Use your answer to explain why a + b + c = 180.
3. Explain why your argument will work for any triangle: that is, explain why the sum of the angle measures in any triangle is 180°.

Are you ready for more?

1. Using a ruler, create a few quadrilaterals. Use a protractor to measure the four angles inside the quadrilateral. What is the sum of these four angle measures?
2. Come up with an explanation for why anything you notice must be true (hint: draw one diagonal in each quadrilateral).

Lesson 16.4 Every Triangle in the World

This diagram shows a square BDFH that has been made by images of triangle ABC under rigid transformations.
Given that angle BAC measures 53 degrees, find as many other angle measures as you can.

Lesson 16 Practice Problems

1. For each triangle, find the measure of the missing angle.
2. Is there a triangle with two right angles? Explain your reasoning.
3. In this diagram, lines AB and CD are parallel.
   Angle ABC measures 35° and angle BAC measures 115°.
   a. What is m△ACE?
   b. What is m△DCB?
   c. What is m△ACB?
   
4. The two figures are congruent.
   a. Label the points A', B' and C' that correspond to A, B, and C in the figure on the right.
   b. If segment AB measures 2 cm, how long is segment A’B'? Explain.
   c. The point D is shown in addition to A and C. How can you find the point D' that corresponds to D? Explain your reasoning.

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.