Squares And Perfect Squares

Related Pages
More Lessons For Grade 8
Perfect Cubes
Math Worksheets

What Is The Square Of A Number?

The square of a number means to multiply the number by itself.

Example:
The square of 3 is 3 × 3 = 9

The square of a number can be written in exponent notation such as 3² where 3 is base and 2 is the exponent.

3² is read as “three to the second power” or “three squared”.

What Are Perfect Squares?

Perfect squares are the squares of whole numbers.

Example:
1, 4, 9, 16, 25 and 36 are the first 6 perfect squares because
1² = 1 × 1 = 1
2² = 2 × 2 = 4
3² = 3 × 3 = 9
4² = 4 × 4 = 16
5² = 5 × 5 = 25
6² = 6 × 6 = 36

How To Check For Perfect Squares?

We use repeated division by prime factors to check whether a given number is a perfect square.

Example:
Check whether 441 is a perfect square.

Solution:

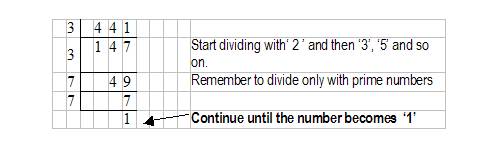
441 = 3 × 3 × 7 × 7
  = 3 × 7 × 3 × 7
  = 21 × 21
  = 21²

So, 441 is a perfect square.

How To Determine If A Number Is A Perfect Square?

We can use prime factorization to check if a number is a perfect square.

• Show Video Lesson

How To Find Smallest Positive Whole Number That Is A Perfect Square And A Multiple Of A Specific Whole Number?

Examples:

1. What is the smallest positive whole number that is a perfect square and is a multiple of 24?
2. What is the smallest positive whole number that is a perfect square and is a multiple of 150?

• Show Video Lesson

How To Find The Square Of Negative Numbers, Decimals And Fractions?

We can also have the square of negative numbers, decimals and fractions.

When calculating the square of a number, take note of the following:

1. The square of a number is always positive.
   Example:
   (−5)² = (−5) × (−5) = 25
   (−7)² = (−7) × (−7) = 49

Observe two important properties of a square in the last example above:
a) The square of a negative number becomes a positive number.
b) The square of a signed number is the same as the square of the unsigned number, i.e. (−7)² = 49 = 7².

2. The square of a decimal will have twice the number of decimal places as the original decimal.
   Example:
   (0.3)² = 0.3 × 0.3 = 0.09 ( 1 d.p. after squaring becomes 2 d.p.)
   (0.03)² = 0.03 × 0.03 = 0.0009 ( 2 d.p. after squaring become 4 d.p.)
   10.2² = 10.2 × 10.2 = 104.04 ( 1 d.p. after squaring becomes 2 d.p.)

3. To square a fraction, multiply the numerator by itself and do the same for the denominator.
   Example:
   
   
   

Take note that if a positive fraction which is less than 1 is squared, the result is always smaller than the original fraction.

4. To square a mixed number, change it to an improper fraction before calculating the square.
   Example:
   

Squaring Negative Numbers

Look for parentheses to group negative numbers that are to be squared.

Example:
Simplify (−3)² and −3²

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.