Prime Factorization Using Repeated Division
In these lessons, we will learn how to find prime factors using factor trees and repeated division.
Related Pages
Prime Factorization Using Factor Trees
Integers
Highest Common Factor
More Arithmetic Lessons
What Are Prime Factors
The prime factors of a number are factors which are prime numbers. We can find the prime factors of a number by repetitive division or stacked division. To find the prime factorization of the number, we need to find the prime factors that when multiplied together gives the number.
Example:
Find the prime factors of 36.
The following diagrams show Prime Factorization of a number using Factors Trees and using Repeated Division.
Scroll down the page for more examples and solutions of prime factorization.
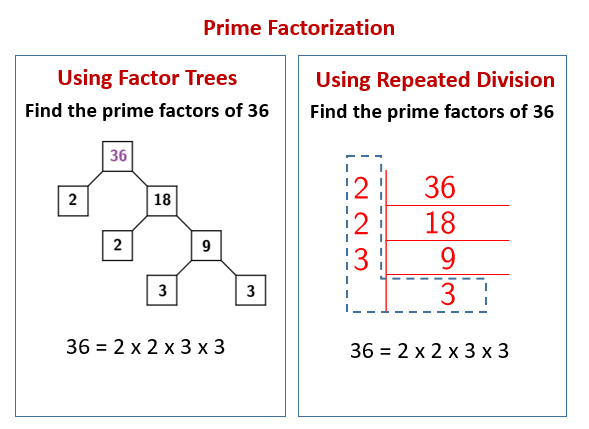
The prime factors of 36 are 2 and 3.
We can write 36 as a product of prime factors: 2 × 2 × 3 × 3
To find prime factors using the repetitive division, it is advisable to start with a small prime factor and continue the process with bigger prime factors.
Prime Factorization Using Stacked Division
Examples of how to use stacked division to find the prime factorization of a number rather than making a prime factorization tree.
Helpful Divisibility Rules
A number is divisible by 2 if it is even or ends in 0, 2, 4, 6, 8.
A number is divisible by 3 if the sum of the digits is divisible by 3.
A number is divisible by 5 if it ends in 0 or 5.
Example:
Use division to find the prime factorization.
a) 300
b) 693
Prime Factorization Using Stacked Division
Example:
Use division to find the prime factorization of 6,552.
Prime Factorization with Upside Down Division
Prime factors of a number can be found by using upside down division.
Prime Factorization with Upside Down Division
Example:
Find the prime factorization of the 210 by the division method.
Examples of prime factorization using repeated division
6, 24, 35, 51
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.