Illustrative Mathematics Grade 7, Unit 2, Lesson 2: Introducing Proportional Relationships with Tables

Related Pages Illustrative Math
Grade 7

Lesson 2: Introducing Proportional Relationships with Tables

Let’s solve problems involving proportional relationships using tables.

Illustrative Math Unit 7.2, Lesson 2 (printable worksheets)

Lesson 2 Summary

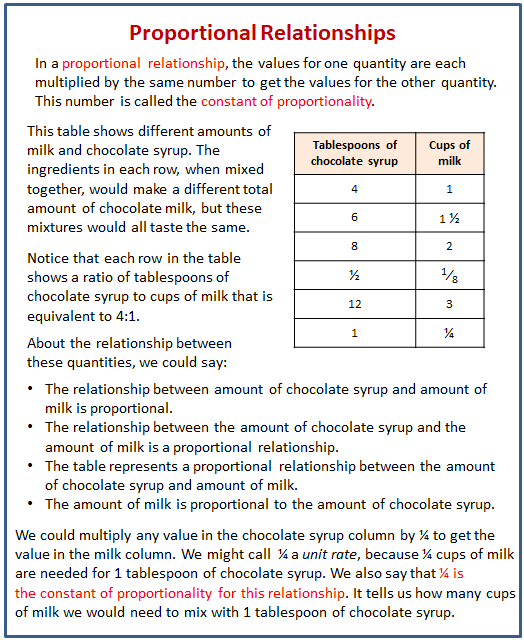
The following diagram shows how to use a table to reason about two quantities that are in a proportional relationship and understand the terms proportional relationship and constant of proportionality.

Lesson 2.1 Notice and Wonder: Paper Towels by the Case

Here is a table that shows how many rolls of paper towels a store receives when they order different numbers of cases.
What do you notice about the table? What do you wonder?

• See Video for Whole Lesson

Lesson 2.2 Feeding a Crowd

1. A recipe says that 2 cups of dry rice will serve 6 people. Complete the table as you answer the questions. Be prepared to explain your reasoning.
   a. How many people will 10 cups of rice serve?
   b. How many cups of rice are needed to serve 45 people?
   

Lesson 2.3 Making Bread Dough

A bakery uses 8 tablespoons of honey for every 10 cups of flour to make bread dough. Some days they bake bigger batches and some days they bake smaller batches, but they always use the same ratio of honey to flour. Complete the table as you answer the questions. Be prepared to explain your reasoning.

1. How many cups of flour do they use with 20 tablespoons of honey?
2. How many cups of flour do they use with 13 tablespoons of honey?
3. How many tablespoons of honey do they use with 20 cups of flour?
4. What is the proportional relationship represented by this table?
   

Lesson 2.4 Quarters and Dimes

4 quarters are equal in value to 10 dimes.

1. How many dimes equal the value of 6 quarters?
2. How many dimes equal the value of 14 quarters?
3. What value belongs next to the 1 in the table? What does it mean in this context?

Are you ready for more?

Pennies made before 1982 are 95% copper and weigh about 3.11 grams each. (Pennies made after that date are primarily made of zinc). Some people claim that the value of the copper in one of these pennies is greater than the face value of the penny. Find out how much copper is worth right now, and decide if this claim is true.

• Show Answers

  If we take the copper price to be $3.5170 per pound.
  95% · 3.11 g = 2.9545 g = 0.0065136 pounds
  The copper in the penny is now worth $3.5170 · 0.0065136 = $0.022908 which is more than than the face value of $0.01

Glossary Terms

constant of proportionality
In a proportional relationship, the values for one quantity are each multiplied by the same number to get the values for the other quantity. This number is called the constant of proportionality.
In this example, the constant of proportionality is 3, because 2 · 3 = 6, 3 · 3 = 9, and 5 · 3 = 15. This means that there are 3 apples for every 1 orange in the fruit salad.

proportional relationship
In a proportional relationship, the values for one quantity are each multiplied by the same number to get the values for the other quantity.
For example, in this table every value of p is equal to 4 times the value of s on the same row.
We can write this relationship as p = 4s. This equation shows that p is proportional to s.

Lesson 2 Practice Problems

1. When Han makes chocolate milk, he mixes 2 cups of milk with 3 tablespoons of chocolate syrup. Here is a table that shows how to make batches of different sizes.
   Use the information in the table to complete the statements. Some terms are used more than once.
   a. The table shows a proportional relationship between ______________ and ______________.
   b. The scale factor shown is ______________.
   c. The constant of proportionality for this relationship is ______________.
   d. The units for the constant of proportionality are ______________ per ______________.
   Bank of Terms: tablespoons of chocolate syrup, 4, cups of milk, cup of milk, 3/2
   
2. A certain shade of pink is created by adding 3 cups of red paint to 7 cups of white paint.
   a. How many cups of red paint should be added to 1 cup of white paint?
   b. What is the constant of proportionality?
   
3. A map of a rectangular park has a length of 4 inches and a width of 6 inches. It uses a scale of 1 inch for every 30 miles.
   a. What is the actual area of the park? Show how you know.
   b. The map needs to be reproduced at a different scale so that it has an area of 6 square inches and can fit in a brochure. At what scale should the map be reproduced so that it fits on the brochure? Show your reasoning.
   
4. Noah drew a scaled copy of Polygon P and labeled it Polygon Q.
   If the area of Polygon P is 5 square units, what scale factor did Noah apply to Polygon P to create Polygon Q? Explain or show how you know.
   
5. Select all the ratios that are equivalent to each other.
   A. 4 : 7
   B. 8 : 15
   C. 16 : 28
   D. 2 : 3
   E. 20 : 35
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.