Direct & Inverse Proportions/Variations
Related Pages:
Direct Variations
Proportion Word Problems
More Algebra Lessons
In these lessons, we will learn how to solve direct proportions (variations) and inverse proportions (inverse variations) problems. (Note: Some texts may refer to inverse proportions/variations as indirect proportions/variations.)
Direct Proportional Word Problems
The following diagram gives the steps to solve ratios and direct proportion word problems.
Scroll down the page for examples and step-by-step solutions.
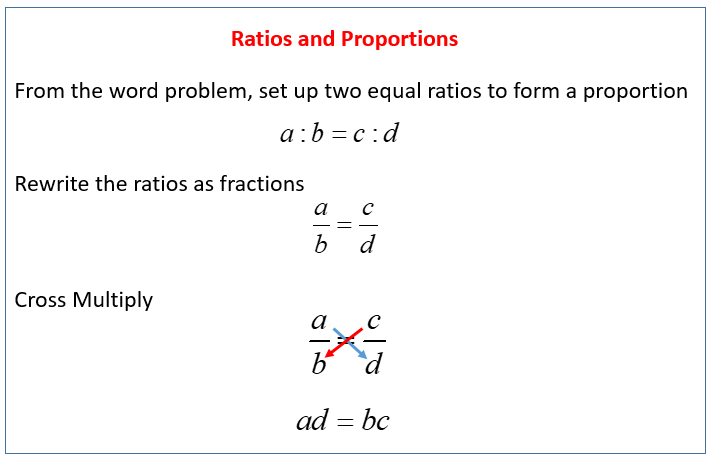
Proportion Word Problems
Direct Proportion Problems
Inverse Proportion Problems
Proportion Word Problems
Ratio & Proportion Problems
Ratio & Proportion Worksheets
Practice your skills with the following worksheets:
Printable & Online Ratio & Proportion Worksheets
Two quantities are in direct proportion if they increase or decrease together at the same rate. This means that their ratio remains constant.
If quantity A is directly proportional to quantity B, then as A doubles, B doubles; as A is halved, B is halved.
How to Identify a Direct Proportional Problem:
Look for phrases like “is directly proportional to,” “varies directly as,” “increases with,” or “decreases with."
Ask yourself: “If I increase the first quantity, does the second quantity also increase?"
Mathematical Relationship:
\( y\propto x\)
\(y=kx\) (where \(k\) is the constant of proportionality)
\(\frac{y}{x}=k\)
For two pairs of values: \(\frac{y_{1}}{x_{1}}=\frac{y_{2}}{x_{2}}\) (This is the proportion you’ll set up)
How to Solve a Direct Proportion Word Problem:
- Set up two ratios comparing the same quantities in the same order.
- Form a proportion by setting the two ratios equal to each other.
- Use cross-multiplication to solve for the unknown variable.
Direct Proportions/Variations
Two values x and y are directly proportional
to each other when the ratio x : y or
is a constant (i.e. always remains the same). This would mean that x and y will either increase together
or decrease together by an amount that would not change the ratio.
Knowing that the ratio does not change allows you to form an equation to find the value of an unknown variable.
Example:
If two pencils cost $1.50, how many pencils can you buy with $9.00?
Solution:
The number of pencils is directly proportional to the cost.
pencils.
How To Solve Directly Proportional Questions?
Example 1: F is directly proportional to x. When F is 6, x is 4. Find the value of F when x is 5.
Example 2: A is directly proportional to the square of B. When A is 10, B is 2. Find the value of A when B is 3.
How To Use Direct Proportion?
How To Solve Word Problems Using Proportions?
This video shows how to solve word problems by writing a proportion and solving
1. A recipe uses 5 cups of flour for every 2 cups of sugar. If I want to make a recipe using 8 cups of flour,
how much sugar do I use?
2. A syrup is made by dissolving 2 cups of sugar in 2/3 cups of boiling water. How many cups of sugar should
be used for 2 cups of boiling water?
3. A school buys 8 gallons of juice for 100 kids. how many gallons do they need for 175 kids?
Solving More Word Problems Using Proportions
1. On a map, two cities are 2 5/8 inches apart. If 3/8 inches on the map represents 25 miles, how far apart
are the cities (in miles)?
2. Solve for the sides of similar triangles using proportions
Inverse Proportions/Variations Or Indirect Proportions
Two values x and y are inversely proportional to each other when their product xy is a constant (always remains the same). This means that when x increases y will decrease, and vice versa, by an amount such that xy remains the same.
Knowing that the product does not change also allows you to form an equation to find the value of an unknown variable.
How to Identify an Inversely Proportional Problem:
Look for phrases like “is inversely proportional to,” “varies inversely as,” “the more… the less…"
Ask yourself: “If I increase the first quantity, does the second quantity decrease?”
Mathematical Relationship:
\( y\propto \frac{1}{x}\)
\(y=\frac{k}{x}\) (where \(k\) is the constant of proportionality)
\(xy=k\)
For two pairs of values: \(x_1y_1=x_2y_2) (This is the equation you’ll set up)
How to Solve an Inversely Proportional Problem:
- Identify the two pairs of quantities.
- Set their products equal to each other.
- Solve for the unknown variable.
Example:
It takes 4 men 6 hours to repair a road. How long will it take 8 men to do the job if they work at the same rate?
Solution:
The number of men is inversely proportional to the time taken to do the job.
Let t be the time taken for the 8 men to finish the job.
4 × 6 = 8 × t
24 = 8t
t = 3 hours
Usually, you will be able to decide from the question whether the values are directly proportional or inversely proportional.
How To Solve Inverse Proportion Questions?
This video shows how to solve inverse proportion questions. It goes through a couple of examples and ends with
some practice questions
Example 1: A is inversely proportional to B. When A is 10, B is 2. Find the value of A when B is 8
Example 2: F is inversely proportional to the square of x. When A is 20, B is 3. Find the value of F when x is 5.
How To Use Inverse Proportion To Work Out Problems?
How to use a more advanced form of inverse proportion where the use of square numbers is involved.
More examples to explain direct proportions / variations and inverse proportions / variations
How to solve Inverse Proportion Math Problems on pressure and volume?
In math, an inverse proportion is when an increase in one quantity results in a decrease in another quantity.
This video will show how to solve an inverse proportion math problem.
Example: The pressure in a piston is 2.0 atm at 25°C and the volume is 4.0L. If the pressure is increased to 6.0 atm at the same temperature, what will be the volume?
Check out many other Algebra Word Problems
Age Word Problems, Average Word Problems, Coin Word Problems, Consecutive Integer Word Problems, Digit Word Problems, Distance Word Problems, Fraction Word Problems, Geometry Word Problems, Integer Word Problems, Interest Word Problems, Lever Word Problems, Mixture Word Problems, Money Word Problems, Motion & Distance Word Problems, Number Sequence Word Problems, Proportion Word Problems, Quadratic Equation Word Problems, Ratio Word Problems, Symbol Word Problems, Variation Word Problems, Work Word Problems.
Try out our new and fun Fraction Concoction Game.
Add and subtract fractions to make exciting fraction concoctions following a recipe. There are four levels of difficulty: Easy, medium, hard and insane. Practice the basics of fraction addition and subtraction or challenge yourself with the insane level.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.