Geometry: Pythagorean Theorem

Related Pages
Pythagorean Triples
Converse Of Pythagorean Theorem
Pythagorean Theorem Word Problems
Applications Of Pythagorean Theorem
More Geometry Lessons

The following diagram gives the formula for the Pythagorean Theorem, scroll down the page for more examples and solutions that use the Pythagorean Theorem.

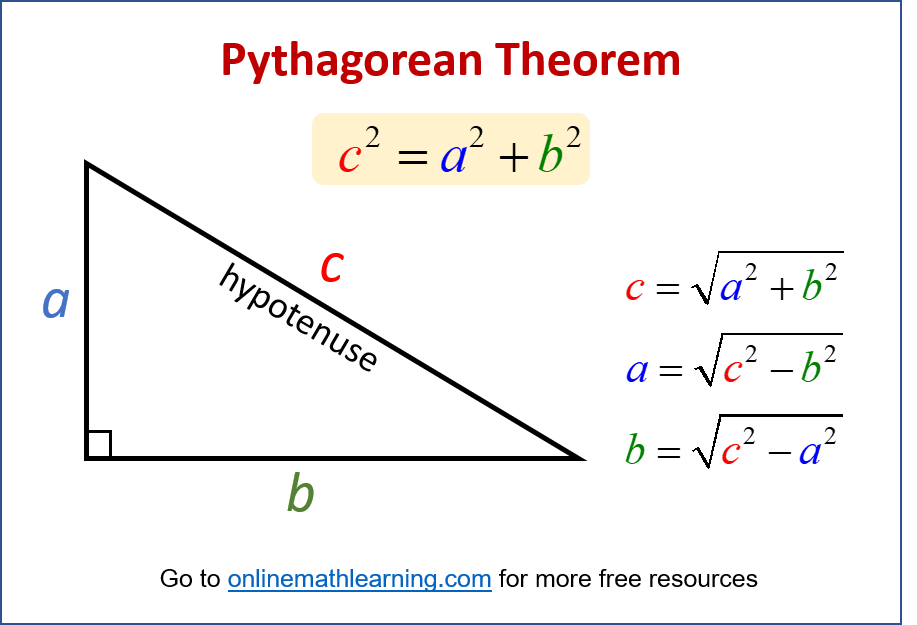
What Is The Pythagorean Theorem?

A right triangle consists of two sides called the legs and one side called the hypotenuse. The hypotenuse is the longest side and is opposite the right angle.

The Pythagorean Theorem or Pythagoras' Theorem is a formula relating the lengths of the three sides of a right triangle.

If we take the length of the hypotenuse to be c and the length of the legs to be a and b then this theorem tells us that:

c² = a² + b²

Pythagorean Theorem states that

In any right triangle, the sum of the squared lengths of the two legs is equal to the squared length of the hypotenuse.

Note: Pythagorean theorem only works for right triangles.

Proof Of The Pythagorean Theorem Using Similar Triangles

This proof is based on the proportionality of the sides of two similar triangles, that is, the ratio of any corresponding sides of similar triangles is the same regardless of the size of the triangles.

Examples:

1. Determine the length of the missing side of the right triangle.
2. Mason wants to lay pavers in his families' backyard for the summer. It is important that he start the pavers at a right angle. If he want the dimensions of the patio to be 8 ft by 10 ft, what should the diagonal measure?

• Show Video Lesson

What Is The Converse Of The Pythagorean Theorem?

The converse of the Pythagorean Theorem is also true.

For any triangle with sides a, b, and c, if a² + b² = c², then the angle between a and b measures 90° and the triangle is a right triangle.

How To Use The Converse Of The Pythagorean Theorem?

We can use the converse of the Pythagorean Theorem to check whether a given triangle is an acute triangle, a right triangle or an obtuse triangle.

For a triangle with sides a, b and c and c is the longest side then: If c² < a² + b² then it is an acute-angled triangle, i.e. the angle facing side c is an acute angle.

If c² = a² + b² then it is a right-angled triangle, i.e. the angle facing side c is a right angle.

If c² > a² + b² then it is an obtuse-angled triangle, i.e. the angle facing side c is an obtuse angle.

Pythagorean Theorem Worksheets
Pythagorean Theorem (Find the missing side)
Pythagorean Theorem (Test for right triangle)
Pythagorean Theorem (Dynamically generated)
Pythagorean Theorem (Word Problems)
Factoring Quadratic Equations
Converse Pythagorean Theorem: Types of Triangles

How to use the Converse of the Pythagorean Theorem?

This video shows how to use the Pythagorean Theorem and its Converse to determine if a triangle is acute, right, or obtuse.

According to the triangle inequality theorem, the sum of the two shorter sides of a triangle must be greater that the longest side.

Examples: Determine if the lengths represent the sides of an acute, right, or obtuse triangle if a triangle is possible.

1. 7, 24, 25
2. 5, 12, 16
3. 6, 8, 9
4. 3, 5, 9

• Show Video Lesson

How to use the Pythagorean Theorem?

The Pythagorean Theorem can be used when we know the length of two sides of a right triangle and we need to get the length of the third side.

Example 1:
Find the length of the hypotenuse of a right triangle if the lengths of the other two sides are 3 inches and 4 inches.

Solution:
Step 1: Write down the formula
c² = a² + b²

Step 2: Plug in the values
c² = 3² + 4²
c² = 9 + 16<
c² = 25
c = √25
c = 5

Answer: The length of the hypotenuse is 5 inches.

Example 2:
Find the length of one side of a right triangle if the length of the hypotenuse is 10 inches and the length of the other side is 9 inches.

Solution:
Step 1: Write down the formula
c² = a² + b²

Step 2: Plug in the values
10² = 9² + b²
100 = 81 + b²
Step 3: Subtract 81 from both sides
19 = b²
b = √19
b ≈ 4.36

Answer: The length of the side is 4.36 inches.

How to use the Pythagorean Theorem to solve real-world problems?

Examples:

1. Claire wants to hang a banner from the sill of a second-story window in her house. She needs to find a ladder that, when rested against the outside wall of her house will be long enough to reach the second-story window. If the window is 16 feet above the ground and Claire places the foot of the ladder 12 feet from the wall, how long will the ladder need to be?
2. During a baseball game, the second baseman gets the ball and throws it to the catcher to stop a runner before he gets to home. If it is 90 feet between each base, how far did the second baseman throw the ball?
3. A water park wants to add a zipline into a pool. If the platform at the top of the zipline is 25 feet tall, and the pool is 40 feet ling, what is the maximum length needed for the zipline?
4. A wheelchair ramp is needed at the entrance to a building. There is only 10 feet of space available for the ramp. How long should the ramp be?
5. A television screen is advertised as 50 inches. If the television is 35 inches wide, how tall is it?
6. A kite at the end of a 40 feet line is 10 feet behind the runner. How high is the kite?
7. A roof is being placed on a frame that is 9 feet tall and 30 feet wide. How long are the diagonal pieces of the frame?

• Show Video Lesson

Proofs of the Pythagorean Theorem

There are many ways to proof the Pythagorean Theorem. We will look at three of them here.

How to proof the Pythagorean Theorem using Similar Triangles?

This proof is based on the fact that the ratio of any two corresponding sides of similar triangles is the same regardless of the size of the triangles.

Given Triangle ABC drawn above in the image and prove a² + b² = c² using Similar Triangles.

Triangle ABC ∼ Triangle ACD AA (Similarity Postulate)

Triangle ABC ∼ Triangle CBD AA (Similarity Postulate)

c/a = a/x (Converse of SSS Similarity Postulate)

c/b = b/y (Converse of SSS Similarity Postulate)

a² = cx (Cross multiply)

b² = cy (Cross multiply)

a² + b² = cx + cy (Adding the equations)

a² + b² = c(x + y) (GCF)

a² + b² = c² (Substitution)

• Show Video Lesson

How to proof the Pythagorean Theorem using Algebra?

In this proof, we use four copies of the right triangle, rearrange them and use algebra to proof the theorem.

• Show Video Lesson

How to proof of the Pythagorean Theorem using Rearrangement of shapes?

The following video shows how a square with area c² can be cut up and rearranged such that it can fit into two other smaller squares with areas a² and b².

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.