Illustrative Mathematics Unit 6.2, Lesson 2: Representing Ratios with Diagrams

Related Topics:
Math Worksheets

Learn more about ratios and how to represent them with diagrams. After trying the questions, click on the buttons to view answers and explanations in text or video.

Return to the list of Illustrative Math lessons

Representing Ratios with Diagrams
Let’s use diagrams to represent ratios.

Illustrative Math Unit 6.2, Lesson 2 (printable worksheets)

2.1 - Number Talk: Dividing by 4 and Multiplying by ¼

Find the value of each expression mentally.

24 ÷ 4
¼ · 24
24 · ¼
5 ÷ 4

What do you notice?

• Answers

  24 ÷ 4 = 6
  ¼ · 24 = 6
  24 · ¼ = 6
  5 ÷ 4 = ⁵⁄₄

  From the first three equations, we can see that dividing by 4 is the same as multiplying by ¼. Dividing by a number is the same as multiplying by its reciprocal.

• What is a Reciprocal?

  The reciprocal of a fraction is a fraction obtained by switching the values in the numerator and the denominator of the given fraction.

  Finding the reciprocal of a whole number uses the same process. The whole number 4 is equal to ⁴⁄₁.

• See Video 1 for Whole Lesson
• See Video 2 for Whole Lesson

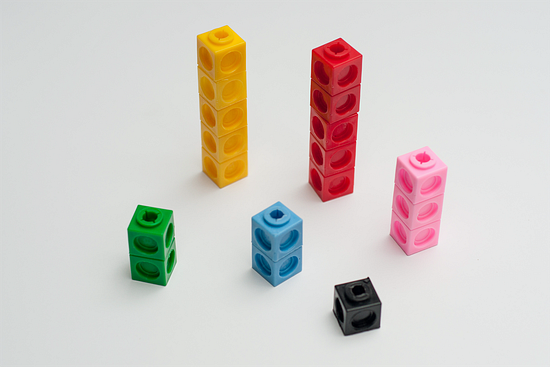
2.2 - A Collection of Snap Cubes

Here is a collection of snap cubes.

• Show Collection
  

1. Choose two of the colors in the image, and draw a diagram showing the number of snap cubes for these two colors.

2. Trade papers with a partner. On their paper, write a sentence to describe a ratio shown in their diagram. Your partner will do the same for your diagram.

3. Return your partner’s paper. Read the sentence written on your paper. If you disagree, explain your thinking.

• See Possible Answers

  1.
  

  2. There are 2 green snap cubes for every 1 black snap cube.

• Notes

  Ratios where all the numbers are the same are also valid. For example, the ratio of green to blue cubes is 2:2.

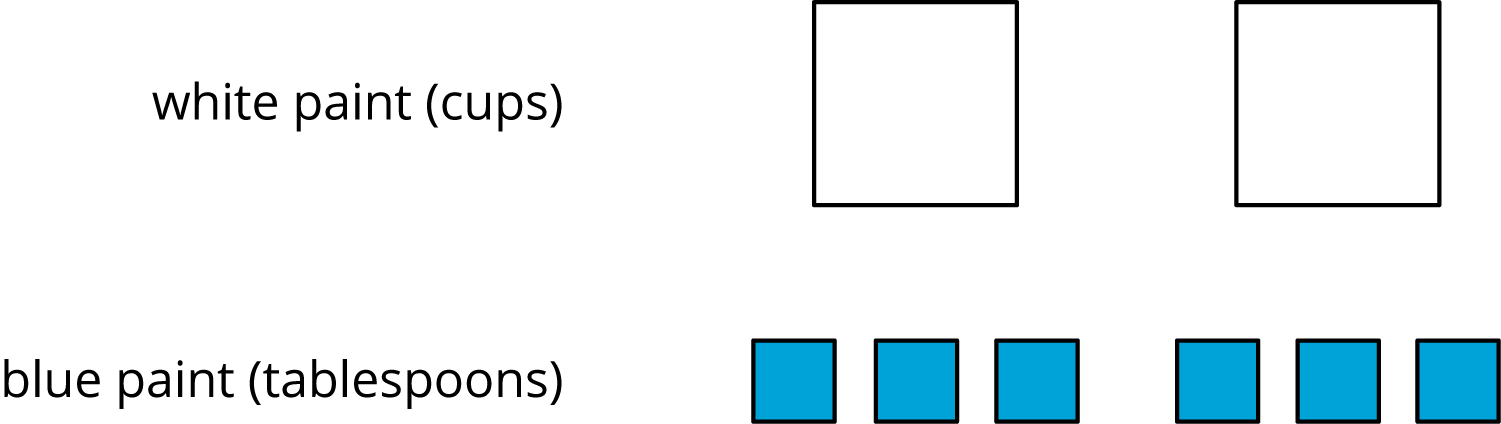
2.3 - Blue Paint and Art Paste

Elena mixed 2 cups of white paint with 6 tablespoons of blue paint. Here is a diagram that represents this situation.

• Show diagram
  

1. Discuss the statements that follow, and circle all those that correctly describe this situation. Make sure that both you and your partner agree with each circled answer.

1. The ratio of cups of white paint to tablespoons of blue paint is 2:6.
2. For every cup of white paint, there are 2 tablespoons of blue paint.
3. There is 1 cup of white paint for every 3 tablespoons of blue paint.
4. There are 3 tablespoons of blue paint for every cup of white paint.
5. For each tablespoon of blue paint, there are 3 cups of white paint.
6. For every 6 tablespoons of blue paint, there are 2 cups of white paint.
7. The ratio of tablespoons of blue paint to cups of white paint is 6 to 2.

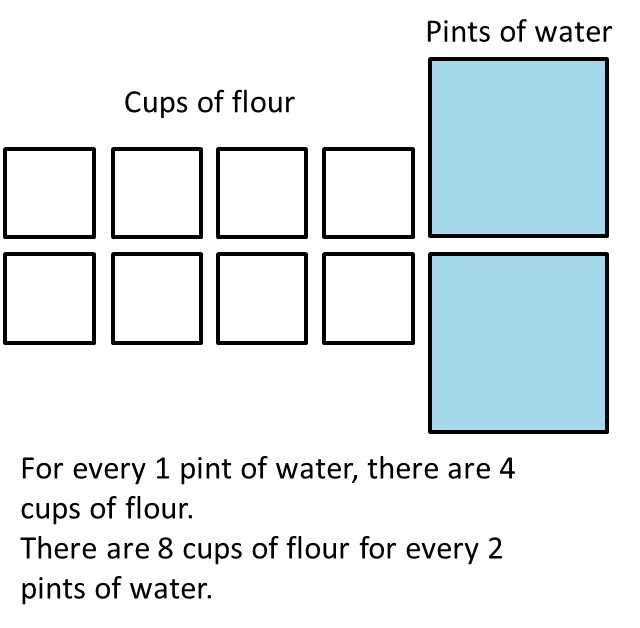
2. Jada mixed 8 cups of flour with 2 pints of water to make paste for an art project.

1. Draw a diagram that represents the situation.
2. Write at least two sentences describing the ratio of flour and water.

• See Possible Answers

  1.
  

  2. For every 1 pint of water, there are 4 cups of flour. (This ratio is equivalent to the second sentence; equivalent ratios will be explored further in later lessons.)
  There are 8 cups of flour for every 2 pints of water.

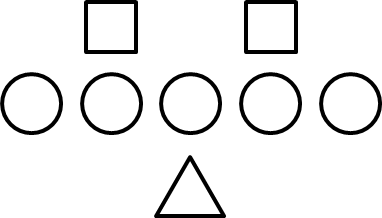
2.4 - Card Sort: Spaghetti Sauce

Your teacher will give you cards describing different recipes for spaghetti sauce. In the diagrams:

A circle represents a cup of tomato sauce.
A square represents a tablespoon of oil.
A triangle represents a teaspoon of oregano.

One diagram is included here as an example:

• Show recipe
  

1. Take turns with your partner to match a sentence with a diagram.

1. For each match that you find, explain to your partner how you know it’s a match.
2. For each match that your partner finds, listen carefully to their explanation. If you disagree, discuss your thinking and work to reach an agreement.

2. After you and your partner have agreed on all of the matches, check your answers with the answer key. If there are any errors, discuss why and revise your matches.

3. There were two diagrams that each matched with two different sentences. Which were they?

4. Select one of the other diagrams and invent another sentence that could describe the ratio shown in the diagram.

• See Possible Answers

  In the example diagram, for every 1 tablespoon of oil, there are 3 cups of tomato sauce.

Create a diagram that represents any of the ratios in a recipe of your choice. Is it possible to include more than 2 ingredients in your diagram?

• See Possible Answers

  

  It is possible to include more than 2 ingredients in the diagram, and to describe relationships between them in the same ratio.

  In this diagram, the ratio of cups of tomato sauce to tablespoons of oil to teaspoons of oregano is 5:2:1.

Lesson 2 Summary

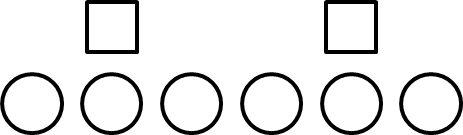
Ratios can be represented using diagrams. The diagrams do not need to include realistic details. For example, a recipe for lemonade says, "Mix 2 scoops of lemonade powder with 6 cups of water."

We can draw something like this:

• Show diagram
  

This diagram shows that the ratio of cups of water to scoops of lemonade powder is 6 to 2. We can also see that for every scoop of lemonade powder, there are 3 cups of water.

Practice Problems

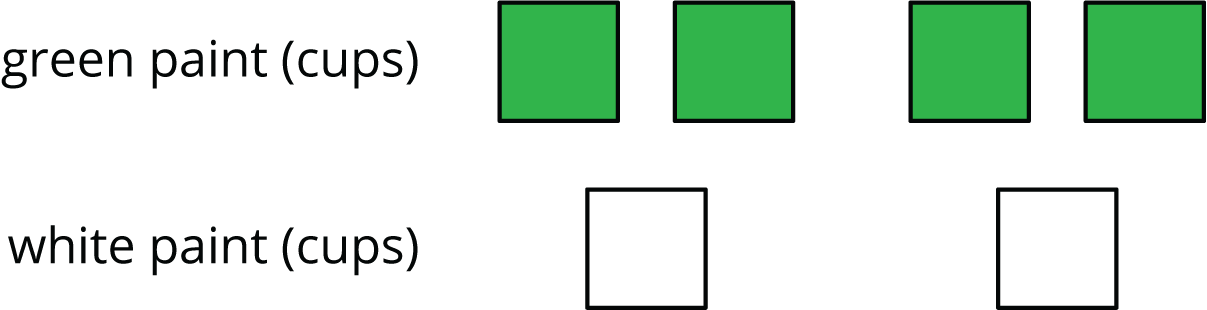
1. Here is a diagram that describes the cups of green and white paint in a mixture.

• Show Diagram
  

Select all the statements that accurately describe this diagram.

1. The ratio of cups of white paint to cups of green paint is 2 to 4.
2. For every cup of green paint, there are two cups of white paint.
3. The ratio of cups of green paint to cups of white paint is 4:2.
4. For every cup of white paint, there are two cups of green paint.
5. The ratio of cups of green paint to cups of white paint is 2:4.

• Answers

  A, C, and D are true. B and E are false.

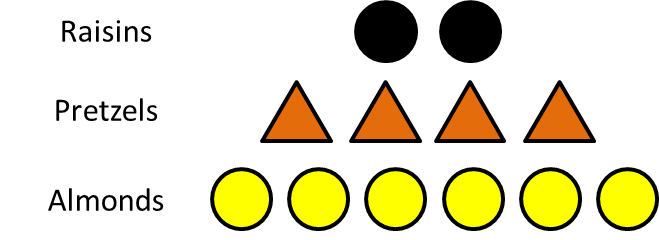
2. To make a snack mix, combine 2 cups of raisins with 4 cups of pretzels and 6 cups of almonds.

a. Create a diagram to represent the quantities of each ingredient in this recipe.

b. Use your diagram to complete each sentence.
The ratio of __________________ to __________________ to __________________ is ________ : ________ : ________.
There are ________ cups of pretzels for every cup of raisins.
There are ________ cups of almonds for every cup of raisins.

• See Possible Answers

  a.
  

  b. The ratio of raisins to pretzels to almonds is 2 : 4 : 6.
  There are 2 cups of pretzels for every cup of raisins.
  There are 3 cups of almonds for every cup of raisins.

3. a. A square is 3 inches by 3 inches. What is its area?

b. A square has a side length of 5 feet. What is its area?

c. The area of a square is 36 square centimeters. What is the length of each side of the square?

• Answers

  a. 3² in² = 9 in²

  b. 5² ft² = 25 ft²

  c. √36 = 6
  (This means that 6² = 36.)
  Hence, the sides of the square are 6 cm each.

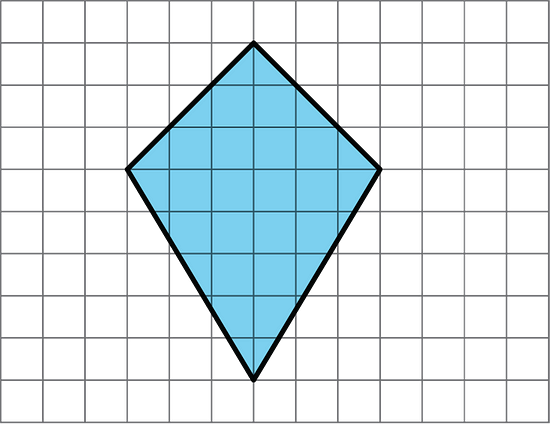
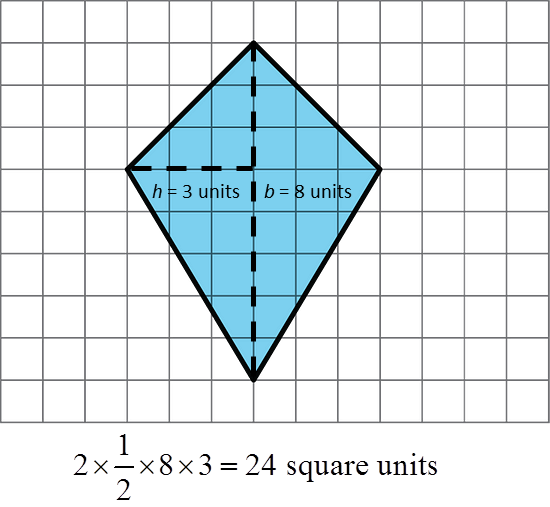
4. Find the area of this quadrilateral. Explain or show your strategy.

• Show Quadrilateral
  

• See Possible Answers

  

  This quadrilateral is a rhombus, which can be divided into 2 congruent triangles.
  The total area of the 2 triangles, and hence the area of the rhombus, is 2 × ½ × 3 × 8 = 24 square units.

5. Complete each equation with a number that makes it true.

a. ¹⁄₈ · 8 = _____
b. ³⁄₈ · 8 = _____
c. ¹⁄₈ · 7 = _____
d. ³⁄₈ · 7 = _____

• Answers

  a. ¹⁄₈ · 8 = 1
  b. ³⁄₈ · 8 = 3
  c. ¹⁄₈ · 7 = ⁷⁄₈
  d. ³⁄₈ · 7 = ²¹⁄₈ = 2⁵⁄₈

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.