Ratio Word Problems - Two Terms Ratio
Related Pages
More Ratio Problems
Math Word Problems
Algebra Word Problems
Math Worksheets
In these lessons, we will learn how to solve ratio word problems involving 2-term ratios. We can use the cross-multiplication method for finding an unknown value when two ratios are equal.
Understanding Cross-Multiplication
A ratio compares two quantities. A proportion states that two ratios are equal. When you have a proportion in the form:
a/b = c/d
Cross-multiplication involves multiplying the numerator of the first fraction (a) by the denominator of the second fraction (d), and setting it equal to the product of the denominator of the first fraction (b) and the numerator of the second fraction (c):
a × d = b × c
This allows you to solve for an unknown variable.
Key Tips for Ratio Problems:
- Set up the proportion correctly: Make sure corresponding quantities are in the same positions in the fractions.
- Use units: Include units in your proportion to avoid errors.
- Cross-multiply: This is the standard way to solve proportions.
- Solve for the unknown: Use algebra to isolate the variable.
- Check your answer: Does your answer make sense in the context of the problem?
The following diagram gives the steps to solve a part-to-total ratio word problem using cross-multiplication. Scroll down the page for examples and step-by-step solutions.
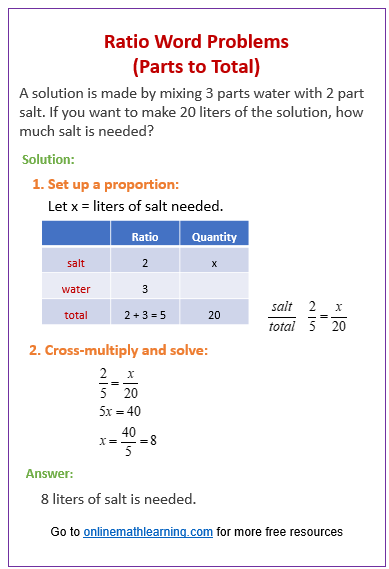
The following diagram gives the steps to solve a part-to-total ratio word problem using the multiplier method. Scroll down the page for examples and step-by-step solutions.

Ratio Word Problems
Ratio Problems
Two-Term Ratios
Three-Term Ratios
Ratio Word Problems
Ratio problems: Two-term Ratios
Example 1:
In a bag of red and green sweets, the ratio of red sweets to green sweets is 3:4. If the
bag contains 120 green sweets, how many red sweets are there?
Solution:
Step 1: Assign variables:
Let x= red sweets
Write the items in the ratio as a fraction.
Step 2: Solve the equation
3 × 120 = 4 × x
360 = 4x
Isolate variable x
Answer: There are 90 red sweets.
Example 2:
John has 30 marbles, 18 of which are red and 12 of which are blue. Jane has 20 marbles, all of
them either red or blue. If the ratio of the red marbles to the blue marbles is the same for
both John and Jane, then John has how many more blue marbles than Jane?
Solution:
Step 1: Sentence: Jane has 20 marbles, all of them either red or blue.
Assign variables:
Let x = blue marbles for Jane
20 – x = red marbles for Jane
We get the ratio from John
John has 30 marbles, 18 of which are red and 12 of which are blue.
We use the same ratio for Jane.
Step 2: Solve the equation
3 × x = 2 × (20 – x)
3x = 40 – 2x
Isolate variable x
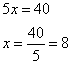
John has 12 blue marbles. So, he has 12 – 8 = 4 more blue marbles than Jane.
Answer: John has 4 more blue marbles than Jane.
Ratios and solving ratio word problems
Examples:
- In a class there are 10 males and 15 females. What is the ratio of males to females?
- A classroom has a total of 30 students. There are 20 males. What is ratio of males to females?
- In a class the ratio of males to females is 4:5. If there are 36 total students, how many females are in the class?
How to use proportions to solve ratio word problems?
Examples:
- The ratio of salamanders to frogs was 5 to 7. If there were 20 salamanders, how many frogs were there?
- If 3 sacks of concrete will make 12 square feet of sidewalk, predict how many sacks of concrete are needed to make 40 square feet of sidewalk.
Check out many other Algebra Word Problems
Age Word Problems, Average Word Problems, Coin Word Problems, Consecutive Integer Word Problems, Digit Word Problems, Distance Word Problems, Fraction Word Problems, Geometry Word Problems, Integer Word Problems, Interest Word Problems, Lever Word Problems, Mixture Word Problems, Money Word Problems, Motion & Distance Word Problems, Number Sequence Word Problems, Proportion Word Problems, Quadratic Equation Word Problems, Ratio Word Problems, Symbol Word Problems, Variation Word Problems, Work Word Problems.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.