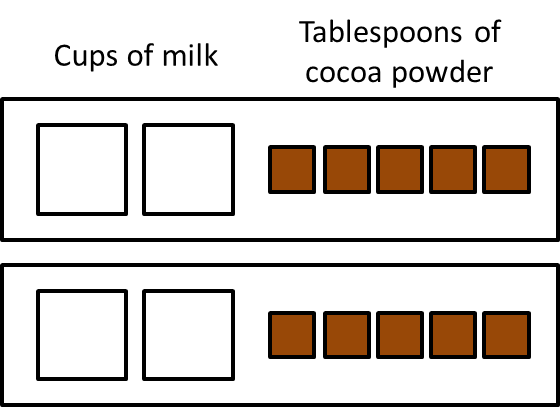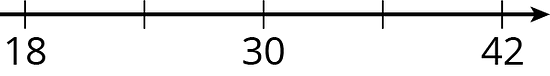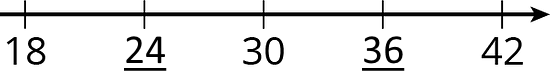Illustrative Mathematics Unit 6.2, Lesson 3: Recipes

Related Topics:
Math Worksheets

Learn more about recipes as a real-life application of ratios, and about how ratios are represented. After trying the questions, click on the buttons to view answers and explanations in text or video.

Return to the list of Illustrative Math lessons

Recipes
Let’s explore how ratios affect the way a recipe tastes.

Illustrative Math Unit 6.2, Lesson 3 (printable worksheets)

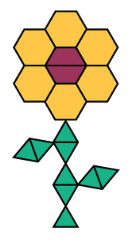
3.1 - Flower Pattern

This flower is made up of yellow hexagons, red trapezoids, and green triangles.

• Show Pattern
  

1. Write sentences to describe the ratios of the shapes that make up this pattern.

2. How many of each shape would be in two copies of this flower pattern?

• See Possible Answers

  1. There are many different sentences that can be written to describe the ratios of the shapes. For example, the ratio of hexagons to trapezoids to triangles is 6:2:9.

  2. There would be 6 × 2 = 12 hexagons, 2 × 2 = 4 trapezoids, and 9 × 2 = 18 triangles.

• See Video 1 for Whole Lesson
• See Video 2 for Whole Lesson

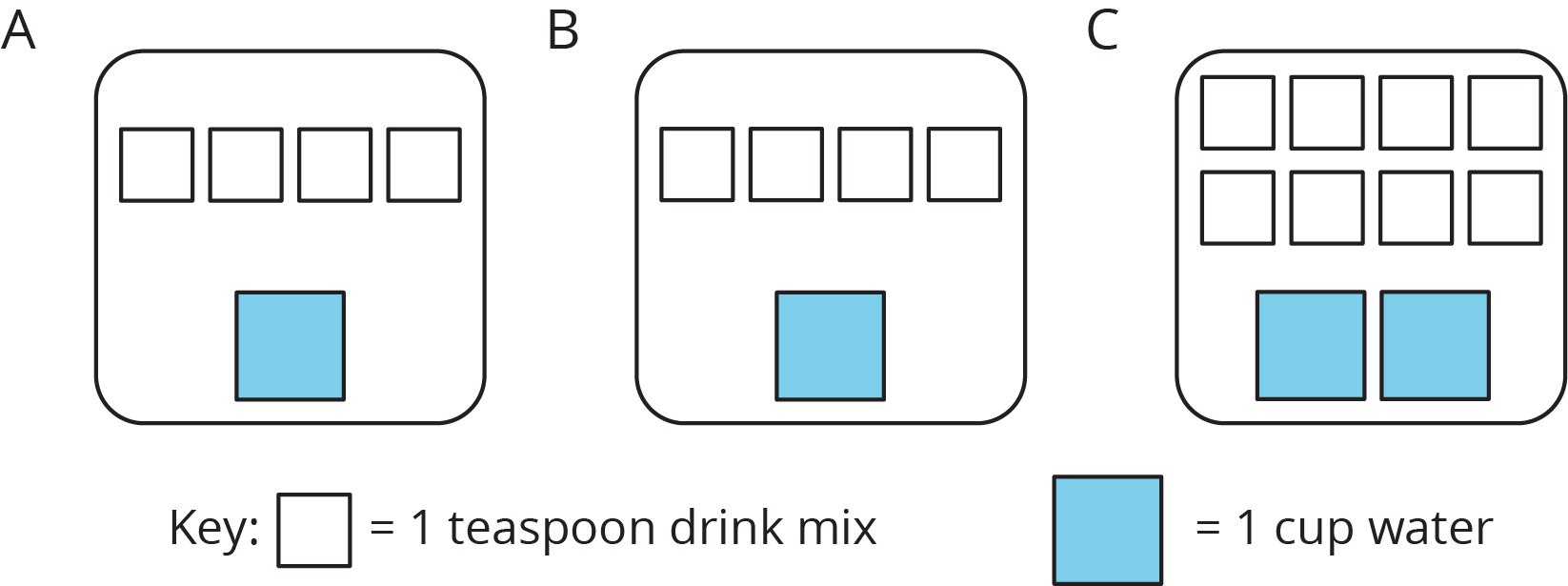
3.2 - Powdered Drink Mix

Here are diagrams representing three mixtures of powdered drink mix and water:

• Show Diagrams
  

1. How would the taste of Mixture A compare to the taste of Mixture B?

2. Use the diagrams to complete each statement:

a. Mixture B uses ______ cups of water and ______ teaspoons of drink mix. The ratio of cups of water to teaspoons of drink mix in Mixture B is ________.
Mixture C uses ______ cups of water and ______ teaspoons of drink mix. The ratio of cups of water to teaspoons of drink mix in Mixture C is ________.

3. How would the taste of Mixture B compare to the taste of Mixture C?

• See Possible Answers

  1. Mixture A would taste the same as Mixture B. The ratios of drink mix to water are the same in both.

  2. a. Mixture B uses 1 cup of water and 4 teaspoons of drink mix. The ratio of cups of water to teaspoons of drink mix in Mixture B is 1:4.
  Mixture C uses 2 cups of water and 8 teaspoons of drink mix. The ratio of cups of water to teaspoons of drink mix in Mixture C is 2:8, which is the same as 1:4.

  3. Mixture B would taste the same as Mixture C. Mixture C uses more water and more drink mix, but the ratio of water to drink mix is the same.

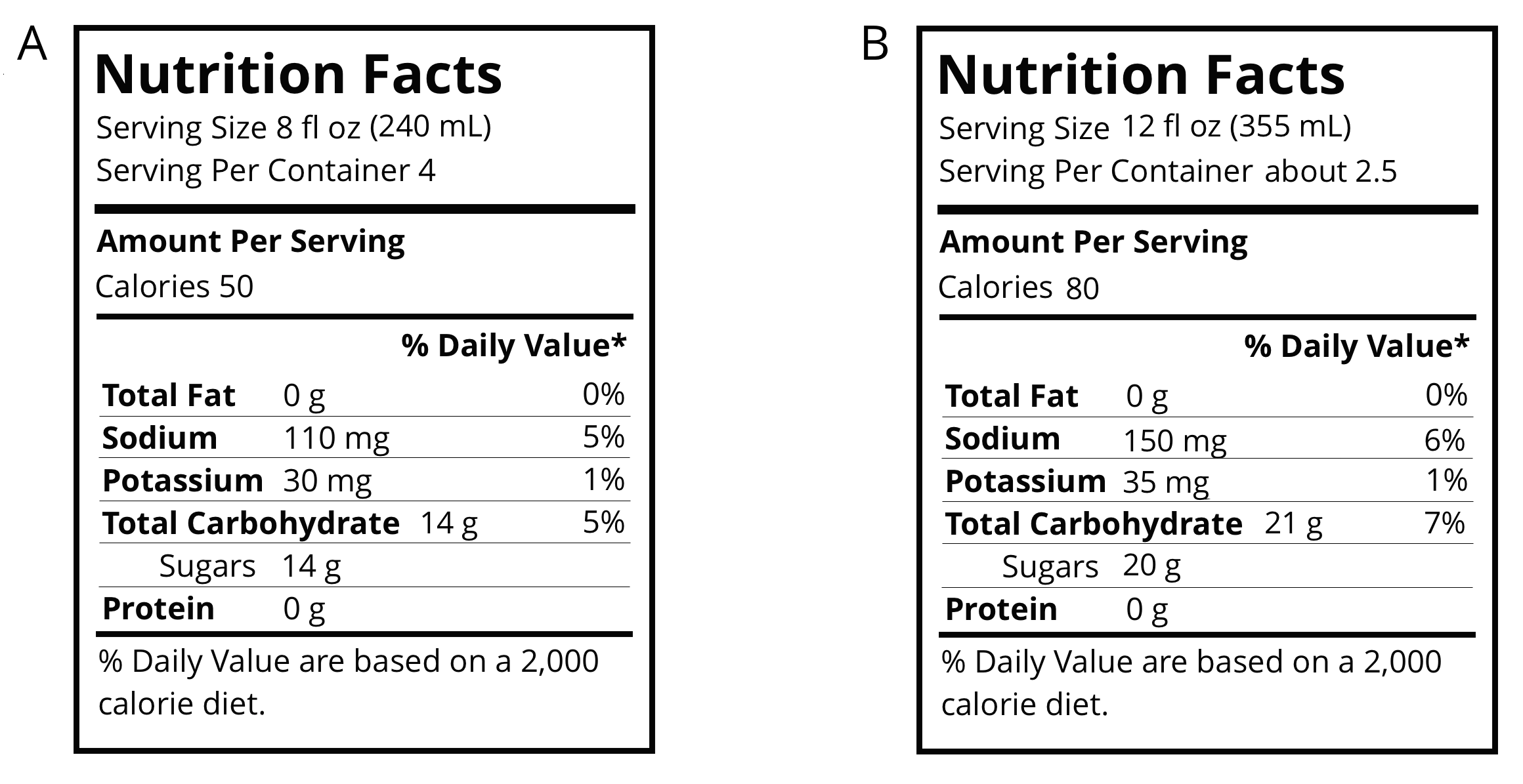
Sports drinks use sodium (better known as salt) to help people replenish electrolytes. Here are the nutrition labels of two sports drinks.

• Show Labels
  

1. Which of these drinks is saltier? Explain how you know.

2. If you wanted to make sure a sports drink was less salty than both of the ones given, what ratio of sodium to water would you use?

• Hints

  Look at the sodium content per serving. The second drink has more sodium per serving, but it also has larger servings. How much sodium is there for every fluid ounce/mL of a serving?

• See Possible Answers

  1. The first drink is saltier. As a ratio, the first drink has 110 ÷ 8 = 13.75 mg sodium for every fluid ounce, while the second drink has 150 ÷ 12 = 12.5 mg sodium for every fluid ounce.

  2. Use a lower ratio of sodium to water, e.g. 11 mg sodium for every fluid ounce of water.

3.3 - Batches of Cookies

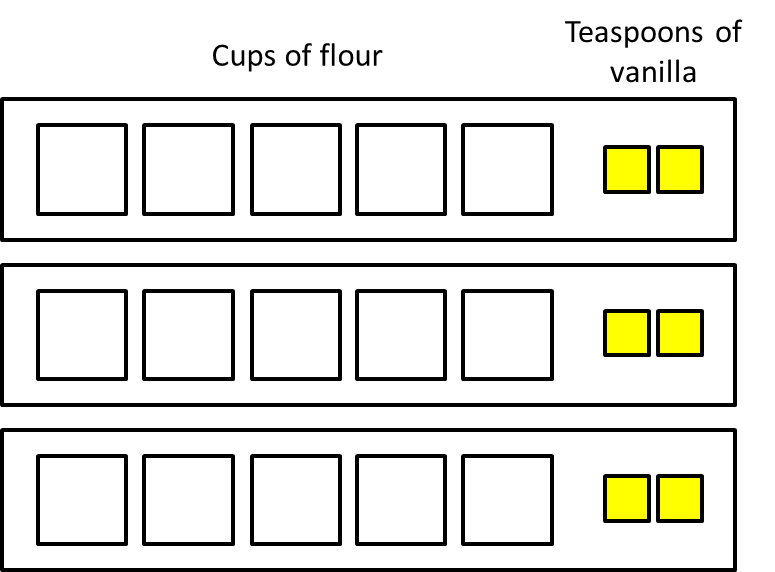
A recipe for one batch of cookies calls for 5 cups of flour and 2 teaspoons of vanilla.

1. Draw a diagram that shows the amount of flour and vanilla needed for two batches of cookies.

2. How many batches can you make with 15 cups of flour and 6 teaspoons of vanilla? Indicate the additional batches by adding more ingredients to your diagram.

3. How much flour and vanilla would you need for 5 batches of cookies?

4. Whether the ratio of cups of flour to teaspoons of vanilla is 5:2, 10:4, or 15:6, the recipes would make cookies that taste the same. We call these equivalent ratios.
a. Find another ratio of cups of flour to teaspoons of vanilla that is equivalent to these ratios.
b. How many batches can you make using this new ratio of ingredients?

• See Possible Answers

  1.
  

  2. 15 = 5 cups of flour × 3
  6 = 2 teaspoons of vanilla × 3
  With 15 cups of flour and 6 teaspoons of vanilla, you could make 3 batches of cookies:
  

  3. 5 cups of flour × 5 = 25
  2 teaspoons of vanilla × 5 = 10
  You would need 25 cups of flour and 10 teaspoons of vanilla to make 5 batches of cookies.

  4. a. 30:12
  b. You could make 6 batches of cookies with this ratio of ingredients.

Lesson 3 Summary

A recipe for fizzy juice says, "Mix 5 cups of cranberry juice with 2 cups of soda water."

To double this recipe, we would use 10 cups of cranberry juice with 4 cups of soda water. To triple this recipe, we would use 15 cups of cranberry juice with 6 cups of soda water.

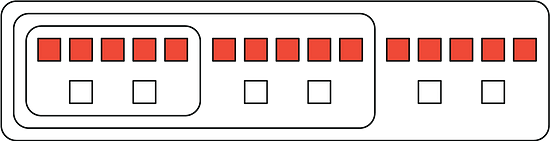
This diagram shows a single batch of the recipe, a double batch, and a triple batch:

• Show Diagram
  

We say that the ratios 5:2, 10:4, and 15:6 are equivalent. Even though the amounts of each ingredient within a single, double, or triple batch are not the same, they would make fizzy juice that tastes the same.

Practice Problems

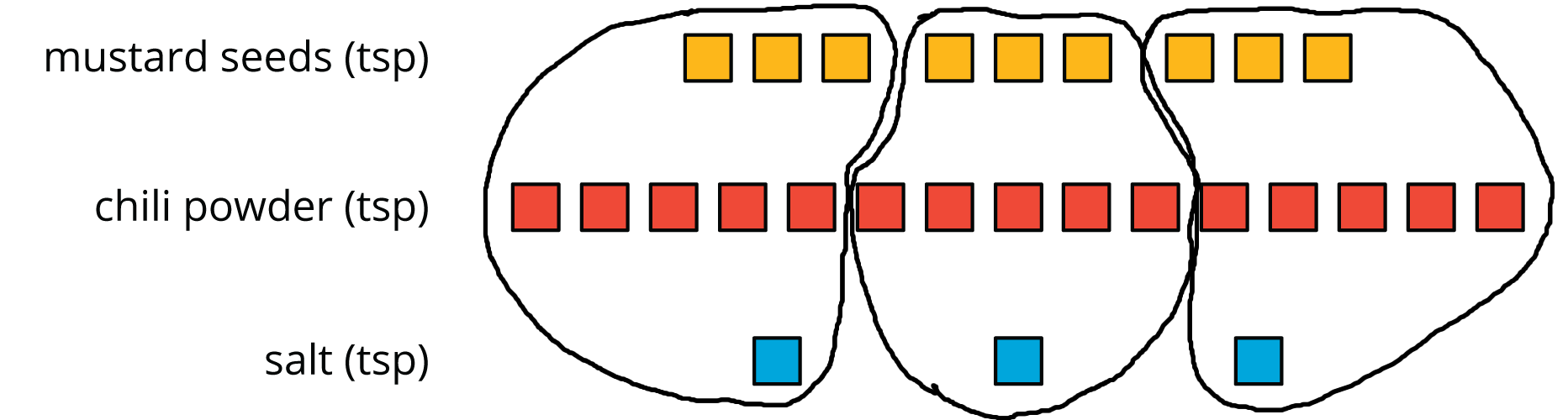
1. A recipe for 1 batch of spice mix says, "Combine 3 teaspoons of mustard seeds, 5 teaspoons of chili powder, and 1 teaspoon of salt." How many batches are represented by the diagram? Explain or show your reasoning.

• Show Diagram
  

• Answers

  There are 3 batches in the diagram:

  

  Dividing any one of the quantities seen here by the number of teaspoons of an ingredient per batch will give this answer.

  9 squares of mustard seeds ÷ 3 teaspoons of mustard seeds per batch = 3 batches
  15 ÷ 5 teaspoons of chili powder per batch = 3 batches
  3 ÷ 1 teaspoon of salt per batch = 3 batches

2. Priya makes chocolate milk by mixing 2 cups of milk and 5 tablespoons of cocoa powder. Draw a diagram that clearly represents two batches of her chocolate milk.

• See Possible Answers

  

  Two batches of chocolate milk would have 4 cups of milk and 10 tablespoons of cocoa powder.

3. In a recipe for fizzy grape juice, the ratio of cups of sparkling water to cups of grape juice concentrate is 3 to 1.
a. Find two more ratios of cups of sparkling water to cups of juice concentrate that would make a mixture that tastes the same as this recipe.
b. Describe another mixture of sparkling water and grape juice that would taste different than this recipe.

• See Possible Answers

  a. You can make equivalent fractions by multiplying both numbers in the ratio by the same multiplier.
  Equivalent ratios for 3:1 include 6:2 (3 × 2 : 1 × 2) and 9:3 (3 × 3 : 1 × 3).

  b. A non-equivalent ratio would taste different, e.g. 3:2 sparkling water to juice concentrate.

4. Write the missing number under each tick mark on the number line.

• Show Number Line
  

• Answers

  

  The missing numbers are 24 and 36. This number line shows skip-counting by 6, or multiples of 6.

5. At the kennel, there are 6 dogs for every 5 cats.

a. The ratio of dogs to cats is ______ to ______.
b. The ratio of cats to dogs is ______ to ______.
c. For every ______ dogs there are ______ cats.
d. The ratio of cats to dogs is ______ : ______.

• Answers

  a. The ratio of dogs to cats is 6 to 5.
  b. The ratio of cats to dogs is 5 to 6.
  c. For every 6 dogs there are 5 cats.
  d. The ratio of cats to dogs is 5 : 6.

6. Elena has 80 unit cubes. What is the volume of the largest cube she can build with them?

• Answers

  The largest perfect cube which is less than 80 is 4³ = 64. (The next largest is 5³ = 125, which is greater than 80.)

7. Fill in the blanks to make each equation true.

a. 3 · ¹⁄₃ = ______
b. 10 · ¹⁄₁₀ = ______
c. 19 · ¹⁄₁₉ = ______
d. a · ¹⁄_a = ______ (As long as a does not equal 0.)
e. 5 · ______ = 1
f. 17 · ______ = 1
g. b · ______ = 1

• Answers

  a. 3 · ¹⁄₃ = 1
  b. 10 · ¹⁄₁₀ = 1
  c. 19 · ¹⁄₁₉ = 1
  d. a · ¹⁄_a = 1 (As long as a does not equal 0.)
  e. 5 · ¹⁄₅ = 1
  f. 17 · ¹⁄₁₇ = 1
  g. b · ¹⁄_b = 1

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.