Reflection Transformation
In these lessons, we will learn
- what is reflection?
- how to draw the reflected image of an object (drawn on grid lines) given the line of reflection.
- how to draw the reflected image of an object (using a compass or ruler) given the line of reflection.
- how to reflect points and shapes on the coordinate plane using the Coordinate Rules.
- how to reflect an object using a transformation matrix.
Related Pages
Properties Of Reflection
Transformation
More Lessons On Geometry
What is Reflection?
In a reflection transformation, all the points of an object are reflected or flipped on a line called the axis of reflection or line of reflection.
Example:
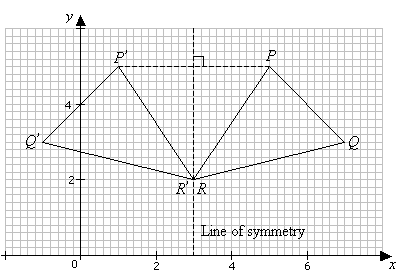
A reflection is defined by the axis of symmetry or mirror line. In the above diagram, the mirror line is x = 3.
Under reflection, the shape and size of an image is exactly the same as the original figure. This type of transformation is called isometric transformation.
The orientation is laterally inverted, that is they are facing opposite directions.
The line of reflection is the perpendicular bisector of the line joining any point and its image (e.g. PP ’ in the above figure).
All the points on the mirror line are not changed. These points are said to be invariant. (R is an invariant point in the above.)
Drawing The Image on Grid Lines
If the axis of reflection is on one of the grid lines, we just count the number of squares from a point on the object to the axis and the image is the same distance from the axis.
Example:
In the diagram, the figure A is reflected in the line XY. Draw the image of A in the diagram.
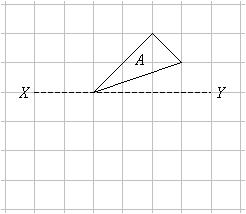
Solution:
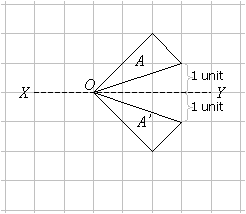
Note that the point O remained unchanged under reflection because it is on the axis of reflection. Any point on the line of reflection is unchanged – such points are described as invariant.
How to reflect a shape on squared paper without using tracing paper
This video shows how to reflect a shape on squared paper without using tracing paper. Just
count the distance of each corner to the mirror line and count the same distance away from
the mirror line. Once all the points have been reflected them, join the points up neatly
using your ruler.
Draw the image using a compass
If the axis of reflection is not on the grid lines, we will need to use a compass to construct the image.
Example:
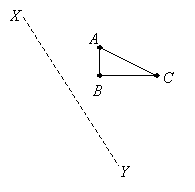
In the diagram below, the triangle ABC is reflected in the line XY. Draw the image of the triangle in the diagram.
Solution:
Step 1: Place the sharp point of a compass at A and draw two
arcs intersecting the line XY

Step 2: Place the sharp point of the compass on the first intersecting point and mark an arc on the opposite side of XY. Place the sharp point of the compass on the second intersecting point and mark an arc to intersect with the first arc. The intersection is the image of A’.
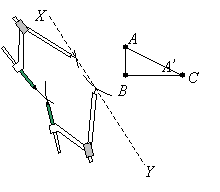
Step 3: Repeat steps 1 and 2 to get the points B ’ and C ’. Join the points A ’ , B ’ and C ’ to get the image A ’ B ’ C ’.

Construct Reflection by Hand
How to reflect a figure over a line by hand using a ruler.
How to construct a Line of Reflection?
Construct a line of reflection given the object and the image.
Reflection on the Coordinate Plane
We will now look at how points and shapes are reflected on the coordinate plane. It will be helpful to note the patterns of the coordinates when the points are reflected over different lines of reflection.
Coordinate Rules for Reflection
If (a, b) is reflected on the x-axis, its image is the point (a, -b)
If (a, b) is reflected on the y-axis, its image is the point (-a, b)
If (a, b) is reflected on the line y = x, its image is the point (b, a)
If (a, b) is reflected on the line y = -x, its image is the point (-b, a)
Geometry Reflection
A reflection is an isometry, which means the original and image are congruent, that can be
described as a “flip”. To perform a geometry reflection, a line of reflection is needed;
the resulting orientation of the two figures are opposite. Corresponding parts of the
figures are the same distance from the line of reflection. Ordered pair rules reflect over
the x-axis: (x, -y), y-axis: (-x, y), line y = x: (y, x).
This video shows reflection over the x-axis, y-axis, x = 2, y = −2
This video shows reflection over y = x, y = − x. A reflection that results in an overlapping shape.
This video shows reflection over the x-axis, y-axis, x = −3, y = 5, y = x, and y = − x.
Reflections using Matrices
This lesson involves reflections in the coordinate plane. We use coordinate rules as well as matrix multiplication to reflect a polygon (or polygon matrix) about the x-axis, y-axis, the line y = x or the line y = -x.
Matrices and Reflections
Performing reflections with matrices over the y-axis and x-axis.
Matrices for Reflections over the y-axis and x-axis.
Matrices for Reflections over the line y = x.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.