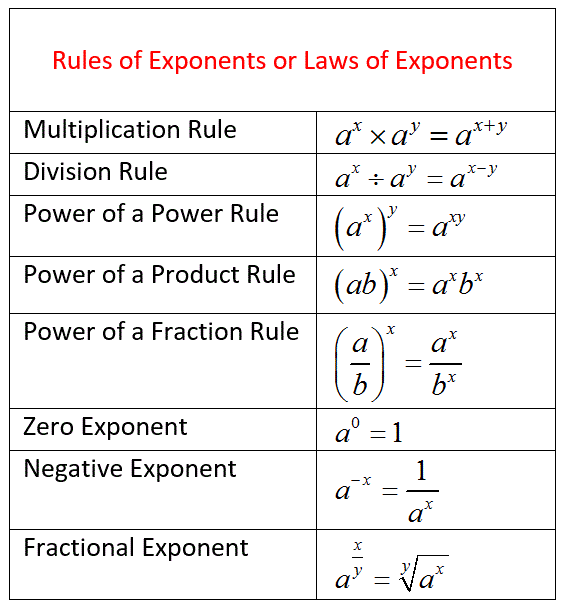
Rules of Exponents or Laws of Exponents

What are the Rules of Exponents?

Multiplication or Product Rule:
To multiply powers with the same base, keep the base the same and add the exponents.

Division or Quotient Rule:
To divide powers with the same base, keep the base the same and subtract the exponents.

Power of a Power Rule:
When a power has an exponent, keep the base the same and multiply the exponents.

Some of the Rules of Exponents or Laws of Exponents are summarized in the following table. Scroll down the page for examples and solutions on how to use the Rules of Exponents.

We will now consider combined operations of multiplication and division on numbers in exponent form, using all the rules of exponents introduced above.

Example:
Simplify the following expressions, giving your answers in exponent form:

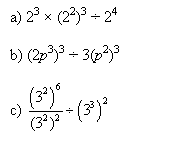
Solution:

Rules of Exponents Worksheet

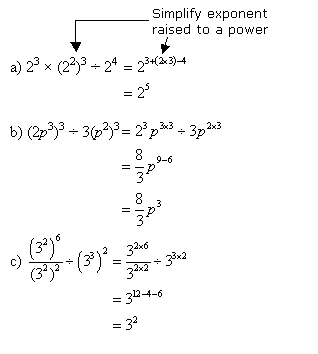
How to simplify expressions using the Product Rule of Exponents?
The product rule of exponents states that to multiply exponential terms with the same base, add the exponents.

Example:
Write each of the following products using a single base. Do not simplify further.

1. x² • x⁴
2. (-2)⁴ • (-2)¹
3. y⁴ • y⁵ • y

• Show Video Lesson

How to simplify expressions using the Quotient Rule of Exponents?
The quotient rule of exponents states that to divide exponential terms with the same base, subtract the exponents.

Example:
Write each of the following products using a single base. Do not simplify further.

1. t⁷ / t³
2. (-2)¹⁵ / (-2)¹²

• Show Video Lesson

How to simplify expressions using the Power Rule of Exponents?

Example:
Write each of the following products using a single base. Do not simplify further.

1. (x³)²
2. ((2y)⁴)³
3. ((-3)⁴)⁶

• Show Video Lesson

How to simplify expressions using the Power of a Product Rule of Exponents?

Example:
Simplify each expression.

1. (x³)²
2. (3^az)⁶
3. (-4b⁵)²
4. 1/(-2p)⁴
5. (a^-3b²)⁴

• Show Video Lesson

Example:
Simplify the following expressions:
a) (x²/2)⁴
b) (4n⁶)²

• Show Video Lesson

How to simplify expressions using the Power of a Quotient Rule of Exponents?

Example:
Simplify each expression.

1. (2/x⁴)³
2. (p³/q)⁴
3. (-1/y³)¹²
4. (m²n^-4)³
5. (3^-2z^-3)²

• Show Video Lesson

How to simplify expressions using the Quotient and Zero Exponent Rules?

Example:
Simplify each expression using the zero exponent rule. Assume all variables are nonzero.

• Show Video Lesson

How to simplify expressions using the Quotient and Negative Exponent Rules?

Example:
Write each quotient using a single base. Do not simplify further. Write all answers with positive exponents.

• Show Video Lesson

How to simplify expressions using the Product, Quotient and Zero Exponent Rules?

Example:
Write each expression using a single base. Do not simplify further. Write all answers with positive exponents.

• Show Video Lesson

Tutorial on the Basic Exponent Rules

Multiplication, Division, Power of a Power

• Show Video Lesson

Learn exponent rules through music and songs

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.