Illustrative Mathematics Grade 7, Unit 8, Lesson 17: More about Sampling Variability

Related Pages
Illustrative Math
Grade 7

Lesson 17: More about Sampling Variability

Let’s compare samples from the same population.

Illustrative Math Unit 7.8, Lesson 17 (printable worksheets)

Lesson 17 Summary

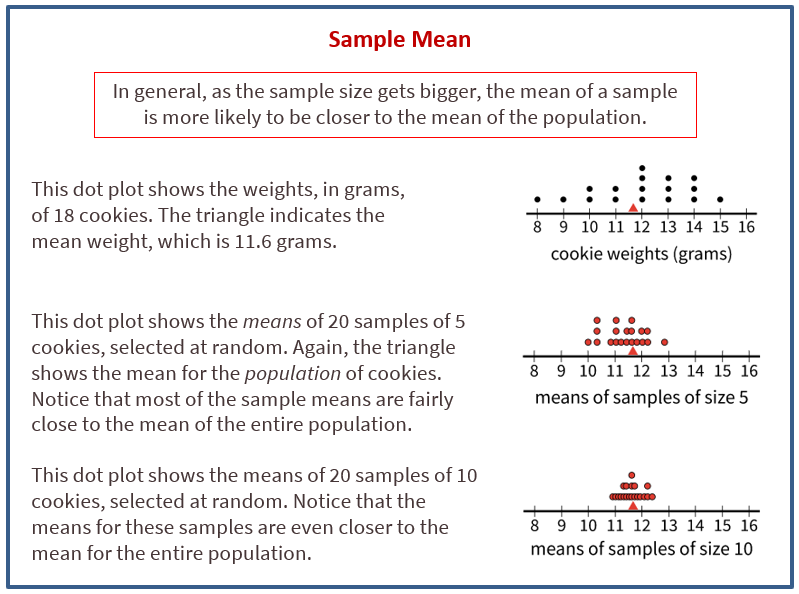
The following diagram as the sample size gets bigger, the sample mean is more likely to be close to the population mean.

Lesson 17.1 Average Reactions

The other day, you worked with the reaction times of twelfth graders to see if they were fast enough to help out at the track meet. Look back at the sample you collected.

1. Calculate the mean reaction time for your sample.
2. Did you and your partner get the same sample mean? Explain why or why not.

• See Video for Whole Lesson

Lesson 17.2 Reaction Population

Your teacher will display a blank dot plot.

1. Plot your sample mean from the previous activity on your teacher’s dot plot.
2. What do you notice about the distribution of the sample means from the class?
   a. Where is the center?
   b. Is there a lot of variability?
   c. Is it approximately symmetric?
3. The population mean is 0.442 seconds. How does this value compare to the sample means from the class?
   Pause here so your teacher can display a dot plot of the population of reaction times.
4. What do you notice about the distribution of the population?
   a. Where is the center?
   b. Is there a lot of variability?
   c. Is it approximately symmetric?
5. Compare the two displayed dot plots.
6. Based on the distribution of sample means from the class, do you think the mean of a random sample of 20 items is likely to be:
   a. within 0.01 seconds of the actual population mean?
   b. within 0.1 seconds of the actual population mean?
   Explain or show your reasoning.

Lesson 17.3 How Much Do You Trust the Answer?

The other day you worked with 2 different samples of viewers from each of 3 different television shows. Each sample included 10 viewers. Here are the mean ages for 100 different samples of viewers from each show.

1. For each show, use the dot plot to estimate the population mean.
   a. Trivia the Game Show
   b. Science Experiments YOU Can Do
   c. Learning to Read
2. For each show, are most of the sample means within 1 year of your estimated population mean?
3. Suppose you take a new random sample of 10 viewers for each of the 3 shows. Which show do you expect to have the new sample mean closest to the population mean? Explain or show your reasoning.

Are you ready for more?

Market research shows that advertisements for retirement plans appeal to people between the ages of 40 and 55. Younger people are usually not interested and older people often already have a plan. Is it a good idea to advertise retirement plans during any of these three shows? Explain your reasoning.

Lesson 17 Practice Problems

1. One thousand baseball fans were asked how far they would be willing to travel to watch a professional baseball game. From this population, 100 different samples of size 40 were selected. Here is a dot plot showing the mean of each sample.
   Based on the distribution of sample means, what do you think is a reasonable estimate for the mean of the population?
2. Last night, everyone at the school music concert wrote their age on a slip of paper and placed it in a box. Today, each of the students in a math class selected a random sample of size 10 from the box of papers. Here is a dot plot showing their sample means, rounded to the nearest year.
   a. Does the number of dots on the dot plot tell you how many people were at the concert or how many students are in the math class?
   b. The mean age for the population was 35 years. If Elena picks a new sample of size 10 from this population, should she expect her sample mean to be within 1 year of the population mean? Explain your reasoning.
   c. What could Elena do to select a random sample that is more likely to have a sample mean within 1 year of the population mean?
3. A random sample of people were asked which hand they prefer to write with.
   l means they prefer to use their left hand.
   r means they prefer to use their right hand.
   Based on this sample, estimate the proportion of the population that prefers to write with their left hand.
4. Andre would like to estimate the mean number of books the students at his school read over the summer break. He has a list of the names of all the students at the school, but he doesn’t have time to ask every student how many books they read.
   What should Andre do to estimate the mean number of books?
5. A hockey team has a 75% chance of winning against the opposing team in each game of a playoff series. To win the series, the team must be the first to win 4 games.
   a. Design a simulation for this event.
   b. What counts as a successful outcome in your simulation?
   c. Estimate the probability using your simulation.

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.