Scale Factor, Perimeter, Area & Volume of Similar Figures
Related Pages
Scale Factor & Shapes
Scaling and Area
Surface Area of Similar Figures
Grade 8
In these lessons, we will learn the scale factors of similar figures, the ratio of lengths, perimeters, areas and volumes of similar figures; suitable for Grade 7 or Grade 8 Math.
Scale Factor
A scale factor is the factor by which all the components of an object are multiplied in order to create a proportional enlargement or reduction. It tells you how much larger or smaller one shape is compared to another. If the scale factor is greater than 1, the shape gets bigger (an enlargement). If the scale factor is between 0 and 1, the shape gets smaller (a reduction).
The following diagram shows an example of scale factor. Scroll down the page for more examples and solutions on how to use scale factors.
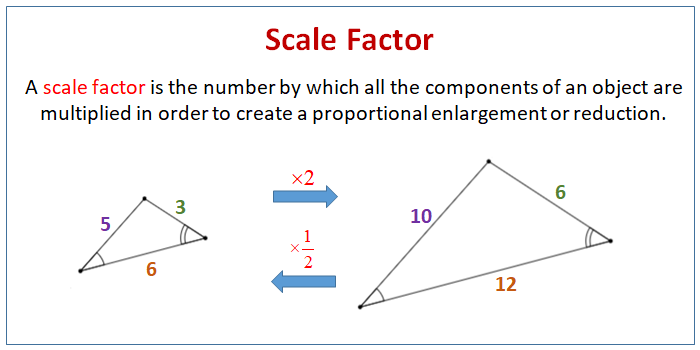
How to Find a Scale Factor:
- Identify Corresponding Sides: Look for sides on the original shape and the new shape that match up. These are called corresponding sides.
- Create a Ratio: Divide the length of a side on the new shape by the length of the corresponding side on the original shape.
Scale Factor = (New Length) / (Original Length) - Simplify the Ratio: If possible, simplify the ratio to its simplest form.
How to use scale factor to sketch a proportional scale model?
We can multiply the lengths of the original shape by the scale factor to get the new length.
(New Length) = Scale Factor × (Original Length)
Example:
- The scale factor of a model car is 1:24. If the actual car is 18 ft long and 8 ft wide, how long and wide will the model car be in inches?
- You need to sketch a ladybug using a scale factor of 12. If a ladybug is 8 mm long and 4 mm wide, what will be the length and width of the sketch be in cm?
Scale factor for similar geometric figures
Scale Factor is defined as the ratio of any two corresponding lengths in two similar geometric figures.
Similar Figures are figures such that:
- Corresponding angles are congruent.
- Corresponding side lengths are proportional.
Applying Scale Factor to Perimeter & Area
When a shape is scaled, its area changes by the square of the scale factor.
How to find the ratio of areas and ratios of perimeters for similar polygons.
Ratio of perimeters = ratio of sides
Ratio of areas = (ratio of sides)2
Scale Factor, Perimeter Ratio & Area Ratio
Example:
Given that the polygon in each pair are similar. Find the scale factor, perimeter ratio and area ratio.
Areas and Perimeters of Similar Figures
This video discusses how to find the ratio of the perimeters and the ratio of the areas of similar
figures from the scale factor. Also how to use these ratios to find missing perimeters and areas.
If the scale factor of two similar figures is a/b, then
- the ratio of their perimeters is a/b and
- the ratio of their areas is a2/b2
Examples:
- The trapezoids at the right are similar. The ratio of the lengths of corresponding sides is 6/9 or 2/3.
a) What is the ratio (smaller to larger) of the perimeters?
b) What is the ratio (smaller to larger) of the areas? - Two similar polygons have corresponding sides in the ratio 5:7.
a) What is the ratio (larger to smaller) of their perimeters?
b) What is the ratio (larger to smaller) of their areas? - The area of the smaller regular pentagon is about 27.5 cm
2. What is the best approximation for the area of the larger regular pentagon?< - If the area of the smaller triangle is about 39 ft2, what is the area of the larger triangle to the nearest tenth?
- The triangles are similar. What is the scale factor? What is the ratio of their perimeters?
- The areas of two similar rhombuses are 48m2 and 128m2. What is the ratio of their perimeters?
How does scale factor impact side lengths, perimeter, area, and angles?
If the scale factor from A to B is x then
The side lengths of B will be x times larger than A
The perimeter of B will be x times larger than A
The area of B will be x2 times larger than A
A and B will have the same shape and angles.
Applying Scale Factor to Area & Volume
Scale Factor, Length, Area and Volume for similar shapes
Ratio of lengths = ratio of sides = scale factor
Ratio of surface areas = (ratio of sides)2 = (scale factor)2
Ratio of volume = (ratio of sides)3 = (scale factor)3
Surface Areas And Volumes Of Similar Solids
Similar solids have the same shape, and all their corresponding dimensions are proportional.
If the scale factor of two similar solids is a:b, then
• the ratio of their corresponding areas is a2:b2
• the ratio of their volumes is a3:b3
Examples:
- Are the two rectangular prisms similar? If so, what is the scale factor of the first figure to the second figure?
- The square prisms at the right are similar. What is the scale factor of the smaller prism to the larger prism?
- What is the scale factor of two similar prisms with surface areas 144 m2 and 324 m2?
- The volumes of two similar solids are 128 m3 and 250 m3. The surface area of the larger solid is 250 m2. What is the surface area of the smaller solid?
Similar Figures, Scale Factor, Area & Volume Ratios
Examples:
- The scale factor between two similar figures is given. The surface area and volume of the
smaller figure are given. Find the surface area and volume of the larger figure.
Scale factor 5:6
SA: 275 cm2
V: 3000 cm3 - Some information about the surface area and volume of two similar solids has been given. Find the missing value.
3D Figures - Scale Models and Factors
Learn about scaling 3D figures using scale factor
Problem: A 6 cm by 2 cm rectangular prism is built from small rectangular prisms of length 3 cm.
a) What is the scale factor from the smaller to the larger model?
b) Find the width and height of the smaller rectangular prisms.
c) Compare the surface area of the two rectangular prisms.
d) Compare the volume of the two rectangular prisms.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.