

Scatter Plot and Line of Best Fit
Related Topics:
More Lessons for Grade 8
Math Worksheets
Examples, solutions, videos, worksheets, and lessons to help Grade 8 students learn about Scatter Plots, Line of Best Fit and Correlation.
A scatter plot or scatter diagram is a two-dimensional graph in which the points corresponding to two related factors are graphed and observed for correlation. A downward trend in points shows a negative correlation. An upward trend in points shows a positive correlation. If there is no trend in graph points then there is no correlation.
The following diagram shows some examples of scatter plots and correlations. Scroll down the page for more examples and solutions using scatter plots, correlations and lines of best fit.
In this video, you will learn that a scatter plot is a graph in which the data is plotted as points on a coordinate grid, and note that a "best-fit line" can be drawn to determine the trend in the data. If the x-values increase as the y-values increase, the scatter plot represents a positive correlation. If the x-values increase as the y-values decrease, the scatter plot represents a negative correlation. If the data is spread out so that it is not possible to draw a "best-fit line", there is no correlation.
Creating a Scatter Plot.
Learn Scatter Plots and Best Fitting Lines.
This video explains what a scatter plot is and how to find the best fitting line. Scatter plot graphs, correlation, line of best fit, univariate data and bivariate data. Scatter Plots : Introduction to Positive and Negative Correlation. Interpreting Scatterplots
Starts with a description of how to plot a scatterplot from a table.
How to interpret scatterplots in terms of direction, form, strength and outliers?


More Lessons for Grade 8
Math Worksheets
Examples, solutions, videos, worksheets, and lessons to help Grade 8 students learn about Scatter Plots, Line of Best Fit and Correlation.
A scatter plot or scatter diagram is a two-dimensional graph in which the points corresponding to two related factors are graphed and observed for correlation. A downward trend in points shows a negative correlation. An upward trend in points shows a positive correlation. If there is no trend in graph points then there is no correlation.
The following diagram shows some examples of scatter plots and correlations. Scroll down the page for more examples and solutions using scatter plots, correlations and lines of best fit.
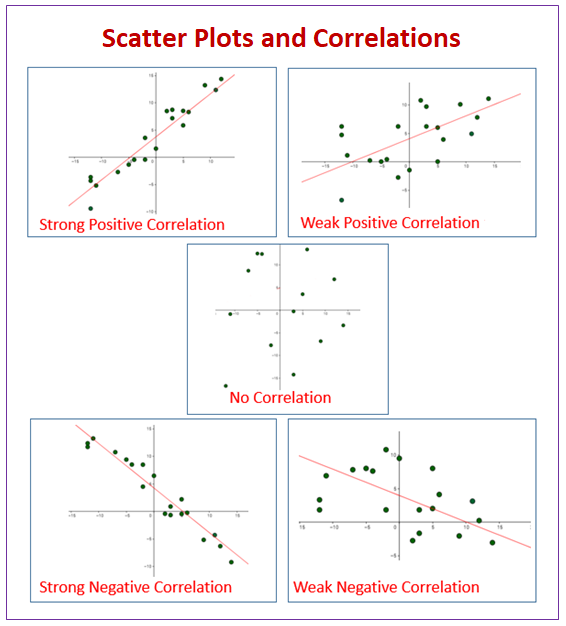
In this video, you will learn that a scatter plot is a graph in which the data is plotted as points on a coordinate grid, and note that a "best-fit line" can be drawn to determine the trend in the data. If the x-values increase as the y-values increase, the scatter plot represents a positive correlation. If the x-values increase as the y-values decrease, the scatter plot represents a negative correlation. If the data is spread out so that it is not possible to draw a "best-fit line", there is no correlation.
This video explains what a scatter plot is and how to find the best fitting line. Scatter plot graphs, correlation, line of best fit, univariate data and bivariate data. Scatter Plots : Introduction to Positive and Negative Correlation. Interpreting Scatterplots
Starts with a description of how to plot a scatterplot from a table.
How to interpret scatterplots in terms of direction, form, strength and outliers?
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.



We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.