Illustrative Mathematics Grade 8, Unit 2, Lesson 9: Side Length Quotients in Similar Triangles

Related Pages
Illustrative Math
Grade 8

Lesson 9: Side Length Quotients in Similar Triangles

Let’s find missing side lengths in triangles.

Illustrative Math Unit 8.2, Lesson 9 (printable worksheets)

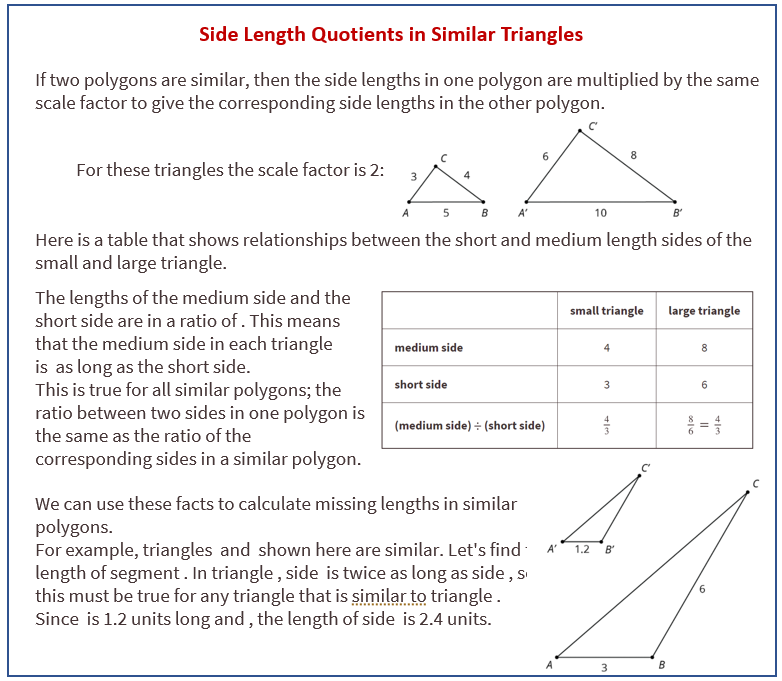
Lesson 9 Summary

The following diagram shows how to decide if two triangles are similar by looking at quotients of lengths of corresponding sides.

Lesson 9.1 Two-three-four and Four-five-six

Triangle A has side lengths 2, 3, and 4. Triangle B has side lengths 4, 5, and 6. Is Triangle A similar to Triangle B?

• See Video for Whole Lesson

Lesson 9.2 Quotients of Sides Within Similar Triangles

Your teacher will assign you one of the three columns in the second table.
Triangle ABC is similar to triangles DEF, GHI, and JKL. The scale factors for the dilations that show triangle ABC is similar to each triangle are in the table.

1. Find the side lengths of triangles DEF, GHI, and JKL. Record them in the first table.
2. For all four triangles, find the quotient of the triangle side lengths assigned to you and record them in the second table. What do you notice about the quotients?
3. Compare your results with your partner’s and complete your table.

Are you ready for more?

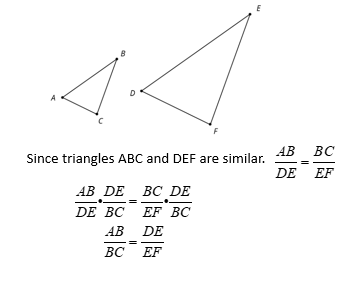
Triangles ABC and DEF are similar. Explain why AB/BC = DE/EF.

• Show Answer

  

Lesson 9.3 Using Side Quotients to Find Side Lengths of Similar Triangles

Triangles ABC, EFD, and GHI are all similar. The side lengths of the triangles all have the same units. Find the unknown side lengths.

Lesson 9 Practice Problems

1. These two triangles are similar.
   What are a and b? Note: the two figures are not drawn to scale.
2. Here is triangle ABC. Triangle XYZ is similar to ABC with scale factor 1/4.
   a. Draw what triangle XYZ might look like.
   b. How do the angle measures of triangle XYZ compare to triangle ABC? Explain how you know.
   c. What are the side lengths of triangle XYZ?
   d. For triangle XYZ, calculate (long side) ÷ (medium side), and compare to triangle ABC.
3. The two triangles shown are similar. Find the value of d/c.
4. The diagram shows two nested triangles that share a vertex. Find a center and a scale factor for a dilation that would move the larger triangle to the smaller triangle.
   
   
   

   The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.