Simple Interest Formula

Interest Formulas
When working with financial calculations, especially in savings, loans, and investments, there are three primary interest formulas you will encounter: simple interest, compound interest, and continuously compounded interest. Each formula calculates interest differently, leading to different final amounts.

The following table gives the Formulas for Simple Interest, Compound Interest, and Continuously Compounded Interest. Scroll down the page for more examples and solutions.

 

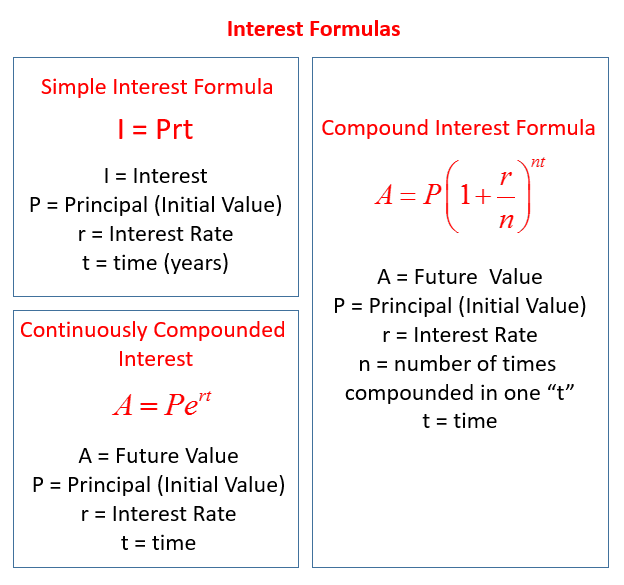
Interest & Percent Worksheets
Practice your skills with the following worksheets:

• Printable & Online Percent Word Problems Worksheets

  Printable
  Percent Word Problems (6th Grade)
  Percent Word Problem (7th Grade)
  Markup/Markdown Word Problems
  Percent Error Problems
  Simple Interest Word Problems
  Percentage Word Problems
  Percent Population Problems
  

  Online
  Percent Word Problems
  Percentage Word Problems
  Percent Word Problems (Profit, Loss)
  Percent Word Problems (Increase, Decrease)

1. Simple Interest
Simple interest is only based on the original principal amount. The interest earned each period is not added back to the principal for subsequent interest calculations.
Formula for Simple Interest (I):
I=P×r×t
Formula for Total Amount (A):
A=P+I
Where:
I = Interest earned/paid
P = Principal amount (initial sum)
r = Annual interest rate (in decimal form, e.g., 5% = 0.05)
t = Time (in years)
A = Total amount after interest

2. Compound Interest
Compound interest is “interest on interest.” The interest earned in each period is added to the principal, and the interest for the next period is calculated on this new, larger amount. This leads to exponential growth over time.
Formula for Total Amount (A):
\(A=P\left( 1+\frac{r}{n} \right)^{nt}\)
Formula for Compound Interest (CI):
CI=A−P
Where:
P = Principal amount (the initial amount of money)
r = Annual interest rate (as a decimal)
t = Time period (in years)
n = Number of times interest is compounded per year (compounding frequency)

3. Continuously Compounded Interest
Continuously compounded interest is an extreme case of compound interest where the interest is compounded an infinite number of times per year. While not practical in the real world, this formula is essential in financial theory and for modeling exponential growth. It uses the mathematical constant e (Euler’s number, approximately 2.71828).
Formula for Total Amount (A):
\(A=Pe^{rt}\)
Where:
P = Principal amount
e = Euler’s number
r = Annual interest rate (as a decimal)
t = Time period (in years)
A = Total amount after a given time period

Steps to Use the Simple Interest Formula

1. Identify the Given Values:
   Determine the principal (P), interest rate (r), and time (t).
   
2. Convert the Interest Rate:
   If the interest rate is given as a percentage, convert it to a decimal by dividing by 100. For example, 5% becomes 0.05.
   
3. Plug Values into the Formula:
   Substitute P, r, and t into the formula I=P×r×t.
   
4. Calculate the Interest:
   Perform the multiplication to find the interest.
   
5. Calculate the Total Amount (if needed):
   Add the interest to the principal to find the total amount.
   

Example:
Sarah deposits $4,000 at a bank at an interest rate of 4.5% per year. How much interest will she earn at the end of 3 years?

Solution:
Simple Interest = 4,000 × 4.5% × 3 = 540

She earns $540 at the end of 3 years.

Example:
Wanda borrowed $3,000 from a bank at an interest rate of 12% per year for a 2-year period. How much interest does she have to pay the bank at the end of 2 years?

Solution:
Simple Interest = 3,000 × 12% × 2 = 720

She has to pay the bank $720 at the end of 2 years.

Example:
Raymond bought a car for $40, 000. He took a $20,000 loan from a bank at an interest rate of 15% per year for a 3-year period. What is the total amount (interest and loan) that he would have to pay the bank at the end of 3 years?

Solution:
Simple Interest = 20,000 × 13% × 3 = 7,800

At the end of 3 years, he would have to pay

$20,000 + $7,800 = $27,800

Tutorial On Simple Interest

Examples:

1. Ian is investing $4,000 for 2 years. The interest rate is 5.5%. How much interest will Ian earn after 2 years?
2. Doug made a 3 year investment. The interest rate was 4.5%. After 3 years, he earned $675 in interest. How much was his original investment?
3. Kim got a loan of $4700 to buy a used car. The interest rate is 7.5%. She paid $1057.50 in interest. How many years did it take her to pay off her loan?

• Show Video Lesson

How To Solve Interest Problems Using The Simple Interest Formula?

Interest represents a change in money.

If you have a savings account, the interest will increase your balance based upon the interest rate paid by the bank.

If you have a loan, the interest will increase the amount you owe based upon the interest rate charged by the bank.

Examples:

1. If you invest $3,500 in savings account that pays 4% simple interest, how much interest will you earn after 3 years? What ill the new balance be?
2. You borrow $6000 from a loan shark. If you will owe $7200 in 18 months, what would be the simple interest rate?

• Show Video Lesson

How To Use The Formula For Simple Interest To Find The Principal, The Rate Or The Time?

Examples:

1. An investment earned $11.25 interest after 9 months. The rate was 5%. What was the principal?
2. $2000 was invested for 3 years. It earned $204 in interest. What was the rate?
3. A loan of $1200 had $36 in interest. The rate was 6%. What was the length of the loan?

• Show Video Lesson

How To Solve Simple Interest Problems, Compound Interest Problems, Continuously Compounded Interest Problems, And Determining The Effective Rate Of Return?

Examples Of Simple Interest Problems:

1. Joseph buys a new home using an interest only loan where he pays only the interest on the value of the home each month. The home is valued at $200,000 and Joesph pays 5% interest per year on the home. How much is his monthly interest payment?
2. Anthony puts $10000 dollars into a savings account that pays interest every month at a rate of 1.8% per year. How much money does Anthony have after one month? If he leaves his original investment and the first month of interest in the account, how much will he have after the second month?

Examples Of Compound Interest Problems:

1. Matt is saving for a new car. He invests $5000 into an account that pays 3% interest a year and is compound monthly. How much will he have after 5 years?
2. Matt is planning to buy a car in three years. He wants to invest $5000 now and hopes to have $6000 to spend on the car when he buys it. What kind of interest rate would he need if his investment is compounded monthly?

Examples Of Continuously Compound Interest Problems:

1. Lindsey invests $1000 into an account with 4% per year continuously compounded interest. How much will she have after 10 years? How long will it take for her investment to double?
2. Tony and Matt both invest $5000 in an account that receives 3% interest annually for 10 years. Tony invests in an account that is compounded monthly. Matt invests in an account that is compounded continuously. Who made the better investment?

Example Of Effective Rate Of Return:

1. If $2500 is invested at 5% compounded monthly, what is the effective rate of return. What is the effective rate of return if this investment is compounded semiannually?

• Show Video Lesson

Key Points to Remember

1. Simple Interest:
   Interest is calculated only on the principal amount.
   Does not account for interest earned on previously accumulated interest.
   
2. Interest Rate:
   Always convert the interest rate from a percentage to a decimal before using the formula.
   
3. Time:
   Ensure the time t is in years. If given in months or days, convert it to years.

Try out our new and fun Fraction Concoction Game.

Add and subtract fractions to make exciting fraction concoctions following a recipe. There are four levels of difficulty: Easy, medium, hard and insane. Practice the basics of fraction addition and subtraction or challenge yourself with the insane level.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.