Sine Graph

Related Pages
Trigonometric Functions
Trigonometric Graphs
Trigonometric Identities
Lessons On Trigonometry

We will start with the unit circle.

A unit circle is a circle of radius one unit with its center at the origin.

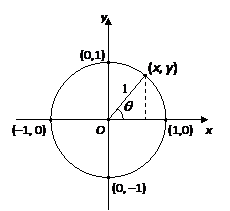
For a point (x, y) on the unit circle;

y = sin θ is known as the sine function.

Using the unit circle, we can plot the values of y against the corresponding values of θ.

The graph of y = sin θ, for 0˚ ≤ θ ≤ 360˚ obtained is as shown:

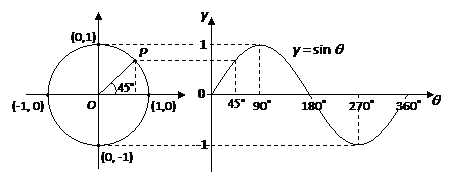
Properties of the sine function:

• The sine function forms a wave that starts from the origin
• sin** θ**= 0 when** θ** = 0˚, 180˚, 360˚.
• Maximum value of sin** θ** is 1 when **θ**= 90˚. Minimum value of sin** θ** is –1 when **θ** = 270˚. So, the range of values of sin **θ** is –1 ≤ sin** θ** ≤ 1
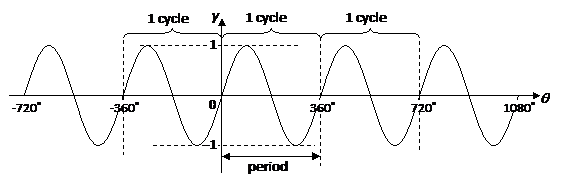
• As the point P moves round the circle in either the clockwise or anticlockwise direction, the sine curve above repeats itself for every interval of 360˚. The interval over which the sine wave repeats itself is called the period.

Example:
The diagram shows a graph of  y = sin x for 0˚ ≤ x  ≤ 360˚, determine the values of p, q and r.

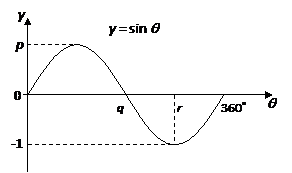
Solution:
In order to answer this type of questions, you will need to remember the general properties (or shape) of a sine graph.

We know that the maximum value of a sine graph is 1. So, p = 1.
We know that for a sine graph, sin θ = 0 for θ= 0˚, 180˚ and 360˚. So,** θ**= 180˚
We know that for a sine graph, sin** θ** = –1 for** θ**= 270˚. So, r = 270˚

Example:
Sketch the graph of y = sin 2x for 0˚ ≤ 2x ≤ 360˚.

Solution:
Set up a table of values for the equation y = sin 2x

x	0	45	90	135	180
2x	0	90	180	270	360
sin 2x	0	1	0	–1	0

Plot the points and join with a smooth curve.

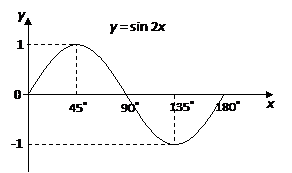
Graphing basic sine and cosine functions (in degrees)

• Show Video Lesson

Graphing basic sine and cosine functions (in radians)
How to graph sine and cosine from the unit circle and from a table of values
Graph the Sine and Cosine functions on the coordinate plane using the unit circle.
Determine the domain and range of the Sine and Cosine function.
Determine the period of the Sine and Cosine Function.
Determine the period of the Sine and Cosine functions.

Sine Graph
• The graph is continuous over its domain (-∞, ∞)
• The period is 2π.
• The x-intercepts occur at multiples of π • sin(-θ) = -sin(θ)

Cosine Graph
• The graph is continuous over its domain (-∞, ∞)
• The period is 2π.
• The x-intercepts occur at multiples of (2n + 1)(π/2)
• cos(-θ) = -cos(θ)

• Show Video Lesson

Amplitude and Period of Sine and Cosine
How to determine the amplitude and period of sine and cosine?
Graphs of functions with different amplitudes and periods.

Periodic Function
A function f is said to be periodic if f(x + P) = f(x) for all values of x.
The constant P is called the period, and is required to be positive. A function with period P will repeat on intervals of length P, and these intervals are sometimes also referred as periods. Period is the horizontal distance before the values begin to repeat.

In general, the graph or y = sin(Bx) or y = cos(Bx) will have period
360°/B = 2π/B
Changing B results in what is called a horizontal dilation or horizontal stretch or compression.

Example:
Determine the period of each function.

1. y = cos(3x)
2. y = sin(x/4)

The amplitude of a periodic function measures its height. The amplitude is defined as the farthest distance the wave reaches from the center of the wave.
Amplitude is also half the vertical distance from the maximum and minimum values.

Example:
Determine the amplitude and period of each function. Then graph the function.

1. y = sin(x/3)
2. y = 1/2 cos(2x)
3. y = -2sin(3x)

• Show Video Lesson

How to use the unit circle definition of the sine function to make a graph of it? (in radians)

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.