Singular Matrix
These lessons help Algebra students to learn what a singular matrix is and how to tell whether a matrix is singular.
Related Pages
Types Of Matrices
More On Singular Matrices
More Lessons On Matrices
If the determinant of a matrix is 0 then the matrix has no inverse. Such a matrix is called a singular matrix.
The following diagrams show how to determine if a 2×2 matrix is singular and if a 3×3 matrix is singular. Scroll down the page for examples and solutions.
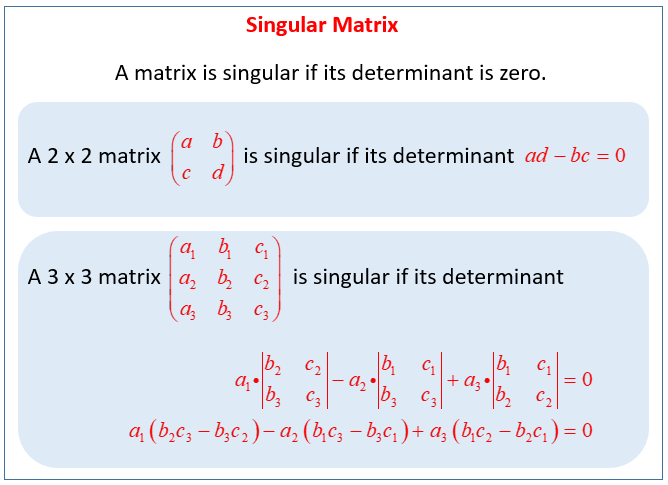
Example:
![]()
Solution:
Determinant = (3 × 2) – (6 × 1) = 0
The given matrix does not have an inverse. It is a singular matrix.
When A Matrix Cannot Be Inverted And The Reasons Why It Cannot Be Inverted?
How to know if a matrix is invertible?
How to know if a matrix is singular?
What Is A Singular Matrix And How To Tell If A 2x2 Matrix Is Singular?
A singular matrix is one which is non-invertible i.e. there is no multiplicative inverse, B, such that the original matrix A × B = I (Identity matrix)
A matrix is singular if and only if its determinant is zero.
Example: Are the following matrices singular?
Determine A Value In A 2×2 Matrix To Make The Matrix Singular
A square matrix A is singular if it does not have an inverse matrix.
Matrix A is invertible (non-singular) if det(A) = 0, so A is singular if det(A) = 0
Example: Determine the value of b that makes matrix A singular.
Singular Matrices Examples
- Determine whether or not there is a unique solution.
- For what value of x is A a singular matrix.
Determine A Value In A 3x3 Matrix To Make The Matrix Singular
Example: Determine the value of a that makes matrix A singular.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.