Slope of a Line Worksheets

Printable “Linear Equations” worksheets:
Linear Equations & Coordinate Plane
Slope of a Line (from 2 points)
Slope of a Line (from equation)
Graph Equation using Intercepts
Find the Equation of a Line from a Graph
Find the Equation of a Line from 2 Points
Constant Rate & Linear Equations

Examples, solutions, videos, and worksheets to help Grade 7 and Grade 8 learn how to use the slope formula to compute the slope of a non-vertical line.

How to use the slope formula to compute the slope of a non-vertical line?

The slope of a line can be calculated using any two points on the same line because the slope triangles formed are similar, and corresponding sides will be equal in ratio.

Slope is referred to as the difference in y-values compared to the difference in x-values, or as the height compared to the base of the slope triangle, or as rise over run.

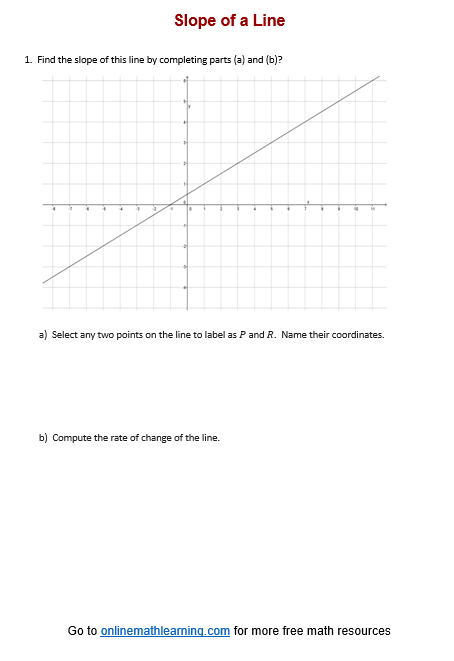
The slope of a non-vertical line in a coordinate plane that passes through two different points is the number given by the difference in y-coordinates of those points divided by the difference in the corresponding x-coordinates. For two points P(p₁,p₂) and R(r₁,r₂) on the line where p₁ ≠ r₁, the slope of the line m can be computed by the formula

m = (p₂ - r₂)/(p₁ - r₁)

The slope of a vertical line is not defined.

Have a look at this video if you need to review how to find the slope of a line.

• How to find the slope of a line

Click on the following worksheet to get a printable pdf document.
Scroll down the page for more Slope of a Line Worksheets.

 

More Slope of a Line Worksheets

Printable
(Answers on the second page.)
Slope of a Line Worksheet #1
Slope of a Line Worksheet #2
Slope of a Line Worksheet #3
Slope of a Line Worksheet #4
Slope of a Line Worksheet #5
Slope of a Line Worksheet #6
Slope of a Line Worksheet #7
Slope of a Line Worksheet #8

Related Lessons & Worksheets

Slope of a Line
More Printable Worksheets

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.