Illustrative Mathematics Grade 7, Unit 6, Lesson 10: Different Options for Solving One Equation

Related Pages
Illustrative Math
Grade 7

Lesson 10: Different Options for Solving One Equation

Let’s think about which way is easier when we solve equations with parentheses.

Illustrative Math Unit 7.6, Lesson 10 (printable worksheets)

Lesson 10 Summary

The following diagram explains how to think about which way is easier when we solve equations with parentheses.

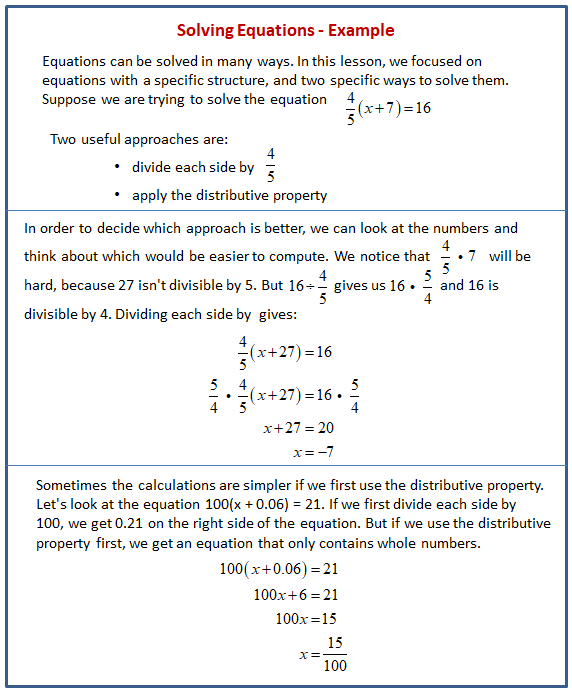
Lesson 10.1 Algebra Talk: Solve Each Equation

100(x - 3) = 1,000
500(x - 3) = 5,000
0.03(x - 3) = 0.3
0.72(x + 2) = 7.2
1/7 (x + 2) = 10/7

• See Video for Whole Lesson

Lesson 10.2 Analyzing Solution Methods

Three students each attempted to solve the equation 2(x - 9) = 10, but got different solutions. Here are their methods. Do you agree with any of their methods, and why?
Noah’s method:
2(x - 9) = 10
2(x - 9) + 9 = 10 + 9
2x = 19
2x ÷2 = 19 ÷ 2
x = 19/2
Elena’s method:
2(x - 9) = 10
2x - 18 = 10
2x - 18 - 18 = 10 - 18
2x = -8
2x ÷2 = 8 ÷ 2
x = -4
Andre’s method:
2(x - 9) = 10
2x - 18 = 10
2x - 18 + 18 = 10 + 18
2x = 28
2x ÷2 = 28 ÷ 2
x = 14

Lesson 10.3 Solution Pathways

For each equation, try to solve the equation using each method (dividing each side first, or applying the distributive property first). Some equations are easier to solve by one method than the other. When that is the case, stop doing the harder method and write down the reason you stopped.

1. 2,000(x - 0.03) = 6,000
2. 2(x + 1.25) = 3.5
3. 1/4 (4 + x) = 4/3
4. -10(x - 1.7) = .3
5. 5.4 = 0.3(x + 8)

Lesson 10 Practice Problems

1. Andre wants to buy a backpack. The normal price of the backpack is $40. He notices that a store that sells the backpack is having a 30% off sale. What is the sale price of the backpack?
2. On the first math exam, 16 students received an A grade. On the second math exam, 12 students received an A grade. What percentage decrease is that?
3. Solve each equation.
   a. 2(x - 3) =14
   b. -5(x - 1) = 40
   c. 12(x + 10) = 24
   d. 1/6(x + 6) = 11
   e. 5/7 (x - 9) = 25
   
4. Select all expressions that represent a correct solution to the equation 6(x + 4) = 20.
   A. (20 - 4) ÷ 6
   B. 1/6 (20 - 4)
   C. 20 - 6 - 4
   D. 20 ÷ 6 - 4
   E. 1/6 (20 - 24)
   F. (20 - 24) ÷ 6
   
5. Lin and Noah are solving the equation 7(x + 2) = 91.
   Lin starts by using the distributive property. Noah starts by dividing each side by 7.
   a. Show what Lin’s and Noah’s full solution methods might look like.
   b. What is the same and what is different about their methods?

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.