

Solve Equations (Grade 8)
Related Topics:
Common Core for Grade 8
Common Core for Mathematics
More Math Lessons for Grade 8
Examples, solutions, videos and lessons to help Grade 8 students learn how to solve linear equations in one variable.
A. Give examples of linear equations in one variable with one solution, infinitely many solutions, or no solutions. Show which of these possibilities is the case by successively transforming the given equation into simpler forms, until an equivalent equation of the form x = a, a = a, or a = b results (where a and b are different numbers).
B. Solve linear equations with rational number coefficients, including equations whose solutions require expanding expressions using the distributive property and collecting like terms.
Common Core: 8.EE.7b
Solving Multi-Step Equations with Rational Numbers
Examples:
On MOnday, David rides his bicycles m miles in 2 hours. On Tuesday, he rides three times as far in 5 hours. If his average speed for two days is 12 mi/h, how far did he ride on the second day? Round your answer to the nearest tenth of a mile. Solving equations with rational numbers (Common Core Standard 8.EE.7b)
Two Step Equations in the Real World Solving Equations with Variables on Both Sides


Common Core for Grade 8
Common Core for Mathematics
More Math Lessons for Grade 8
Examples, solutions, videos and lessons to help Grade 8 students learn how to solve linear equations in one variable.
A. Give examples of linear equations in one variable with one solution, infinitely many solutions, or no solutions. Show which of these possibilities is the case by successively transforming the given equation into simpler forms, until an equivalent equation of the form x = a, a = a, or a = b results (where a and b are different numbers).
B. Solve linear equations with rational number coefficients, including equations whose solutions require expanding expressions using the distributive property and collecting like terms.
Common Core: 8.EE.7b
Suggested Learning Targets
- I can solve linear equations with rational number coefficients.
- I can solve equations whose solutions require expanding expressions using the distributive property and/or collecting like terms.
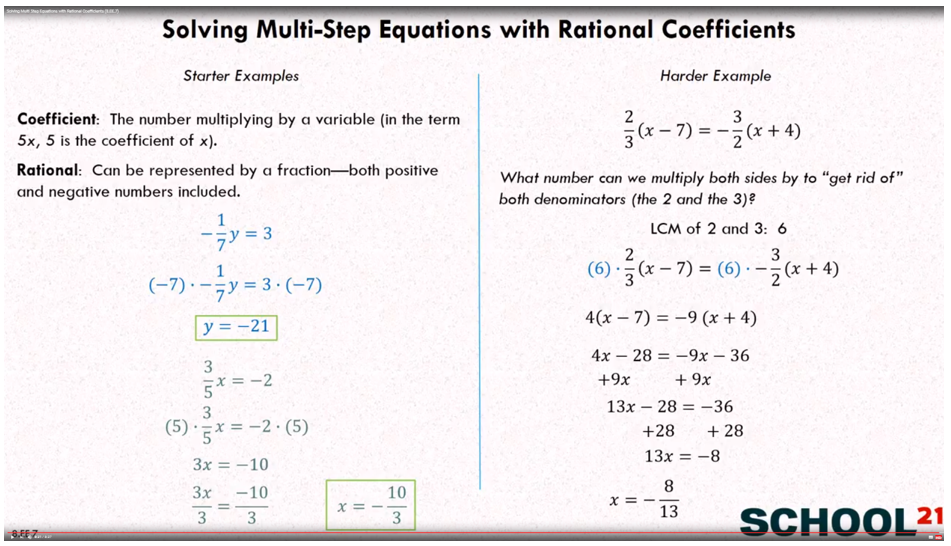
Solve linear equations with rational coefficients (Common Core Standard 8.EE.7b)
Solving Multi Step Equations with Rational Coefficients (8.EE.7)
Coefficient: The number multiplying by a variable.
Rational: Can be represented by a fraction - both positive and negative numbers included.
Examples:
On MOnday, David rides his bicycles m miles in 2 hours. On Tuesday, he rides three times as far in 5 hours. If his average speed for two days is 12 mi/h, how far did he ride on the second day? Round your answer to the nearest tenth of a mile. Solving equations with rational numbers (Common Core Standard 8.EE.7b)
Two Step Equations in the Real World Solving Equations with Variables on Both Sides
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.



We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.