Illustrative Mathematics Grade 7, Unit 6, Lesson 15: Efficiently Solving Inequalities

Related Pages
Illustrative Math
Grade 7

Lesson 15: Efficiently Solving Inequalities

Let’s solve more complicated inequalities.

Illustrative Math Unit 7.6, Lesson 15 (printable worksheets)

Lesson 15 Summary

The following diagram shows how to graph the solutions to an inequality on a number line. and can solve inequalities by solving a related equation and then checking which values are solutions to the original inequality.

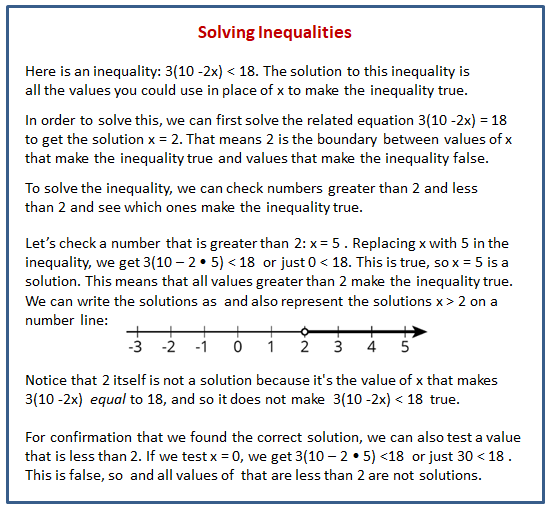
Lesson 15.1 Lots of Negatives

Here is an inequality: -x ≥ -4.

1. Predict what you think the solutions on the number line will look like.
2. Select all the values that are solutions to -x ≥ -4:
   a. 3
   b. -3
   c. 4
   d. -4
   e. 4.001
   f. -4.001
   
3. Graph the solutions to the inequality on the number line:

• See Video for Whole Lesson

Lesson 15.2 Inequalities with Tables

1. Let’s investigate the inequality x - 3 > -2.
   a. Complete the table.
   b. For which values of x is it true that x - 3 = -2?
   c. For which values of x is it true that x - 3 > -2?
   d. Graph the solutions to x - 3 > -2 on the number line:
   
2. Here is an inequality: 2x < 6.
   a. Predict which values of x will make the inequality 2x < 6 true.
   b. Complete the table. Does it match your prediction?
   c. Graph the solutions to 2x < 6 on the number line:
   
3. Here is an inequality: -2x < 6.
   a. Predict which values of x will make the inequality -2x < 6 true.
   b. Complete the table. Does it match your prediction?
   c. Graph the solutions to -2x < 6 on the number line:
   d. How are the solutions to 2x < 6 different from the solutions to -2x < 6?

Lesson 15.3 Which Side are the Solutions?

1. Let’s investigate -4x + 5 ≥ 25.
   a. Solve -4x + 5 = 25.
   b. Is -4x + 5 ≥ 25 true when x is 0? What about when x is 7? What about when x is -7?
   c. Graph the solutions to -4x + 5 ≥ 25 on the number line
   
2. Let’s investigate 4/3 x + 3 < 23/3.
   a. Solve 4/3 x + 3 = 23/3.
   b. Is 4/3 x + 3 < 23/3 true when is 0?
   c. Graph the solutions to 4/3 x + 3 < 23/3 on the number line.
   
3. Solve the inequality 3(x + 4) > 17.4 and graph the solutions on the number line.
   
4. Solve the inequality -3(x - 4/3) ≤ 6 and graph the solutions on the number line

Are you ready for more?

Write at least three different inequalities whose solution is x > -10. Find one with x on the left side that uses a <.

Lesson 15 Practice Problems

1. a. Consider the inequality -1 ≤ x/2.
   i. Predict which values of will make the inequality true.
   Complete the table to check your prediction
   ii. How much sugar was originally in each cake recipe?
   b. Consider the inequality 1 ≤ -x/2.
   i. Predict which values of x will make it true.
   ii. Complete the table to check your prediction.
   
2. Diego is solving the inequality 100 - 3x ≥ -50. He solves the equation 100 - 3x = 50 and gets x = 50. What is the solution to the inequality?
   A. x < 50
   B. x ≤ 50
   C. x > 50
   D. x ≥ 50
   
3. Solve the inequality -5(x - 1) > -40, and graph the solution on a number line.
4. Select all values of x that make the inequality -x + 6 ≥ 10 true.
   A. -3.9
   B. 4
   C. -4.01
   D. -4
   E. 4.01
   F. 3.9
   G. 0
   H. -7
   
5. Draw the solution set for each of the following inequalities.
   a. x > 7
   b. x ≥ -4.2
   
6. The price of a pair of earrings is $22 but Priya buys them on sale for $13.20.
   a. By how much was the price discounted?
   b. What was the percentage of the discount?

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.