Illustrative Mathematics Unit 6.4, Lesson 16: Solving Problems Involving Fractions

Related Pages
Illustrative Math
Grade 6

Lesson 16: Solving Problems Involving Fractions

Let’s add, subtract, multiply, and divide fractions.

Illustrative Math Unit 6.4, Lesson 16 (printable worksheets)

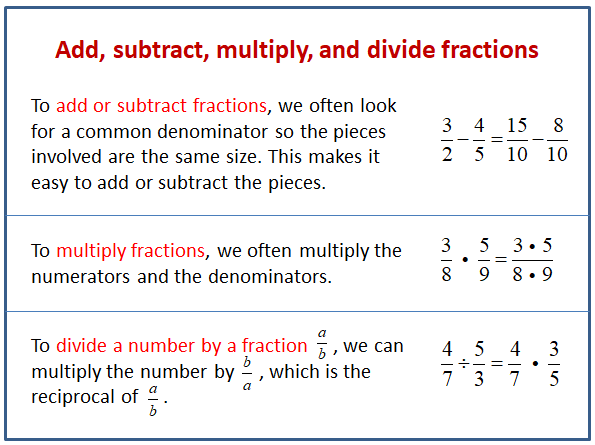
Lesson 16 Summary

The following diagram shows how to add, subtract, multiply, and divide both whole numbers and fractions.

Lesson 16.1 Operations with Fractions

Without calculating, order the expressions according to their values from least to greatest. Be prepared to explain or show your reasoning.
¾ + ⅔
¾ - ⅔
¾ · ⅔
¾ ÷ ⅔

• See Video for Whole Lesson

Lesson 16.2 Situations with ¾ and ½

Here are four situations that involve ¾ and ½.

• Before calculating, decide if each answer is greater than 1 or less than 1.
• Write a multiplication equation or division equation for the situation.
• Answer the question. Show your reasoning. Draw a tape diagram, if needed.
  

1. There was ¾ liter of water in Andre’s water bottle. Andre drank ½ of the water. How many liters of water did he drink?
2. The distance from Han’s house to his school is ¾ kilometer. Han walked ½ kilometer. What fraction of the distance from his house to the school did Han walk?
3. Priya’s goal was to collect ½ kilogram of trash. She collected ¾ kilogram of trash. How many times her goal was the amount of trash she collected?
4. Mai’s class volunteered to clean a park with an area of ½ square mile. Before they took a lunch break, the class had cleaned ¾ of the park. How many square miles had they cleaned before lunch?

Lesson 16.3 Pairs of Problems

1. Work with a partner to write equations for the following questions. One person should work on the questions labeled A1, B1, . . . , E1 and the other should work on those labeled A2, B2, . . . , E2.
   A1. Lin’s bottle holds 3¼ cups of water. She drank 1 cup of water. What fraction of the water in the bottle did she drink?
   B1. Plant A is 16/3 feet tall. This is 4/5 as tall as Plant B. How tall is Plant B?
   C1. 8/9 kilogram of berries is put into a container that already has 7/3 kilogram of berries. How many kilograms are in the container?
   D1. The area of a rectangle is 14½ sq cm and one side is 4½ cm. How long is the other side?
   E1. A stack of magazines is 4⅔ inches high. The stack needs to fit into a box that is 2⅛ inches high. How many inches too high is the stack?
   
   A2. Lin’s bottle holds 3¼ cups of water. After she drank some, there were 1½ cups of water in the bottle. How many cups did she drink?
   B2. Plant A is 16/3 feet tall. Plant C is 4/5 as tall as Plant A. How tall is Plant C?
   C2. A container with 8/9 kilogram of berries is 2/3 full. How many kilograms can the container hold?
   D2. The side lengths of a rectangle are 4½ cm and 2⅖ cm. What is the area of the rectangle?
   E2. A stack of magazines is 4⅖ inches high. Each magazine is ⅖-inch thick. How many magazines are in the stack?
   
2. Trade papers with your partner, and check your partner’s equations. If there is a disagreement about what an equation should be, discuss it until you reach an agreement.
3. Your teacher will assign 2–3 questions for you to answer. For each question:
   a. Estimate the answer before calculating it.
   b. Find the answer, and show your reasoning.

Lesson 16.4 Baking Cookies

Mai, Kiran, and Clare are baking cookies together. They need ¾ cup of flour and ½ cup of butter to make a batch of cookies. They each brought the ingredients they had at home.

• Mai brought 2 cups of flour and ¼ cup of butter.
• Kiran brought 1 cup of flour and ½ cup of butter.
• Clare brought 1¼ cups of flour and ¾ cup of butter.

If the students have plenty of the other ingredients they need (sugar, salt, baking soda, etc.), how many whole batches of cookies can they make? Explain your reasoning.

Lesson 16 Practice Problems

1. An orange has about ¼ cup of juice. How many oranges are needed to make 2½ cups of juice? Select all equations that represent this question.
   A. ? · ¼ = 2½
   B. ¼ ÷ 2½ = ?
   C. ? ÷ 2½ = ¼
   D. 2½ ÷ ¼ = ?
   
2. Mai, Clare, and Tyler are hiking from a parking lot to the summit of a mountain. They pass a sign that gives distances.

• Parking lot: ¾ mile
• Summit: 1½ miles

Mai says: “We are one third of the way there.” Clare says: “We have to go twice as far as we have already gone.” Tyler says: “The total hike is three times as long as what we have already gone.”
Can they all be correct? Explain how you know.
3. Priya’s cat weighs 5½ pounds and her dog weighs 8¼ pounds. Estimate the missing number in each statement before calculating the answer. Then, compare your answer to the estimate and explain any discrepancy.
The cat is _______ as heavy as the dog.
Their combined weight is _______ pounds.
The dog is _______ pounds heavier than the cat.
4. Before refrigerators existed, some people had blocks of ice delivered to their homes. A delivery wagon had a storage box in the shape of a rectangular prism that was feet by 6 feet by 6 feet. The cubic ice blocks stored in the box had side lengths feet. How many ice blocks fit in the storage box?
A. 270
B. 3⅜
C. 80
D. 180
5. Fill in the blanks with 0.001, 0.1, 10, or 1000 so that the value of each quotient is in the correct column.
close to 1/100

• ____ ÷ 9
  
• 12 ÷ ____
  
  close to 1
  
• ____ ÷ 0.12
  
• ⅛ ÷ ____
  
  greater than 100
  
• ____ ÷ ⅓
  
• 700.7 ÷____
  

6. A school club sold 300 shirts. 31% were sold to fifth graders, 52% were sold to sixth graders, and the rest were sold to teachers. How many shirts were sold to each group—fifth graders, sixth graders, and teachers? Explain or show your reasoning.
7. Jada has some pennies and dimes. The ratio of Jada’s pennies to dimes is 2 to 3.
   a. From the information given above, can you determine how many coins Jada has?
   b. If Jada has 55 coins, how many of each kind of coin does she have?
   c. How much are her coins worth?
   
   
   
   

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.