Solve Quadratic Equation by Factoring Worksheets

Printable “Quadratic Equations” worksheets:
Solve Quadratic Equation (use factoring)
Solve Word Problems using Quadratic Equations
Sketching Quadratic Graphs

Rewrite Expressions in Completed-Squared Form
Solve Quadratic Equation (use completing the square)
Solve Quadratic Equation (use quadratic formula)
Discriminant in the Quadratic Formula

Examples, solutions, videos, and worksheets to help Grade 7 and Grade 8 students learn how to solve quadratic equations by factoring.

How to solve quadratic equations using factoring?

There are four sets of solving equations using factoring worksheets.

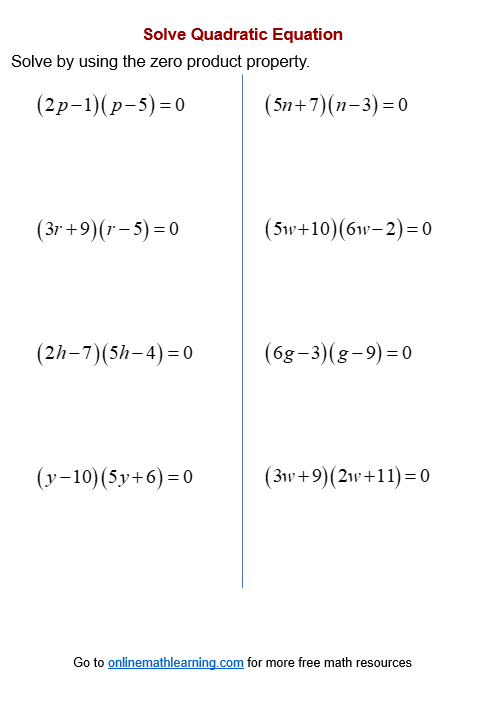
• Solve Quadratic Equation by Factoring (use zero product property).
• Solve Quadratic Equation by Factoring (factor & solve, a = 1).
• Solve Quadratic Equation by Factoring (factor & solve, a ≠ 1).
• Solve Quadratic Equation by Factoring (rearrange, factor & solve).

These are the steps to solve a quadratic equation by factoring:

1. Write the quadratic equation in the form: ax²+bx+c=0, where a, b, and c are constants.
2. Factor the quadratic expression. Look for two binomials whose product gives you the original quadratic expression.
3. Set each of the binomial factors equal to zero. This will give you two separate linear equations to solve.
4. Solve each linear equation for x. These solutions are the roots or solutions of the quadratic equation.
5. Check if the solutions obtained in step 4 satisfy the original quadratic equation.

Example: Solve the quadratic equation x² − 5x = -6.

1. Rewrite down the equation as: x² − 5x + 6 = 0.
2. Factor the quadratic expression:
   x² − 5x + 6 = 0
   (x − 2)(x − 3) = 0
   
3. Set each factor equal to zero:
   x − 2 = 0 or
   x − 3 = 0
   
4. Solve for x For
   x − 2 = 0
   x = 2
   For
   x − 3 = 0
   x = 3
   
5. Check solutions: Substitute x = 2 and x = 3 back into the original equation.
   2² − 5(2) + 6 = 0 (True)
   3² − 5(3) + 6 = 0 (True)

Remember that not all quadratic equations can be easily factored. Some may require more complex factoring methods, or you may need to use the quadratic formula or completing the square to find the solutions.

Have a look at this video if you need to review how to solve quadratics equations by factoring.

• Solve Quadratic Equation by Factoring

Click on the following worksheet to get a printable pdf document.
Scroll down the page for more Solve Quadratic Equation by Factoring Worksheets.

 

More Solve Quadratic Equation by Factoring Worksheets

Printable
(Answers on the second page.)
Solve Quadratic Equation by Factoring Worksheet #1 (use zero product property)
Solve Quadratic Equation by Factoring Worksheet #2 (factor & solve, a = 1)
Solve Quadratic Equation by Factoring Worksheet #3 (factor & solve, a ≠ 1)
Solve Quadratic Equation by Factoring Worksheet #4 (rearrange, factor & solve)
Solve Quadratic Equation by Factoring Worksheet #5 (mixed)
Solve Quadratic Equation by Factoring Worksheet #6 (mixed)
Solve Quadratic Equation by Factoring Worksheet #7 (mixed)
Solve Quadratic Equation by Factoring Worksheet #8 (use square root)
Solve Quadratic Equation by Factoring Worksheet #9 (use square root)
Solve Quadratic Equation by Factoring Worksheet #10 (use square root)

Online or Generated
Factor Binomials by Difference of Squares
Factor Perfect Square Trinomials

Factor Trinomials or Quadratic Equations
Factor Different Types of Trinomials 1
Factor Different Types of Trinomials 2

Solve Trinomials using Quadratic Formula
Find Discriminants of Quadratic Polynomials

Related Lessons & Worksheets

Solve Quadratic Equation by Factoring (a > 1)
Solve Quadratic Equation by Factoring (common factors)

More Printable Worksheets

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.