

Solve Rational Equations
Common Core: HSA-REI.A.2
Related Topics:
Algebra Word Problems
Common Core (Algebra)
Common Core for Mathematics
Examples, solutions, videos and lessons to help High School students learn how to solve simple rational and radical equations in one variable, and give examples showing how extraneous solutions may arise.
Examples:
Solve and eliminate any extraneous solutions:
x2/(x + 2) = 4/(x + 2)
Solve a Rational Equation with Extraneous Solution
Solve a rational equation by multiplying both sides by the LCD and check the answers for extraneous solutions.
Solve 4(x - 3)/(x2 - 36) = 1/(6 - x) + 2x/(6 + x)
Solving Rational Equations
This video explains how to solve rational equations.
Examples:
1. 2/3 - 5/6 = 1/t
2. (x + 2)/(x - 6) = (x - 1)/(x + 2)
3. x/(x + 3) - x/(x - 2) = 10/(x2 + x - 6)
4. 4y/(y + 2) - 3y/(y - 1) = (y2 - 8y - 4)/(y2 + y - 2) Ex 1: Solving Rational Equations.
Examples:
1. 2x/15 - 1/6 = x/5
2. 5/(x - 3) = 4/(x + 2)
Ex 2: Solving Rational Equations.
Examples:
1. 2/5x - 3 = 4/x
2. 2x - 16/x = 4
Ex 3: Solving Rational Equations.
Examples:
1. (x + 4)/x = 6/(x - 4)
2. 5/(x - 7) = -2x/(x + 3)
Ex 4: Solving Rational Equations.
Examples:
1. x/(x - 2) - x/(x2 - 4) = (x + 3)/(x + 2)
2. 5/(x - 3) = 4/(x + 2)
Ex 5: Solving Rational Equations.
Examples:
1. (x - 3)/(x + 6) + (x - 2)/(x - 3) = x2/(x2 + 3x - 18)
2. 5/(x - 3) = 4/(x + 2)
Applications of Rational Equations I
The video explains application problems that use rational equations. Part 1 of 2. Applications of Rational Equations II
The video explains application problems that use rational equations. Part 2 of 2.


Related Topics:
Algebra Word Problems
Common Core (Algebra)
Common Core for Mathematics
Examples, solutions, videos and lessons to help High School students learn how to solve simple rational and radical equations in one variable, and give examples showing how extraneous solutions may arise.
Suggested Learning Targets
- Solve simple rational and radical equations in one variable and provide examples of how extraneous solutions arise.
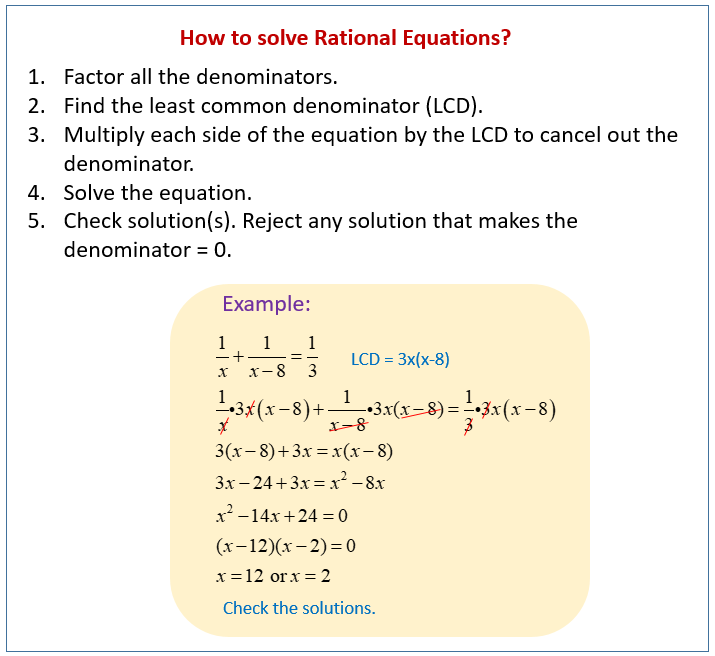
Examples:
Solve and eliminate any extraneous solutions:
x2/(x + 2) = 4/(x + 2)
Solve a Rational Equation with Extraneous Solution
Solve a rational equation by multiplying both sides by the LCD and check the answers for extraneous solutions.
Solve 4(x - 3)/(x2 - 36) = 1/(6 - x) + 2x/(6 + x)
This video explains how to solve rational equations.
Examples:
1. 2/3 - 5/6 = 1/t
2. (x + 2)/(x - 6) = (x - 1)/(x + 2)
3. x/(x + 3) - x/(x - 2) = 10/(x2 + x - 6)
4. 4y/(y + 2) - 3y/(y - 1) = (y2 - 8y - 4)/(y2 + y - 2) Ex 1: Solving Rational Equations.
Examples:
1. 2x/15 - 1/6 = x/5
2. 5/(x - 3) = 4/(x + 2)
Ex 2: Solving Rational Equations.
Examples:
1. 2/5x - 3 = 4/x
2. 2x - 16/x = 4
Ex 3: Solving Rational Equations.
Examples:
1. (x + 4)/x = 6/(x - 4)
2. 5/(x - 7) = -2x/(x + 3)
Ex 4: Solving Rational Equations.
Examples:
1. x/(x - 2) - x/(x2 - 4) = (x + 3)/(x + 2)
2. 5/(x - 3) = 4/(x + 2)
Ex 5: Solving Rational Equations.
Examples:
1. (x - 3)/(x + 6) + (x - 2)/(x - 3) = x2/(x2 + 3x - 18)
2. 5/(x - 3) = 4/(x + 2)
Applications of Rational Equations I
The video explains application problems that use rational equations. Part 1 of 2. Applications of Rational Equations II
The video explains application problems that use rational equations. Part 2 of 2.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.



We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.