

Solving Two Step Equations - Integers
Related Topics:
Algebra Worksheets
Algebra Games
This is part of a series of free, online Basic Algebra Lessons or Algebra I lessons.
We also have videos, worksheets, and activities to help Algebra students.
This video explains how to solve basic two step equations.
Examples:
1. 2x + 3 = 9
2. 5x - 8 = 22
3. 9 - 2x = 23
4. -11 = 4x - 43
5. -15 = 5 - 5x
Ex 1: Solving Two-Step Equations Using Integers
This video provides 4 examples of how to solve two step equations by adding, subtracting, multiplying and dividing integers.
Examples:
4x + 7 = -21
-2x - 9 = -21
x/3 + 5 = -4
-x/2 - 9 = -2
Ex 2: Solving Two-Step Equations Using Integers
This video provides 2 more examples of how to solve two step equations by adding, subtracting, multiplying and dividing integers.
Examples:
12 - 3x = -3
5 = x/4 + 17
Solving Two-Step Linear Equations
Here we look at solving linear equations where we have to perform addition / subtraction as well as division / multiplication to solve.
Example:
Solve the following:
-4x - 14 = 10
Solving Two-Step Linear Equations Example:
Solve the following:
9x - 4 = 77
-4 + n/5 = -8 Solving Two-Step Equations
Five examples of solving two-step equations.
Examples:
b/3 + 13 = 11
9g + 11 = 2
t/4 - 10 = -6
-a + 6 = 8
15x + 3 = 48


Algebra Worksheets
Algebra Games
This is part of a series of free, online Basic Algebra Lessons or Algebra I lessons.
We also have videos, worksheets, and activities to help Algebra students.
In these lessons, we will learn how to
- solve basic two-step equations using integers.
- solve two-step equations using integers.
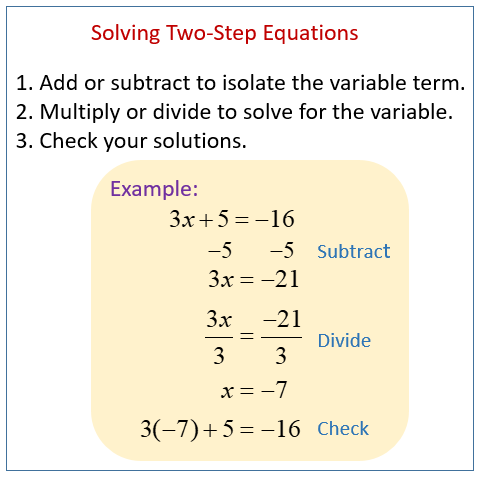
Solving Two Step Equations
Equations can be tricky, and solving two step equations is an important step beyond solving equations in one step. Solving two-step equations will help introduce students to solving equations in multiple steps, a skill necessary in Algebra I and II. To solve these types of equations, we use additive and multiplicative inverses to isolate and solve for the variable. Solving Two Step Equations: The BasicsThis video explains how to solve basic two step equations.
Examples:
1. 2x + 3 = 9
2. 5x - 8 = 22
3. 9 - 2x = 23
4. -11 = 4x - 43
5. -15 = 5 - 5x
This video provides 4 examples of how to solve two step equations by adding, subtracting, multiplying and dividing integers.
Examples:
4x + 7 = -21
-2x - 9 = -21
x/3 + 5 = -4
-x/2 - 9 = -2
Ex 2: Solving Two-Step Equations Using Integers
This video provides 2 more examples of how to solve two step equations by adding, subtracting, multiplying and dividing integers.
Examples:
12 - 3x = -3
5 = x/4 + 17
Solving Two-Step Linear Equations
Here we look at solving linear equations where we have to perform addition / subtraction as well as division / multiplication to solve.
Example:
Solve the following:
-4x - 14 = 10
Solving Two-Step Linear Equations Example:
Solve the following:
9x - 4 = 77
-4 + n/5 = -8 Solving Two-Step Equations
Five examples of solving two-step equations.
Examples:
b/3 + 13 = 11
9g + 11 = 2
t/4 - 10 = -6
-a + 6 = 8
15x + 3 = 48
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.



We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.