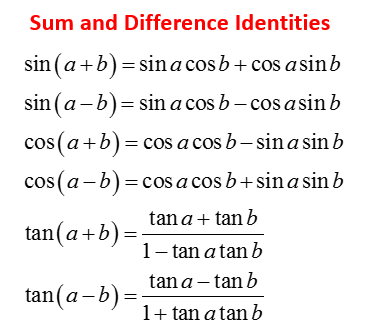
Sum And Difference Identities

What are the Sum and Difference Identities?

Sum and difference identities are a set of trigonometric formulas that allow you to express trigonometric functions of the sum or difference of two angles in terms of trigonometric functions of those individual angles. These identities are incredibly useful for simplifying expressions, solving equations, and finding exact values of trigonometric functions.

The following shows the Sum and Difference Identities for sin, cos and tan. Scroll down the page for more examples and solutions on how to use the identities.

Example:

Solution:
Given that cos(α + β) = cos α cos β – sin α sin β, then

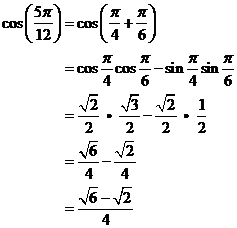
Example:

Solution:

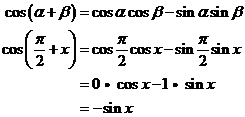
How to use the sum and difference identities for sin, cos, and tan?
Example:

1. Find sin(105°) exactly
2. Find cos(105°) exactly
3. Find tan(105°) exactly

• Show Video Lesson

How to use Sum and Difference Identities to find exact trig values?
Example:

1. Find $\cos \left( {\frac{{3\pi }}{4},\frac{\pi }{3}} \right)$ exactly
2. Find cos(42°)cos(18°) - sin(42°)sin(18°) exactly
3. Find $\frac{{\tan 80^\circ - \tan 35^\circ }}{{1 + \tan 80^\circ \tan 35^\circ }}$ exactly
4. Find cos(u + v) exactly if sin(u) = 3/5 and sin(v) = 12/13 where u and v are acute angles (quadrant I)

• Show Video Lesson

How to use the Sum and Difference Identities to Prove Other Identities
Example:
Prove sin(α + β) - sin(α - β) = 2cosαsinβ

• Show Video Lesson

Using the Sum and Difference Identities for Sine, Cosine and Tangent
Example 1:
If sin x = 12/13 and x is in the first quadrant, find tan(2x)

• Show Video Lesson

Using the Sum and Difference Identities for Sine, Cosine and Tangent
Example 2:
If tan x = 5/3 and x is in the first quadrant, find sin(2x)

• Show Video Lesson

Using the Sum and Difference Identities for Sine, Cosine and Tangent
Example 3:
Simplify 1 - 16sin²x cos²x

• Show Video Lesson

Key Applications

1. Finding Exact Values:
   These identities can be used to find the exact values of trigonometric functions for angles that are not directly on the unit circle. For example, you can find sin(75°) by expressing it as sin(45° + 30°).
2. Simplifying Expressions:
   They can help simplify complex trigonometric expressions, making them easier to work with.
3. Solving Trigonometric Equations:
   These identities are essential tools for solving various trigonometric equations.

Important Notes:

• It’s crucial to remember the signs in these formulas, as they can easily lead to errors.
• These identities are valid for any angles A and B.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.