

Solving Systems of Equations Using Elimination By Addition
Related Topics:
More Lessons for Algebra
Math Worksheets
Examples, solutions, videos, worksheets, games, and activities to help Algebra 1 students learn how to solve systems of linear equations by addition or elimination.
The following diagram shows an example of solving systems of equations by elimination by addition. Scroll down the page for more examples and solutions of solving systems of equations.
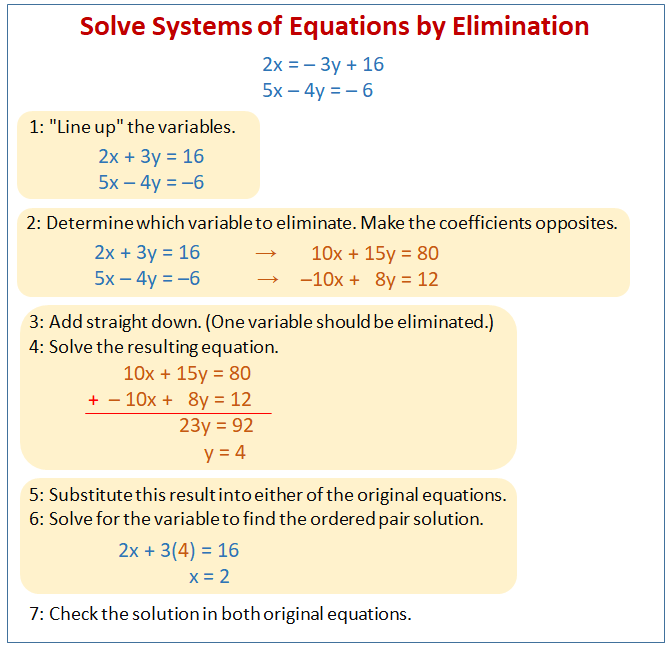
Solving Systems of Equations... Elimination Method
How to use the elimination method to solve a system of linear equations (simultaneous equations)?
Example 1: where terms cancel right away when you add the equations.
Example 2: Multiply one equation by a number before adding the equations
Example 3: Multiply both equations by numbers before adding.
Example 4: How to subtract equations instead of adding them.
How to Solve Systems of Equations Using Elimination By Addition?
1. Line up the variables.
2. Determine which variables to eliminate. Make the coefficients opposites.
3. Add straight down. (One variable should be eliminated).
4. Solve the resulting equation.
5. Substitute the result into either of the original equation.
6. Solve for the variable to find the ordered pair solution.
7. Check the solution to both original equations.
Solving Systems of Equations
Students learn to solve a system of linear equations by addition
To solve a system of equations by addition, the goal is to cancel out one of the variables by adding the equations together. Students may need to multiply one or both of the equations by a number in order to set up a situation where one of the variables will cancel out when the equations are added together. Solving a system of two equations in two variables by the addition method Solving Systems of Linear Equations Using Elimination By Addition
Two complete examples and part of a third problem are shown! Solve Systems of Linear Equations by Elimination


More Lessons for Algebra
Math Worksheets
Examples, solutions, videos, worksheets, games, and activities to help Algebra 1 students learn how to solve systems of linear equations by addition or elimination.
The following diagram shows an example of solving systems of equations by elimination by addition. Scroll down the page for more examples and solutions of solving systems of equations.

Solving Systems of Equations... Elimination Method
How to use the elimination method to solve a system of linear equations (simultaneous equations)?
Example 1: where terms cancel right away when you add the equations.
Example 2: Multiply one equation by a number before adding the equations
Example 3: Multiply both equations by numbers before adding.
Example 4: How to subtract equations instead of adding them.
1. Line up the variables.
2. Determine which variables to eliminate. Make the coefficients opposites.
3. Add straight down. (One variable should be eliminated).
4. Solve the resulting equation.
5. Substitute the result into either of the original equation.
6. Solve for the variable to find the ordered pair solution.
7. Check the solution to both original equations.
Solving Systems of Equations
Students learn to solve a system of linear equations by addition
To solve a system of equations by addition, the goal is to cancel out one of the variables by adding the equations together. Students may need to multiply one or both of the equations by a number in order to set up a situation where one of the variables will cancel out when the equations are added together. Solving a system of two equations in two variables by the addition method Solving Systems of Linear Equations Using Elimination By Addition
Two complete examples and part of a third problem are shown! Solve Systems of Linear Equations by Elimination
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.



We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.