Theorems involving Trapezoids
In these lessons, we will learn how to use theorems involving trapezoids in High School Math as required for the Regents Exam math conducted by NYSED.
Related Pages
More Lessons for High School Regents Exam
Math Worksheets
The following diagram shows some theorems for rectangles, rhombuses, and squares. Scroll down the page for more examples and solutions on postulates and theorems for rectangles, rhombuses, and squares.
Trapezoid and Kite Theorems & Corollaries
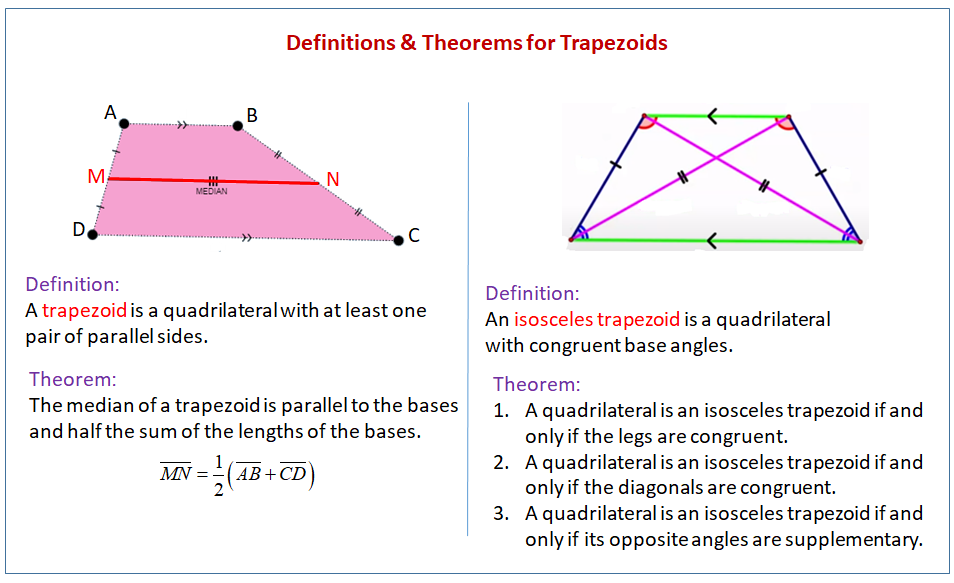
Theorems:
-
Midsegment Theorem for Trapezoids
The midsegment of a trapezoid is parallel to each base and its length is one half the sum of the lengths of the bases (average of the bases) -
If a trapezoid is isosceles, then each pair of base angles is congruent.
-
If a trapezoid has a pair of congruent base angles, then it is an isosceles trapezoid.
-
A trapezoid is isosceles if and only if its diagonals are congruent.
-
If a quadrilateral is a kite, then its diagonals are perpendicular.
-
If a quadrilateral is a kite, then exactly one pair of opposite angles are congruent.
Isosceles Trapezoid Theorem
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.