Illustrative Mathematics Unit 6.1, Lesson 1: Tiling the Plane
Learn about tiling the plane and reasoning to find the area of regular and irregular shapes. After trying the questions, click on the buttons to view answers and explanations in text or video.
Related Pages
Math Worksheets
Return to the list of Illustrative Math lessons
Tiling the Plane
Let’s look at tiling patterns and think about area.
Illustrative Math Unit 6.1, Lesson 1 (printable worksheets)
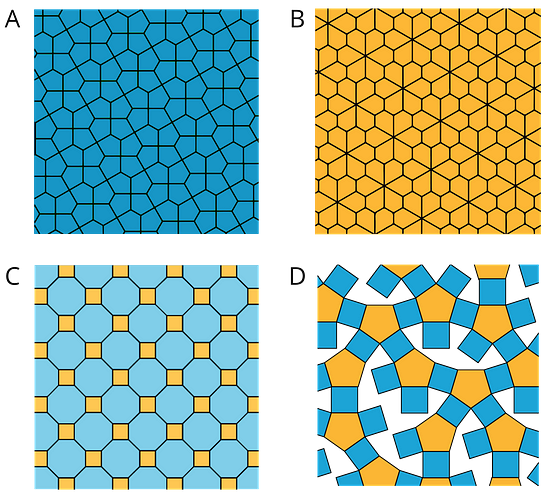
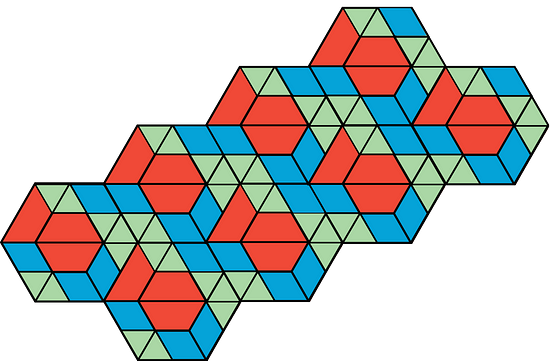
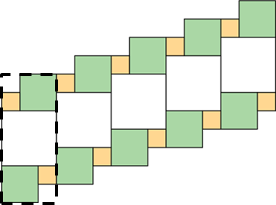
1.1 - Which One Doesn’t Belong: Tilings
Which pattern doesn’t belong? Give reasons.
- What is Tiling the Plane?
- See Possible Answers
- See Video 1 for Whole Lesson
- See Video 2 for Whole Lesson
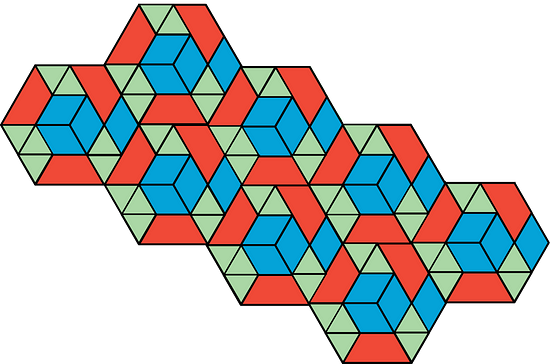
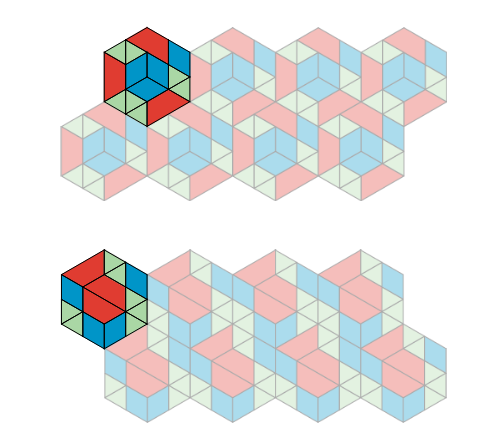
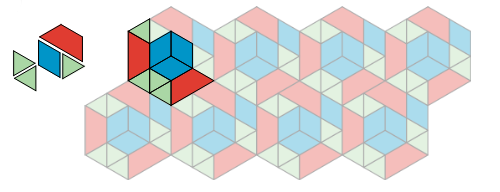
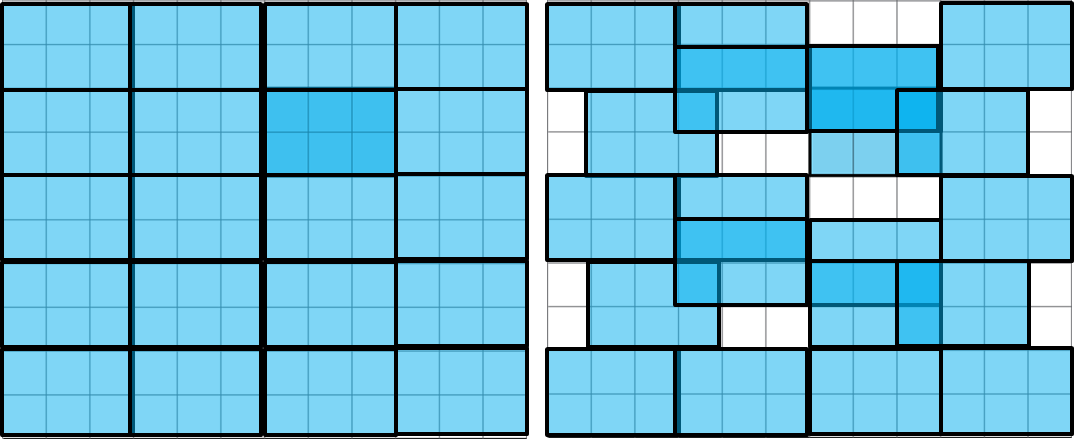
1.2 - More Red, Green, or Blue?
Look at Pattern A or B.
In your pattern, which shapes cover more of the plane: blue rhombuses, red trapezoids, or green triangles? Explain how you know.
You may use the applet here to help.
Lesson 1 Summary
In this lesson, we learned about tiling the plane, which means covering a two-dimensional region with copies of the same shape or shapes such that there are no gaps or overlaps.
Then, we compared tiling patterns and the shapes in them. In thinking about which patterns and shapes cover more of the plane, we have started to reason about area.
We will continue this work, and to learn how to use mathematical tools strategically.
Practice Problems
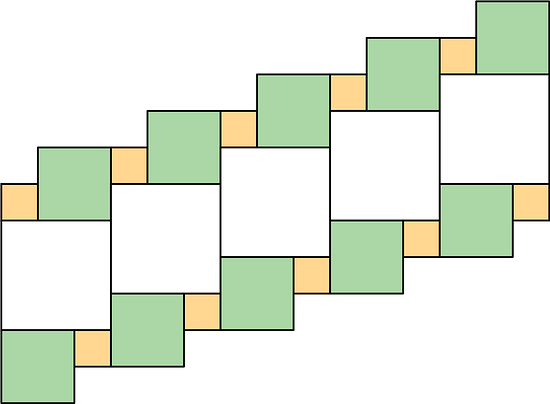
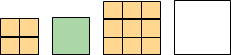
- Which square—large, medium, or small—covers more of the plane? Explain your reasoning.
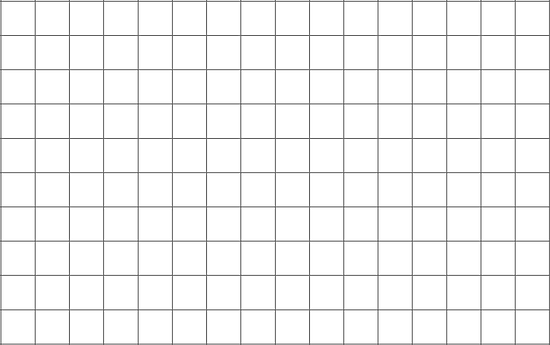
- Draw three different quadrilaterals, each with an area of 12 square units. (The grid is 10 units tall and 16 units wide.)
- Use copies of the rectangle to show how a rectangle could:
a. tile the plane.
b. not tile the plane.
- The area of this shape is 24 square units. Which of the statements below is true about the area? Select all that apply.
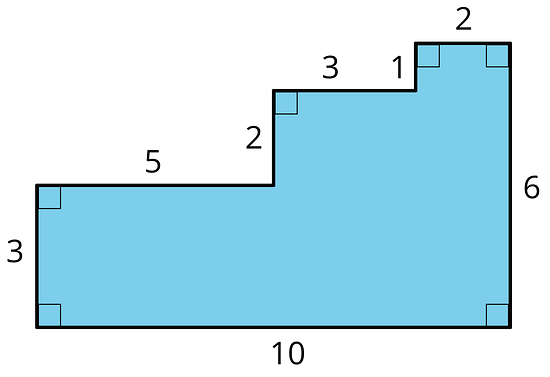
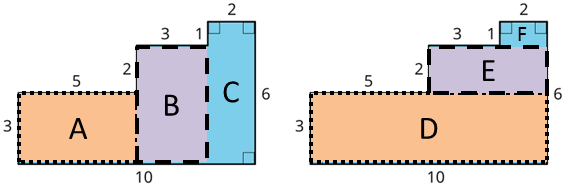
- Show two different ways for finding the area of the shaded region. All angles are right angles.
- Which shape has a larger area: a rectangle that is 7 inches by ¾ inch, or a square with a side length of 2½ inches? Show your reasoning.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.
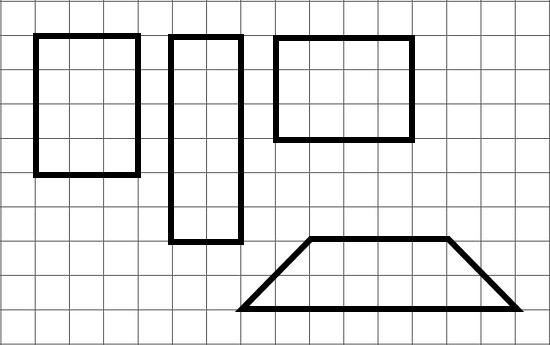
 A: The area can be found by counting the number of squares that touch the edge of the shape.
A: The area can be found by counting the number of squares that touch the edge of the shape.