Distance Word Problems - Given the Total Time

Distance problems are word problems that involve the distance an object will travel at a certain average rate for a given period of time. These problems can also involve an object traveling to a destination and returning. The goal is to find the total distance, time, or average speed.

The formula for distance problems is: distance = rate × time or d = r × t.

It would be helpful to use a table to organize the information for distance problems.

The following diagram shows how to set up a Rate-Time-Distance (RTD) table to help solve time-distance problems. Scroll down the page for more examples and solutions on how to solve distance problems.

 

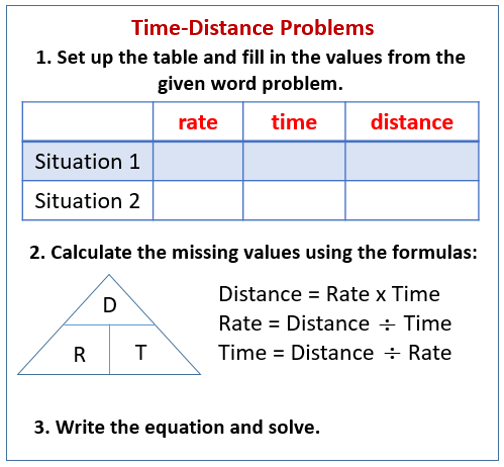
Key Points for Round Trip Problems:

• Distance is the same: The distance to and from the destination is equal.
• Time is different: The times for each leg of the trip are usually different because the speeds are different.
• Average speed is not the average of speeds: Calculate total distance and total time to find average speed. It’s a common mistake to simply average the two speeds.
• Be careful with units: Make sure your units (miles, hours, km, etc.) are consistent.

The following diagram gives the steps to solve a round-trip distance problem.

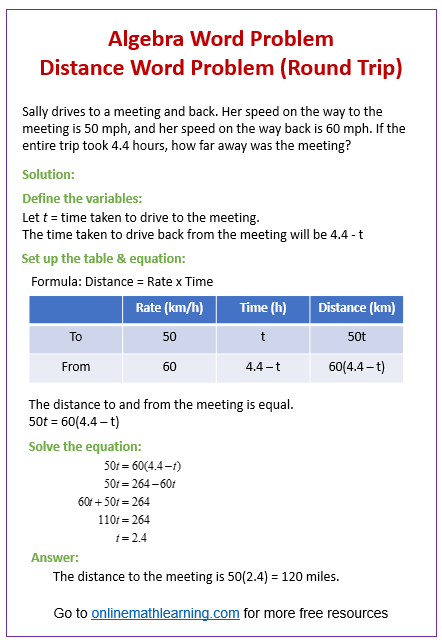
Types of Distance Word Problems:
Travel in Same Direction
Travel in Opposite Directions
Round Trip Problems
Average Speed Problems
Wind/Current Problems

Printable & Online Algebra Worksheets

Distance Problems: Given Total Time

Example:
John took a drive to town at an average rate of 40 mph. In the evening, he drove back at 30 mph. If he spent a total of 7 hours traveling, what is the distance traveled by John?

Solution:
Step 1: Set up a rtd table.

	r	t	d
Case 1
Case 2

Step 2: Fill in the table with information given in the question.

John took a drive to town at an average rate of 40 mph. In the evening, he drove back at 30 mph. If he spent a total of 7 hours traveling, what is the distance traveled by John?

Let t = time to travel to town.

7 – t = time to return from town.

	r	t	d
Case 1	40	t
Case 2	30	7 – t

Step 3: Fill in the values for d using the formula d = rt

	r	t	d
Case 1	40	t	40t
Case 2	30	7 – t	30(7 – t)

Step 4: Since the distances traveled in both cases are the same, we get the equation:

40t = 30(7 – t)
Use distributive property
40t = 210 – 30t
Isolate variable t
40t + 30t = 210
70t = 210


Step 5: The distance traveled by John to town is

40t = 120
The distance traveled by John to go back is also 120
So, the total distance traveled by John is 240

Answer: The distance traveled by John is 240 miles.

Distance, Rate, Time Word Problems
Two examples of distance, rate, and time. One involves adding the distances in our chart, where as the other example involves setting the distances equal to each other.

1. Two truck drivers leave a cafe at the same time, traveling in opposite directions. On truck goes 7 mph faster than the other one. After 4 hr, they are 404 miles apart. How fast is each truck going?
2. Ryan left the science museum and drove south at a rate of 28 km/h. Jenna left three hours later driving 42 km/h faster in an effort to catch up to him. How long did Jenna have to travel to catch up with Ryan?

• Show Video Lesson

Distance-time word problem where the total time is given

Example:
Gordon rode his bike at 15 mph to go get his car. He then drove back at 45 mph. If the entire trip took him 8 hours, how far was his car?

• Show Video Lesson

Check out many other Algebra Word Problems
Age Word Problems, Average Word Problems, Coin Word Problems, Consecutive Integer Word Problems, Digit Word Problems, Distance Word Problems, Fraction Word Problems, Geometry Word Problems, Integer Word Problems, Interest Word Problems, Lever Word Problems, Mixture Word Problems, Money Word Problems, Motion & Distance Word Problems, Number Sequence Word Problems, Proportion Word Problems, Quadratic Equation Word Problems, Ratio Word Problems, Symbol Word Problems, Variation Word Problems, Work Word Problems.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.