Transitive, Reflexive and Symmetric Properties of Equality
Related Pages
More Lessons for Grade 6 Math
Math Worksheets
Examples, solutions, videos, worksheets, stories, and songs to help Grade 6 students learn about the transitive, reflexive and symmetric properties of equality.
The properties of equality are fundamental rules that allow us to manipulate equations while maintaining equality. These properties are used extensively in algebra to solve for unknown variables.
The following diagram gives the properties of equality: reflexive, symmetric, transitive, addition, subtraction, multiplication, division, and substitution. Scroll down the page for more examples and solutions on equality properties.
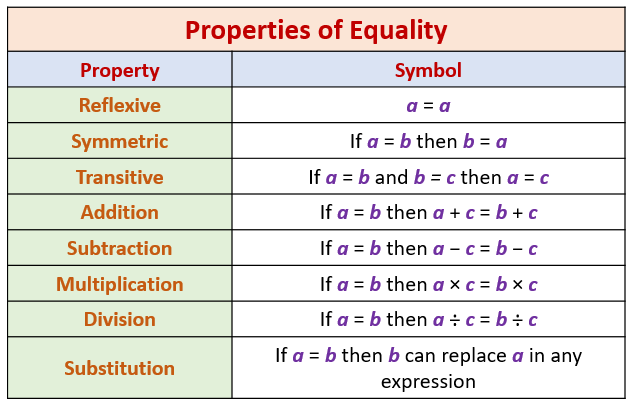
- Reflexive Property:
For any real number a, a = a.
A quantity is equal to itself. - Symmetric Property:
For any real numbers a and b, if a = b, then b = a.
The order in which an equality is stated does not matter. - Transitive Property:
For any real numbers a, b, and c, if a = b and b = c, then a = c.
If two quantities are equal to the same quantity, then they are equal to each other. - Addition Property of Equality:
For any real numbers a, b, and c, if a = b, then a + c = b + c.
You can add the same quantity to both sides of an equation. - Subtraction Property of Equality:
For any real numbers a, b, and c, if a = b, then a - c = b - c.
You can subtract the same quantity from both sides of an equation. - Multiplication Property of Equality:
For any real numbers a, b, and c, if a = b, then a * c = b * c.
You can multiply both sides of an equation by the same quantity. - Division Property of Equality:
For any real numbers a, b, and c, if a = b and c ≠ 0, then a / c = b / c.
You can divide both sides of an equation by the same non-zero quantity. - Substitution Property of Equality:
If a = b, then b can be substituted for a in any expression.
If two quantities are equal, one can be replaced by the other in any expression without changing the value of the expression.
Explanations on the Properties of Equality
Examples of Addition, Subtraction, Multiplication, and Division Properties of Equality
Transitive Property of Equality - Math Help
Students learn the following properties of equality: reflexive, symmetric, addition, subtraction, multiplication, division, substitution, and transitive.
Algebra1 2.01c - The Transitive Property
Reflexive Property and Symmetric Property
Students learn the following properties of equality: reflexive, symmetric, addition, subtraction, multiplication, division, substitution, and transitive.
Try out our new and fun Fraction Concoction Game.
Add and subtract fractions to make exciting fraction concoctions following a recipe. There are four levels of difficulty: Easy, medium, hard and insane. Practice the basics of fraction addition and subtraction or challenge yourself with the insane level.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.