Volume Of Sphere
In these lessons, we will learn
- how to find the volume of a sphere.
- how to find the volume of a hemisphere.
- how to prove the formula for the volume of a sphere.
Related Pages
Volume Formulas
Explanations For The Surface Area Formulas
Volume Of Cylinders
More GCSE Maths Lessons
More Geometry Lessons
Spheres
What is a sphere?
A sphere is a solid with all its points the same distance from the center. The distance is known as the radius of the sphere. The maximum straight distance through the center of a sphere is known as the diameter of the sphere. The diameter is twice the radius.
The following figure gives the formula for the volume of sphere. Scroll down the page for examples and solutions.
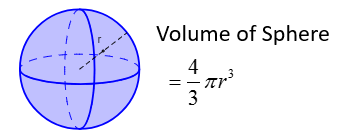
How to find the volume of a sphere?
The volume of a sphere is equal to four-thirds of the product of pi and the cube of the radius.
The volume and surface area of a sphere are given by the formulas:

where r is the radius of the sphere.
Volume of spheres (Worksheets)
Surface area of spheres (Worksheets)
Example:
Calculate the volume of sphere with radius 4 cm.
Solution:
Volume of sphere

We can also change the subject of the formula to obtain the radius given the volume.
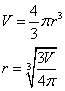
Example:
The volume of a spherical ball is 5,000 cm3. What is the radius of the ball?
Solution:
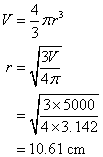
How to use the formula to calculate the volume of a sphere?
Example:
Find the volume of a sphere with a diameter of 14 cm.
Volume of a hemisphere
What is a hemisphere?
A hemisphere is half a sphere, with one flat circular face and one bowl-shaped face.

How to find the volume of a hemisphere?
The volume of a hemisphere is equal to two-thirds of the product of pi and the cube of the radius.
The volume of a hemisphere is given by the formula:
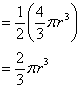
where r is the radius.
How to find the volume of a hemisphere?
Example:
Find the volume of a fishbowl with a diameter of 33cm.
How to solve word problems about spheres?
The following video shows how to solve problems involving the formulas for the surface area
and volume of spheres.
Example:
A sphere has a volume of 288π. Find its area. Leave you answer in terms of π
Example:
A ball has a diameter of 18 cm.
a) Sketch a cylinder that fits the ball and label its height and base.
b) What is the volume of the cylinder?
c) What is the volume of the ball?
Example:
The cylinder is melted down into a sphere of radius r. Find an expression for r in terms of x.
How to proof the Formula of the Volume of a Sphere?
This video gives a proof for the formula of the volume of a sphere that does not involve calculus.
This is an "approximated" proof. You would need to use calculus for a more rigorous proof.
How to derive the formula of a sphere using calculus?
How Archimedes derived the volume of a sphere?
To do so, he had to use a formula for the volume of a cone.
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.